Von Neumann ergodic theorem

For any isometric operator on a Hilbert space and for any the limit

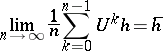exists (in the sense of convergence in the norm of ). For a continuous one-parameter group of unitary transformations on and any , the limit

exists (in the same sense). Here is the orthogonal projection of 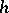onto the space of - (or 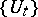-) invariant elements of .

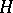J. von Neumann stated and proved this theorem in [1], having in mind in the first instance its application in ergodic theory, when in a measure space an endomorphism is given (or a measurable flow ), when and where is the shift operator:

In this case von Neumann's theorem states that the time average of , that is, the mean value of 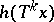, or , on the time interval , or , when this interval is lengthened, converges to 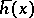in mean square with respect to (which is often emphasized by the term mean ergodic theorem). In particular, for a sufficiently long interval the averaged time mean of for the majority of is close to 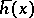. Therefore, von Neumann's theorem (and its generalizations) is frequently (especially when applied to a given case) called the statistical ergodic theorem, in contrast to the individual ergodic theorem, that is, the Birkhoff ergodic theorem (and its generalizations). From the latter (and for 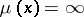, from arguments used in its proof) one can in this case deduce von Neumann's ergodic theorem. 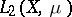However, in general, when is not realized as and the operator or is not connected with any transformation in 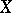, von Neumann's theorem does not follow from Birkhoff's.

Von Neumann's original proof was based on the spectral decomposition of unitary operators. Later a number of other proofs were published (the simplest is due to F. Riesz, see [2]) and it was generalized to wider classes of groups and semi-groups of operators on Banach spaces (see [3], [4]).

Von Neumann's theorem, and its generalizations, is an operator ergodic theorem.

References

[1]	J. von Neumann, "Proof of the quasi-ergodic hypothesis" Proc. Nat. Acad. Sci. USA , 18 (1932) pp. 70–82
[2]	P.R. Halmos, "Lectures on ergodic theory" , Math. Soc. Japan (1956)
[3]	A.M. Vershik, S.A. Yuzvinskii, "Dynamical systems with an invariant measure" Progress in Math. , 8 (1970) pp. 151–215 Itogi Nauk. Mat. Anal. 1967 (1969) pp. 133–187
[4]	A.B. Katok, Ya.G. Sinai, A.M. Stepin, "Theory of dynamical systems and general transformation groups with invariant measure" J. Soviet Math. , 7 : 6 (1977) pp. 964–1065 Itogi Nauk. i Tekhn. Mat. Anal. , 13 (1975) pp. 129–262

Comments

For a wider variety of ergodic theorems see [a1].

References

[a1]	U. Krengel, "Ergodic theorems" , de Gruyter (1985)