Poincaré-Hopf theorem
From Encyclopedia of Mathematics
Revision as of 18:53, 24 March 2012 by Ulf Rehmann (talk | contribs) (moved Poincaré–Hopf theorem to Poincare-Hopf theorem: ascii title)
Let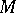 
be a smooth compact manifold with boundary 
, and let 
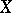be a vector field on 
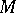with isolated zeros such that 
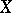points outwards at all points in the boundary 
.
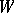Then the sum of the indices of the zeros of 
(see Singular point, index of a) is equal to the Euler characteristic of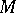 
.
This is the generalization proved by H. Hopf, in 1926, of the two-dimensional version owed to H. Poincaré (1881, 1885) (see Poincaré theorem).
References
| [a1] | J.W. Milnor, "Topology from the differentiable viewpoint" , Univ. Virginia Press (1965) pp. 35 |
| [a2] | N.G. Lloyd, "Degree theory" , Cambridge Univ. Press (1978) pp. 33 |
How to Cite This Entry:
Poincaré-Hopf theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Poincar%C3%A9-Hopf_theorem&oldid=23478
Poincaré-Hopf theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Poincar%C3%A9-Hopf_theorem&oldid=23478
This article was adapted from an original article by M. Hazewinkel (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article