Calderón-Zygmund operator
An operator  defined on a space of sufficiently smooth functions
defined on a space of sufficiently smooth functions  with compact support in
with compact support in  by the formula
by the formula
 |
where the kernel  is a homogeneous function of degree
is a homogeneous function of degree  with zero mean value over the unit sphere
with zero mean value over the unit sphere  . The kernel
. The kernel  has the form
has the form
 |
where  , the characteristic function of
, the characteristic function of 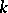 , satisfies the conditions
, satisfies the conditions
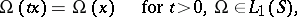 | (*) |
 |
The Calderón–Zygmund operator is usually written in the form
 |
here 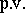 denotes the principal value of the integral. In the one-dimensional case the Calderón–Zygmund operator becomes the Hilbert operator
denotes the principal value of the integral. In the one-dimensional case the Calderón–Zygmund operator becomes the Hilbert operator 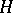 :
:
 |
The Calderón–Zygmund operator can be extended by continuity to the space  of functions
of functions  in
in 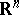 that are summable of degree
that are summable of degree 
 . This extension maps
. This extension maps  continuously into itself. If
continuously into itself. If  satisfies the condition (*) and also Dini's condition:
satisfies the condition (*) and also Dini's condition:
 |
and if
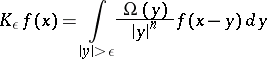 |
for  and
and  , then
, then
a) there exists a constant  (independent of
(independent of 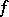 or
or  ) such that
) such that
 |
b) the limit  exists in the sense of convergence in
exists in the sense of convergence in  and
and
 |
The Calderón–Zygmund operator was analyzed by A.P. Calderón and A. Zygmund [1].
References
| [1] | A.P. Calderón, A. Zygmund, "On the existence of certain singular integrals" Acta Math. , 88 (1952) pp. 85–139 |
| [2] | S.G. Mikhlin, "Multidimensional singular integrals and integral equations" , Pergamon (1965) (Translated from Russian) |
| [3] | E.M. Stein, "Singular integrals and differentiability properties of functions" , Princeton Univ. Press (1970) |
Comments
Proofs of the estimates a) and b) above can be found in [3], Chapt. II, Section 4.
In the 1960's the operators described above were often called Mikhlin–Calderón–Zygmund operators, since the main boundedness theorem for them on 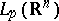 (estimate a)) was proved by S.G. Mikhlin in 1938 (published in [a1]).
(estimate a)) was proved by S.G. Mikhlin in 1938 (published in [a1]).
Nowadays the term Calderón–Zygmund operator is often used for general operators in the class defined by G. David and J.L. Journé in [a2].
Assertion b) has been generalized to singular integrals with odd kernels, and to singular integrals with even kernels  , [a3], Chapt. VI, Sections 2, 3.
, [a3], Chapt. VI, Sections 2, 3.
See also Singular integral; Hilbert singular integral; Hilbert transform.
References
| [a1] | S.G. Mikhlin, "On a boundedness theorem for a singular integral operator" Uspekhi Mat. Nauk , 8 (1953) pp. 213–217 (In Russian) |
| [a2] | G. David, J.L. Journé, "Une characterization des opérateurs intégraux singuliers bornés sur 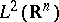 " C.R. Acad. Sci. Paris , 296 (1983) pp. 761–764 " C.R. Acad. Sci. Paris , 296 (1983) pp. 761–764 |
| [a3] | E.M. Stein, G. Weiss, "Fourier analysis on Euclidean spaces" , Princeton Univ. Press (1975) |
Calderón-Zygmund operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Calder%C3%B3n-Zygmund_operator&oldid=23210