Cayley-Klein parameters
Special coordinates in the rotation group  of three-dimensional space, the construction of which is based in the final analysis on the relationship between
of three-dimensional space, the construction of which is based in the final analysis on the relationship between 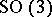 and the group
and the group  of
of  unitary matrices with determinant 1. There exists a mapping
unitary matrices with determinant 1. There exists a mapping  which is an epimorphism by virtue of its algebraic properties and a double covering by virtue of its topological properties. (Restricted to some neighbourhood of the identity matrix,
which is an epimorphism by virtue of its algebraic properties and a double covering by virtue of its topological properties. (Restricted to some neighbourhood of the identity matrix,  is an isomorphism; in other words,
is an isomorphism; in other words,  and
and  are locally isomorphic.) Each matrix
are locally isomorphic.) Each matrix  may be written as
may be written as
 |
where  are complex numbers such that
are complex numbers such that 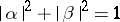 . These are taken to be the Cayley–Klein parameters of
. These are taken to be the Cayley–Klein parameters of 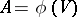 . (The term is sometimes used for all four elements of the matrix
. (The term is sometimes used for all four elements of the matrix  .) As the actual construction of a mapping
.) As the actual construction of a mapping 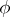 with the above properties may be accomplished in various ways, different authors define the Cayley–Klein parameters in slightly different ways (see [2], [3]).
with the above properties may be accomplished in various ways, different authors define the Cayley–Klein parameters in slightly different ways (see [2], [3]).
Since  is not a true isomorphism, but only a double covering, it is impossible to define the Cayley–Klein parameters as (continuous) coordinates on all of
is not a true isomorphism, but only a double covering, it is impossible to define the Cayley–Klein parameters as (continuous) coordinates on all of  ; this can be done only locally. However, the Cayley–Klein parameters may nevertheless be used to study processes of rotation in which
; this can be done only locally. However, the Cayley–Klein parameters may nevertheless be used to study processes of rotation in which  is a continuous function of a real parameter
is a continuous function of a real parameter  (and there is no necessity to restrict the domain of possible values of
(and there is no necessity to restrict the domain of possible values of  in any way). Indeed, if some fixed value
in any way). Indeed, if some fixed value  has been chosen at
has been chosen at  , the corresponding values of
, the corresponding values of  are uniquely defined by continuity for all
are uniquely defined by continuity for all  . (The fact that the complete inverse
. (The fact that the complete inverse  is double-valued intrudes only in the observation that
is double-valued intrudes only in the observation that  not only when
not only when  but also when
but also when 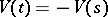 .) Thus the Cayley–Klein parameters can be applied to investigate the motion of a rigid body with a fixed point (the configuration space of which is
.) Thus the Cayley–Klein parameters can be applied to investigate the motion of a rigid body with a fixed point (the configuration space of which is  ). This approach was adopted in [1], but did not achieve popularity.
). This approach was adopted in [1], but did not achieve popularity.
The group  is isomorphic to the group of quaternions with norm 1 (cf. Quaternion); hence, by going over from
is isomorphic to the group of quaternions with norm 1 (cf. Quaternion); hence, by going over from 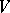 to the corresponding quaternion
to the corresponding quaternion  , one can replace the Cayley–Klein parameters by the Euler–Rodriguez parameters — four real numbers
, one can replace the Cayley–Klein parameters by the Euler–Rodriguez parameters — four real numbers  such that
such that  . These stand in a simple relationship to the Cayley–Klein parameters (see [1], [3]) and possess the same "double-valuedness" property (for the history of the problem see [1]). It was essentially in this context that attention was first directed to two-valued representations of the rotation group (see Spinor).
. These stand in a simple relationship to the Cayley–Klein parameters (see [1], [3]) and possess the same "double-valuedness" property (for the history of the problem see [1]). It was essentially in this context that attention was first directed to two-valued representations of the rotation group (see Spinor).
References
| [1] | F. Klein, A. Sommerfeld, "Ueber die Theorie des Kreises" , 1–2 , Teubner (1965) |
| [2] | H. Goldstein, "Classical mechanics" , Addison-Wesley (1953) |
| [3] | J.L. Synge, "Classical dynamics" , Handbuch der Physik , 3/1 , Springer (1960) pp. 1–225 |
Comments
References
| [a1] | P. Du Val, "Homographies, quaternions and rotations" , Clarendon Press (1964) |
Cayley-Klein parameters. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Cayley-Klein_parameters&oldid=22273