Connected component of the identity

identity component, of a group

The largest connected subset of the topological (or algebraic) group that contains the identity element of this group. The component is a closed normal subgroup of ; the cosets with respect to coincide with the connected components of . The quotient group is totally disconnected and Hausdorff, and is the smallest among the normal subgroups 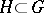such that is totally disconnected. If 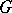is locally connected (for example, if is a Lie group), then is open in and 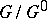is discrete.

In an arbitrary algebraic group the identity component 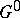is also open and has finite index; also, is the minimal closed subgroup of finite index in 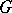. The connected components of an algebraic group coincide with the irreducible components. For every polynomial homomorphism of algebraic groups one has 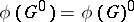. If is defined over a field, then is defined over this field.

If 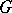is an algebraic group over the field , then its identity component coincides with the identity component of 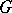considered as a complex Lie group. If is defined over 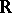, then the group of real points in 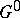is not necessarily connected in the topology of the Lie group 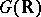, but the number of its connected components is finite. For example, the group splits into two components, although is connected. The pseudo-orthogonal unimodular group 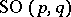, which can be regarded as the group of real points of the connected complex algebraic group 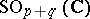, is connected f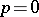or or 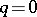, and splits into two components for 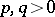. However, if the Lie group 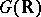is compact, then 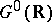is connected.

References

[1]	A. Borel, "Linear algebraic groups" , Benjamin (1969)
[2]	L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1958) (Translated from Russian)
[3]	S. Helgason, "Differential geometry and symmetric spaces" , Acad. Press (1962)
[4]	I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian)