Comitant

concomitant of a group acting on sets and

A mapping 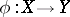such that

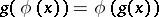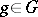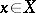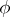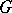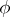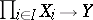for any , . In this case one also says that commutes with the action of , or that is an equivariant mapping. If acts on every set of a family , then a comitant is called a simultaneous comitant of .

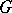The notion of a comitant originates from the classical theory of invariants (cf. Invariants, theory of) in which, however, a comitant is understood in a narrower sense: is the general linear group of some finite-dimensional vector space , 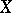and 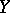are tensor spaces on 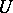of specified (generally distinct) types, on which 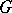acts in the natural way, while 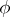is an equivariant polynomial mapping from 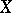into . 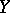If, in addition, is a space of covariant tensors, then the comitant is called a covariant of 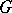, while if 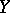is a space of contravariant tensors, the comitant is called a contravariant of .

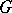Example. Let 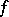be a binary cubic form in the variables and :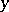

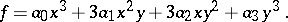Its coefficients are the coordinates of a covariant symmetric tensor. The coefficients of the Hessian form of 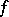, that is, of the form

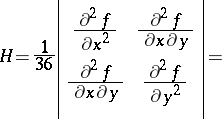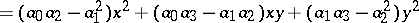are also the coefficients of a covariant symmetric tensor, while the mapping

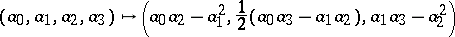of the corresponding tensor spaces is a comitant (the so-called comitant of the form ). The Hessian of an arbitrary form can similarly be defined; this also provides an example of a comitant (see Covariant).

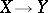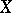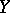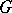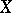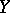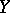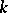In the modern geometric theory of invariants, by a comitant one often means any equivariant morphism , where and are algebraic varieties endowed with a regular action of an algebraic group . If and are affine, then giving a comitant is equivalent to giving a homomorphism of -modules of regular functions on the varieties and , respectively (where is the ground field).

References

[1]	G.B. Gurevich, "Foundations of the theory of algebraic invariants" , Noordhoff (1964) (Translated from Russian)
[2]	D. Mumford, "Geometric invariant theory" , Springer (1965)
[3]	J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1955)