Transvection

A linear mapping 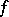of a (right) vector space 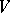over a skew-field 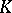with the properties

where is the identity linear transformation. A transvection can be represented in the form

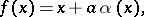where 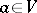, 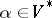and .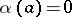

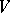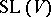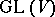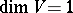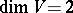The transvections of a vector space generate the special linear, or unimodular, group . It coincides with the commutator subgroup of , with the exception of the cases when or and 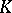is the field of two elements. If is a field, then is the group of matrices with determinant 1. In the general case (provided that ), 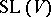is the kernel of the epimorphism

which is called the Dieudonné determinant (cf. Determinant).

References

[1]	J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1955)

Comments

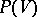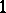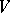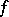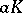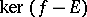In the projective space , whose points are the -dimensional subspaces of , a transvection as above induces a (projective) transvection with as centre and as axis. If one takes to be a hyperplane at infinity in 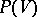, such a transvection induces a translation 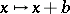in the remaining affine space (interpreted as a linear space). See also Shear.