Measure space

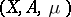
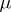A measurable space with a measure given on 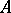(i.e. a countably-additive function 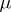with values in for which 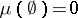; the latter property follows from additivity if the measure is finite, i.e. does not take the value , or even if there is some 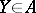with 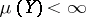). The notation 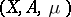is often shortened to 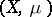and one says that 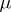is a measure on ; sometimes the notation is shortened to . The basic case is when 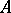is a -algebra (cf. Algebra of sets) and 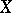can be represented as with and 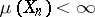. In this case the measure is called (totally) -finite (while if , then it is called (totally) finite). Such is, e.g., the Lebesgue measure on (cf. Lebesgue space). However, sometimes non--finite measures are encountered, such as, e.g., the 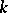-dimensional Hausdorff measure on 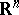for . One may also encounter modifications in which 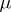takes values in 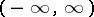, or complex or vector values, as well as cases when 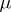is only finitely additive.

References

[1]	P.R. Halmos, "Measure theory" , v. Nostrand (1950)
[2]	N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Interscience (1958)