Pfaffian
From Encyclopedia of Mathematics
of a skew-symmetric matrix 
The polynomial 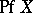 in the entries of
in the entries of 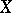 whose square is
whose square is 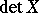 . More precisely, if
. More precisely, if 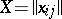 is a skew-symmetric matrix (i.e.
is a skew-symmetric matrix (i.e. 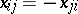 ,
, 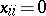 ; such a matrix is sometimes also called an alternating matrix) of order
; such a matrix is sometimes also called an alternating matrix) of order 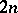 over a commutative-associative ring
over a commutative-associative ring  with a unit, then
with a unit, then 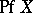 is the element of
is the element of 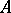 given by the formula
given by the formula
 |
where the summation is over all possible partitions  of the set
of the set 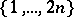 into non-intersecting pairs
into non-intersecting pairs 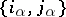 , where one may suppose that
, where one may suppose that 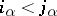 ,
, 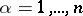 , and where
, and where  is the sign of the permutation
is the sign of the permutation
 |
A Pfaffian has the following properties:
1) 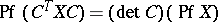 for any matrix
for any matrix 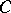 of order
of order 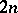 ;
;
2) 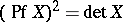 ;
;
3) if 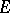 is a free
is a free 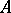 -module with basis
-module with basis  and if
and if
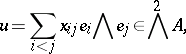 |
then
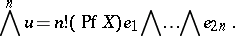 |
References
| [1] | N. Bourbaki, "Elements of mathematics. Algebra: Modules. Rings. Forms" , 2 , Addison-Wesley (1975) pp. Chapt.4;5;6 (Translated from French) |
How to Cite This Entry:
Pfaffian. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pfaffian&oldid=20491
Pfaffian. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Pfaffian&oldid=20491
This article was adapted from an original article by A.L. Onishchik (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article