A-set
analytic set, in a complete separable metric space
A continuous image of a Borel set. Since any Borel set is a continuous image of the set of irrational numbers, an  -set can be defined as a continuous image of the set of irrational numbers. A countable intersection and a countable union of
-set can be defined as a continuous image of the set of irrational numbers. A countable intersection and a countable union of  -sets is an
-sets is an  -set. Any
-set. Any  -set is Lebesgue-measurable. The property of being an
-set is Lebesgue-measurable. The property of being an  -set is invariant relative to Borel-measurable mappings, and to
-set is invariant relative to Borel-measurable mappings, and to  -operations (cf.
-operations (cf.  -operation). Moreover, for a set to be an
-operation). Moreover, for a set to be an  -set it is necessary and sufficient that it can be represented as the result of an
-set it is necessary and sufficient that it can be represented as the result of an  -operation applied to a family of closed sets. There are examples of
-operation applied to a family of closed sets. There are examples of 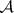 -sets which are not Borel sets; thus, in the space
-sets which are not Borel sets; thus, in the space  of all closed subsets of the unit interval
of all closed subsets of the unit interval  of the real numbers, the set of all closed uncountable sets is an
of the real numbers, the set of all closed uncountable sets is an  -set, but is not Borel. Any uncountable
-set, but is not Borel. Any uncountable  -set topologically contains a perfect Cantor set. Thus,
-set topologically contains a perfect Cantor set. Thus,  -sets "realize" the continuum hypothesis: their cardinality is either finite,
-sets "realize" the continuum hypothesis: their cardinality is either finite,  or
or  . The Luzin separability principles hold for
. The Luzin separability principles hold for  -sets.
-sets.
References
| [1] | K. Kuratowski, "Topology" , 1 , Acad. Press (1966) (Translated from French) |
| [2] | N.N. [N.N. Luzin] Lusin, "Leçons sur les ensembles analytiques et leurs applications" , Gauthier-Villars (1930) |
Comments
Nowadays the class of analytic sets is denoted by 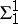 , and the class of co-analytic sets (cf.
, and the class of co-analytic sets (cf.  -set) by
-set) by  .
.
References
| [a1] | T.J. Jech, "The axiom of choice" , North-Holland (1973) |
| [a2] | Y.N. Moschovakis, "Descriptive set theory" , North-Holland (1980) |
A-set. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=A-set&oldid=19565