Kawamata-Viehweg vanishing theorem

Let be a connected complex projective manifold (cf. Projective scheme). Let denote the canonical bundle of , i.e., the determinant bundle of the cotangent bundle (cf. Tangent bundle) of . A line bundle on (cf. also Vector bundle) is said to be nef if the degree of the restriction of to any effective curve on is non-negative. A line bundle is said to be big if the sections of some positive power of give a birational mapping of 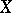into projective space. For a nef line bundle on 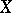, bigness is equivalent to 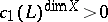, where 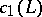denotes the first Chern class of . Let 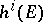be the dimension of the 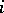th cohomology group of the sheaf of germs of algebraic or analytic sections of an algebraic line bundle on a projective variety. The Kawamata–Viehweg vanishing theorem states that for a nef and big line bundle on a complex projective manifold ,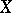

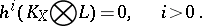When is a complex compact curve of genus , the bigness of a line bundle 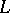is equivalent to the line bundle being ample (cf. also Ample vector bundle), and since 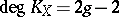, the Kawamata–Viehweg vanishing theorem takes the form if 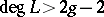; or, equivalently, 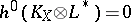if . For 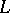with at least one not-identically-zero section, this vanishing theorem is equivalent to the Roch identification [a15], of the number now (1998) denoted by 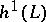with 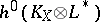, i.e., the one-dimensional Serre duality theorem. In the late 19th century, the numbers intervened in geometric arguments in much the same way as they intervene today, e.g., [a3]. For a very ample line bundle on a two-dimensional complex projective manifold, the Kawamata–Viehweg vanishing theorem was well known as the Picard theorem on the regularity of the adjoint, [a13], Vol. 2; Chap. X111; Sec. IV. This result was based on a description of [a9], Formula I.17, in terms of the double point divisor of a sufficiently general projection of into .

The next large step towards the Kawamata–Viehweg vanishing theorem was due to K. Kodaira [a10]. By means of a curvature technique that S. Bochner [a2] had used to show vanishing of real cohomology groups, Kodaira showed that for an ample line bundle on a compact complex projective manifold, 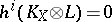for 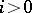. Many generalizations of the Kodaira vanishing theorem appeared. Especially notable are results of C.P. Ramanujan [a14], which include the Kawamata–Viehweg vanishing theorem in the two-dimensional case; see also [a12].

The following formulation [a6], [a7], [a4] of the Kawamata–Viehweg vanishing theorem is better adapted to applications. To state it in its simplest form, additive notation is used and 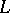is taken to be a line bundle such that 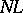, i.e., the 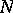-th tensor power of 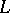, can be written as a sum of a nef and big line bundle plus an effective divisor (cf. Divisor) 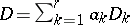, where are positive integers and 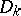are smooth irreducible divisors such that any subset of the divisors meet transversely along their intersection. Then, for ,

where denotes the greatest integer less than or equal to a real number .

For more history and amplifications of these theorems see [a5], [a16]. See [a8] and [a11] for further generalizations of the Kawamata–Viehweg vanishing theorem. The paper [a8] is particularly useful: it contains relative versions of the vanishing theorem with some singularities, for not necessarily Cartier divisors. For applications of the vanishing theorems to classical problems, see [a1].

References

[a1]	M. Beltrametti, A.J. Sommese, "The adjunction theory of complex projective varieties" , Experim. Math. , 16 , W. de Gruyter (1995)
[a2]	S. Bochner, "Curvature and Betti numbers I–II" Ann. of Math. , 49/50 (1948/9) pp. 379–390; 77–93
[a3]	G. Castelnuovo, F. Enriques, "Sur quelques résultat nouveaux dans la théorie des surfaces algébriques" E. Picard (ed.) G. Simart (ed.) , Théorie des Fonctions Algébriques , I–II
[a4]	E. Viehweg, "Vanishing theorems" J. Reine Angew. Math. , 335 (1982) pp. 1–8
[a5]	H. Esnault, E. Viehweg, "Lectures on vanishing theorems" , DMV-Sem. , 20 , Birkhäuser (1992)
[a6]	Y. Kawamata, "On the cohomology of 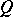-divisors" Proc. Japan Acad. Ser. A , 56 (1980) pp. 34–35
[a7]	Y. Kawamata, "A generalization of Kodaira–Ramanujam's vanishing theorem" Math. Ann. , 261 (1982) pp. 43–46
[a8]	Y. Kawamata, K. Matsuda, K. Matsuki, "Introduction to the minimal model problem" , Algebraic Geometry, Sendai 1985 , Adv. Stud. Pure Math. , 10 (1987) pp. 283–360 MR0946243 Zbl 0672.14006
[a9]	S.L. Kleiman, "The enumerative theory of singularities" P. Holme (ed.) , Real and Complex Singularities, Oslo 1976 , Sijthoff&Noordhoff (1977) pp. 297–396
[a10]	K. Kodaira, "On a differential-geometric method in the theory of analytic stacks" Proc. Nat. Acad. Sci. USA , 39 (1953) pp. 1268–1273
[a11]	J. Kollár, "Higher direct images of dualizing sheaves I–II" Ann. of Math. , 123/4 (1986) pp. 11–42; 171–202
[a12]	Y. Miyaoka, "On the Mumford–Ramanujam vanishing theorem on a surface" , Journees de Geometrie Algebrique, Angers/France 1979 (1980) pp. 239–247
[a13]	Picard, G. Simart, "Théorie des fonctions algébriques I–II" , Chelsea, reprint (1971)
[a14]	C.P. Ramanujam, "Remarks on the Kodaira vanishing theorem" J. Indian Math. Soc. , 36 (1972) pp. 41–51 (See also the Supplement: J. Indian Math. Soc. 38 (1974), 121-124)
[a15]	G. Roch, "Über die Anzahl der willkürlichen Constanten in algebraischen Funktionen" J. de Crelle , 44 (1864) pp. 207–218
[a16]	B. Shiffman, A.J. Sommese, "Vanishing theorems on complex manifolds" , Progr. Math. , 56 , Birkhäuser (1985)