Simple group

A group without normal subgroups different from the unit subgroup and the entire group (cf. Normal subgroup). The description of all finite simple groups is a central problem in the theory of finite groups (cf. Simple finite group). In the theory of infinite groups the significance of simple groups is substantially less, as they are difficult to visualize. 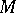The group of all even permutations fixing all but a finite number of elements of a set is simple if has cardinality at least 5. If 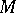is infinite, this group is infinite too. There exist finitely-generated, and even finitely-presented, infinite simple groups. Every group can be imbedded in a simple group. The definition of a simple group given here differs somewhat from that given in the theory of Lie groups and algebraic groups (cf. Lie group, semi-simple).

Comments

In the theory of infinite groups two notions stronger than simplicity are used, viz. those of an absolutely simple group and a strictly simple group. One has the implications: absolutely simple 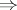strictly simple simple. There are examples of simple groups that are not absolutely simple and of simple groups that are not strictly simple.

A group is strictly simple if it has no non-trivial ascendent subgroup; it is absolutely simple if it has no non-trivial serial subgroup. Cf. [a6] for more details.

An algebraic group over an algebraically closed field is simple if it has no closed non-trivial normal subgroup. It is quasi-simple, or almost simple, if it has no non-trivial infinite normal subgroup. If 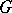is almost simple, then the abstract group 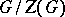, where is the centre, is simple as an abstract group.

A Lie group is simple if it has no non-trivial Lie subgroup. For a connected Lie group this is the same as simplicity of its Lie algebra.

A topological group is called simple if it has no proper closed normal subgroup.

Both for algebraic groups and topological groups one also finds in the literature the definition that such a group is simple if it has no non-trivial closed connected normal subgroup.

References

[a1]	J.E. Humphreys, "Linear algebraic groups" , Springer (1975) pp. Sect. 35.1 MR0396773 Zbl 0325.20039
[a2]	L.S. Pontryagin, "Topological groups" , Princeton Univ. Press (1939) (Translated from Russian) MR0201557 Zbl 0022.17104
[a3]	G.W. Mackey, "Unitary group representations" , Benjamin/Cummings (1978) MR0515581 Zbl 0401.22001
[a4]	H. Freudenthal, H. de Vries, "Linear Lie groups" , Acad. Press (1969) MR0260926 Zbl 0377.22001
[a5]	M. Weinstein, "Examples of groups" , Polygonal (1977) pp. Examples 6.14; 6.15 MR0453847 Zbl 0359.20001
[a6]	D.J.S. Robinson, "Finiteness condition and generalized soluble groups" , 1 , Springer (1972) pp. Chapt. 1 MR0332990 MR0332989