Hamilton-Ostrogradski principle
principle of stationary action
A general integral variational principle of classical mechanics (cf. Variational principles of classical mechanics), established by W. Hamilton [1] for holonomic systems restricted by ideal stationary constraints, and generalized by M.V. Ostrogradski [2] to non-stationary geometrical constraints. According to this principle, in a real motion of the system acted upon by potential forces,
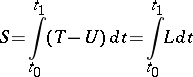 |
has a stationary value as compared with near, kinetically-possible, motions, with initial and final positions of the system and times of motion identical with those for the real motion. Here, 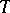 is the kinetic energy,
is the kinetic energy, 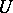 is the potential energy and
is the potential energy and 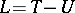 is the Lagrange function of the system. In certain cases the true motion corresponds not only to a stationary point of the functional
is the Lagrange function of the system. In certain cases the true motion corresponds not only to a stationary point of the functional 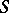 , but corresponds to its smallest value. For this reason the Hamilton–Ostrogradski principle is sometimes called the principle of least action. In the case of non-potential active forces
, but corresponds to its smallest value. For this reason the Hamilton–Ostrogradski principle is sometimes called the principle of least action. In the case of non-potential active forces  the condition of stationary action,
the condition of stationary action, 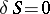 , is replaced by the condition
, is replaced by the condition
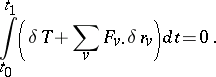 |
References
| [1] | W. Hamilton, , Report of the 4-th meeting of the British Association for the Advancement of Science , London (1835) pp. 513–518 |
| [2] | M. Ostrogradski, Mem. Acad. Sci. St. Petersbourg , 8 : 3 (1850) pp. 33–48 |
Comments
In English-language literature this principle goes by the name of Hamilton principle.
References
| [a1] | V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) |
Hamilton-Ostrogradski principle. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Hamilton-Ostrogradski_principle&oldid=16710