Random event
event
Any combination of outcomes of an experiment that has a definite probability of occurrence.
Example 1. In the throwing of two dice, each of the 36 outcomes can be represented as a pair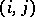 
, where 
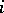is the number of dots on the upper face of the first dice and 
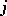the number on the second. The event "the sum of the dots is equal to 11" is just the combination of the two outcomes 
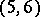and 
.
Example 2. In the random throwing of two points into an interval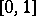 
, the set of all outcomes can be represented as the set of points 
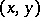(where 
is the value of the first point and 
that of the second) in the square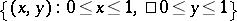 
. The event "the length of the interval joining x and y is less than a, 0<a< 1" is just the set of points in the square whose distance from the diagonal passing through the origin is less than 
.
Within the limits of the generally accepted axiomatics of probability theory (see [1]), where at the base of the probability model lies a probability space 
(
is a space of elementary events, i.e. the set of all possible outcomes of a given experiment, 
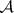is a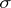 
-algebra of subsets of 
and 
is a probability measure defined on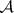 
), random events are just the sets which belong to 
.
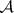In the first of the above examples, 
is a finite set of 36 elements: the pairs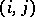 
, 
; 
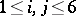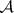is the class of all 
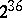subsets of 
(including 
itself and the empty set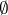 
), and for every 
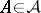the probability 
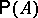is equal to 
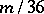, where 
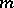is the number of elements of 
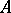. In the second example, 
is the set of points in the unit square, 
is the class of its Borel subsets and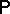 
is ordinary Lebesgue measure on 
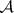(which for simple figures coincides with their area).
The class 
of events associated with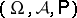 
forms a Boolean ring with identity with respect to the operations 
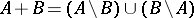(symmetric difference) and 
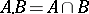(it has a multiplicative identity 
), that is, it forms a Boolean algebra. The function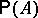 
defined on this Boolean algebra has all the properties of a norm except one: it does not follow from 
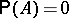that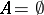 
. By declaring two events to be equivalent if the 
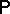-measure of their symmetric difference is zero, and considering equivalence classes 
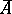instead of events 
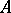, one obtains the normalized Boolean algebra 
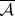of classes 
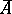. This observation leads to another possible approach to the axiomatics of probability theory, in which the basic object is not the probability space connected with a given experiment, but a normalized Boolean algebra of random events (see [2], [3]).
References
| [1] | A.N. Kolmogorov, "Foundations of the theory of probability" , Chelsea, reprint (1950) (Translated from Russian) |
| [2] | B.V. Gnedenko, A.N. Kolmogorov, "Probability theory" , Mathematics in the USSR during thirty years: 1917–1947 , Moscow-Leningrad (1948) pp. 701–727 (In Russian) |
| [3] | A.N. Kolmogorov, "Algèbres de Boole métriques complètes" , VI Zjazd Mathematyków Polskich , Kraków (1950) |
| [4] | P.R. Halmos, "Measure theory" , v. Nostrand (1950) |
Comments
References
| [a1] | W. Feller, "An introduction to probability theory and its applications", 1, Wiley (1957) |
| [a2] | H. Bauer, "Probability theory and elements of measure theory", Holt, Rinehart & Winston (1972) pp. Chapt. 11 (Translated from German) |
Random event. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Random_event&oldid=13100