Gâteaux gradient
From Encyclopedia of Mathematics
Revision as of 18:52, 24 March 2012 by Ulf Rehmann (talk | contribs) (moved Gâteaux gradient to Gateaux gradient: ascii title)
of a functional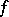 
at a point 
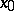of a Hilbert space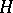 
The vector in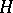 
equal to the Gâteaux derivative 
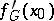of 
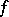at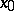 
. In other words, the Gâteaux gradient is defined by the formula
 |
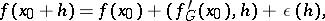where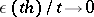 
as 
. In an 
-dimensional Euclidean space the Gâteaux gradient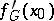 
is the vector with coordinates
 |
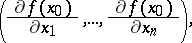and is simply known as the gradient. The concept of the Gâteaux gradient may be extended to the case when 
is a Riemannian manifold (finite-dimensional) or an infinite-dimensional Hilbert manifold and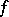 
is a smooth real function on 
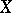. The growth of 
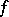in the direction of its Gâteaux gradient is larger than in any other direction passing through the point 
.
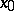How to Cite This Entry:
Gâteaux gradient. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=G%C3%A2teaux_gradient&oldid=12952
Gâteaux gradient. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=G%C3%A2teaux_gradient&oldid=12952
This article was adapted from an original article by V.M. Tikhomirov (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article