Vector field on a manifold
A section of the tangent bundle 
. The set of differentiable vector fields forms a module over the ring 
of differentiable functions on 
.
Example 1.
For a chart 
of the manifold 
one defines the 
-th basic vector field 
according to the formula
 |
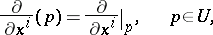where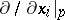 
is the 
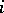-th basic tangent vector to 
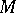at the point 
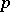. Any vector field 
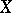can be uniquely represented in the form
 |
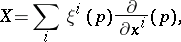where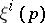 
are the components of 
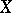in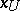 
. Since a vector field can be regarded as a derivation of the ring 
(see example 2), the set of vector fields forms a Lie algebra with respect to the commutation operation (the Lie bracket).
Example 2.
For the chart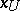 
and 
, the function 
is defined by the formula
 |
 |
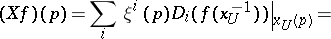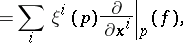where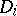 
is the partial derivative with respect to 
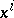. Note that 
; 
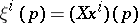is called the derivative of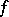 
in the direction 
.
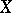Example 3.
For the chart 
and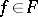 
, the commutator (Lie bracket) 
of the vector fields
 |
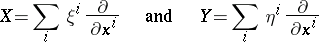is defined by the formula
 |
 |
It satisfies the relations
 |
 |
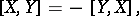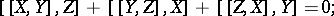in particular,
 |
Each vector field 
induces a local flow on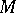 
— a family of diffeomorphisms of a neighbourhood 
,
 |
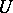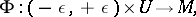such that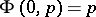 
for 
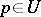and
 |
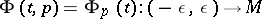is the integral curve of the vector field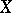 
through 
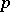, i.e.
 |
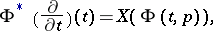where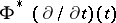 
is the tangent vector 
to 
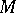at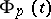 
. Conversely, a vector field 
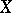is associated with a local flow 
, which is a variation of the mapping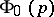 
; here
 |
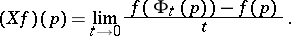Each vector field defines a Lie derivation 
of a tensor field of type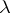 
with values in a vector space (infinitesimal transformation of 
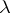), corresponding to the local flow 
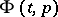; its special cases include the action of the vector field on 
,
 |
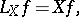and the Lie bracket
 |
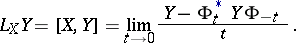A vector field without singularities generates an integrable one-dimensional differential system as well as a Pfaffian system associated with it on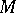 
.
A generalization of the concept of a vector field on a manifold is that of a vector field along a mapping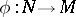 
, which is a section of the bundle 
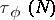induced by 
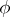, as well as a tensor field of type 
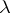, which is a section of the bundle 
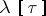associated with 
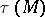with the aid of the functor 
.
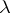References
| [1] | C. Godbillon, "Géométrie différentielle et mécanique analytique" , Hermann (1969) MR0242081 Zbl 0653.53001 Zbl 0284.53018 |
| [2] | D. Gromoll, W. Klingenberg, W. Meyer, "Riemannsche Geometrie im Grossen" , Springer (1968) MR0229177 Zbl 0155.30701 |
| [3] | S. Lang, "Introduction to differentiable manifolds" , Interscience (1967) pp. App. III MR1931083 MR1532744 MR0155257 Zbl 1008.57001 Zbl 0103.15101 |
| [4] | K. Nomizu, "Lie groups and differential geometry" , Math. Soc. Japan (1956) MR0084166 Zbl 0071.15402 |
| [5] | M.M. Postnikov, "Introduction to Morse theory" , Moscow (1971) (In Russian) MR0315739 |
| [6] | S. Helgason, "Differential geometry and symmetric spaces" , Acad. Press (1962) MR0145455 Zbl 0111.18101 |
Comments
References
| [a1] | W. Klingenberg, "Riemannian geometry" , de Gruyter (1982) (Translated from German) MR0666697 Zbl 0495.53036 |
Vector field on a manifold. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Vector_field_on_a_manifold&oldid=11561