Difference between revisions of "Tomography"
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
Ulf Rehmann (talk | contribs) m (Undo revision 48984 by Ulf Rehmann (talk)) Tag: Undo |
||
| Line 1: | Line 1: | ||
| − | < | + | Reconstruction from projections, i.e. the recovery of a function from its line or (hyper)plane integrals. Important issues are existence, uniqueness and stability of inversion procedures, as well as the development of efficient numerical algorithms. In tomography a fundamental role is played by the [[Radon transform|Radon transform]] on Euclidean space. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929801.png" /> be a continuous function of the real variables <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929802.png" /> that is decreasing sufficiently fast at infinity, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929803.png" />; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929804.png" />. Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929805.png" /> be a unit vector in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929806.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929807.png" /> a real number and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929808.png" /> a hyperplane, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t0929809.png" /> denotes the Euclidean inner product. The integral of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298010.png" /> over the hyperplane <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298011.png" />, |
| − | t0929801.png | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298012.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a1)</td></tr></table> | |
| − | |||
| − | + | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298013.png" /> is the Euclidean measure on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298014.png" />, is called the Radon transform of the function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298015.png" />. The Radon operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298016.png" /> maps the space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298017.png" /> of rapidly-decreasing <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298018.png" />-functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298019.png" /> to the space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298020.png" /> of rapidly-decreasing <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298021.png" />-functions on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298022.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298023.png" /> is the unit cylinder in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298024.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298025.png" /> the unit sphere in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298026.png" />. The integral <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298027.png" />, with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298028.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298029.png" /> fixed, is called a projection. The Radon transform is connected to the [[Fourier transform|Fourier transform]] through the central slice theorem: | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | a | ||
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298030.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a2)</td></tr></table> | |
| − | |||
| − | |||
| − | where | + | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298031.png" /> denotes the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298032.png" />-dimensional Fourier transform of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298033.png" />. To the Radon operator is associated a dual or backprojection operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298034.png" />, by |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | of | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298035.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a3)</td></tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | Whereas <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298036.png" /> integrates over all points in a hyperplane, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298037.png" /> integrates over all hyperplanes through a point. Thus <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298038.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298039.png" /> form a dual pair in the sense of [[Integral geometry|integral geometry]] (see [[#References|[a1]]], [[#References|[a8]]], [[#References|[a9]]]). Related transforms are the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298041.png" />-plane transform, which integrates a function over <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298042.png" />-dimensional subspaces (the case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298043.png" /> is called the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298045.png" />-ray transform; the case <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298046.png" /> is identical to the Radon transform), and the divergent beam transform, which integrates over half-lines. | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | integrates over all points in a hyperplane, | ||
| − | integrates over all hyperplanes through a point. Thus | ||
| − | |||
| − | form a dual pair in the sense of [[Integral geometry|integral geometry]] (see [[#References|[a1]]], [[#References|[a8]]], [[#References|[a9]]]). Related transforms are the | ||
| − | plane transform, which integrates a function over | ||
| − | dimensional subspaces (the case | ||
| − | is called the | ||
| − | ray transform; the case | ||
| − | is identical to the Radon transform), and the divergent beam transform, which integrates over half-lines. | ||
==Inversion.== | ==Inversion.== | ||
The inversion formula of the Radon transform takes two different forms, depending on whether the dimension is even or odd [[#References|[a12]]], [[#References|[a17]]]: | The inversion formula of the Radon transform takes two different forms, depending on whether the dimension is even or odd [[#References|[a12]]], [[#References|[a17]]]: | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298047.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298048.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | Here | + | Here <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298049.png" /> denotes the <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298050.png" />-st derivative of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298051.png" /> with respect to its last (scalar) argument. So, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298052.png" /> odd only local information in a neighbourhood of the point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298053.png" /> is needed, whereas for even <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298054.png" /> the integrals along all hyperplanes meeting the support of the function are required. In his 1917 paper, J. Radon [[#References|[a17]]] also discussed the problem of determining a function on the hyperbolic plane from its integrals over all geodesics. Previously, a similar method had been used by P. Funk [[#References|[a5]]] for the elliptic case of reconstructing an even function on the sphere from the integrals along the great circles. A unified form of the inversion formula for odd and even <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298055.png" /> is given by |
| − | denotes the | ||
| − | st derivative of | ||
| − | with respect to its last (scalar) argument. So, for | ||
| − | odd only local information in a neighbourhood of the point | ||
| − | is needed, whereas for even | ||
| − | the integrals along all hyperplanes meeting the support of the function are required. In his 1917 paper, J. Radon [[#References|[a17]]] also discussed the problem of determining a function on the hyperbolic plane from its integrals over all geodesics. Previously, a similar method had been used by P. Funk [[#References|[a5]]] for the elliptic case of reconstructing an even function on the sphere from the integrals along the great circles. A unified form of the inversion formula for odd and even | ||
| − | is given by | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298056.png" /></td> <td valign="top" style="width:5%;text-align:right;">(a4)</td></tr></table> | |
| − | |||
| − | |||
| − | |||
| − | where | + | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298057.png" /> (with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298058.png" /> the gamma-function and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298059.png" /> the [[Laplace operator|Laplace operator]] (acting on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298060.png" />)), see [[#References|[a8]]]. Similar expressions for the dual transform exist. Inversion formulas for the divergent beam and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298061.png" />-ray transforms are analogous to those for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298062.png" />. Other inversion formulas for the Radon transform are obtained by analytic series expansion methods, see [[#References|[a3]]]. |
| − | with | ||
| − | the gamma-function and | ||
| − | the [[Laplace operator|Laplace operator]] (acting on | ||
| − | see [[#References|[a8]]]. Similar expressions for the dual transform exist. Inversion formulas for the divergent beam and | ||
| − | ray transforms are analogous to those for | ||
| − | Other inversion formulas for the Radon transform are obtained by analytic series expansion methods, see [[#References|[a3]]]. | ||
| − | From the inversion formulas it follows that | + | From the inversion formulas it follows that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298063.png" /> is uniquely determined by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298064.png" />. If the transform is only known on a subset of the domain, this may no longer be true. Some of these so-called incomplete data problems are as follows (cf. [[Potential theory, inverse problems in|Potential theory, inverse problems in]]). i) The exterior problem, i.e. recovering a function in the exterior of some ball from integrals over lines or planes outside that ball. This problem is uniquely solvable, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298065.png" /> is decaying fast enough at infinity. ii) The interior problem, i.e. determining <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298066.png" /> inside some ball from integrals inside that ball. A unique solution exists for odd dimension only. iii) The limited angle problem, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298067.png" /> is given only for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298068.png" /> in a subset of a half-sphere. Uniqueness holds as long as the set of directions is such that no non-trivial homogeneous polynomial vanishes on it. iv) Problems with a finite number of directions. The solution is non-unique to a very large extent. In fact, for any finite set of directions and any function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298069.png" />, with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298070.png" /> a compact set in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298071.png" />, and any compact set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298072.png" /> in the interior of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298073.png" />, one can find a function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298074.png" /> which coincides with <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298075.png" /> on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298076.png" /> and for which all the line integrals along the given directions are identically zero, see [[#References|[a20]]]. Such (highly oscillatory) functions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298077.png" />, sometimes called "ghostghosts" , form the null-space of the transform for finitely many projections. To resolve this indeterminacy one has to put restrictions on the variation of the function <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298078.png" />. On the other hand, by increasing the number of projections, arbitrarily good approximations to the unknown function can be found. |
| − | is uniquely determined by | ||
| − | If the transform is only known on a subset of the domain, this may no longer be true. Some of these so-called incomplete data problems are as follows (cf. [[Potential theory, inverse problems in|Potential theory, inverse problems in]]). i) The exterior problem, i.e. recovering a function in the exterior of some ball from integrals over lines or planes outside that ball. This problem is uniquely solvable, if | ||
| − | is decaying fast enough at infinity. ii) The interior problem, i.e. determining | ||
| − | inside some ball from integrals inside that ball. A unique solution exists for odd dimension only. iii) The limited angle problem, where | ||
| − | is given only for | ||
| − | in a subset of a half-sphere. Uniqueness holds as long as the set of directions is such that no non-trivial homogeneous polynomial vanishes on it. iv) Problems with a finite number of directions. The solution is non-unique to a very large extent. In fact, for any finite set of directions and any function | ||
| − | with | ||
| − | a compact set in | ||
| − | and any compact set | ||
| − | in the interior of | ||
| − | one can find a function | ||
| − | which coincides with | ||
| − | on | ||
| − | and for which all the line integrals along the given directions are identically zero, see [[#References|[a20]]]. Such (highly oscillatory) functions | ||
| − | sometimes called "ghostghosts" , form the null-space of the transform for finitely many projections. To resolve this indeterminacy one has to put restrictions on the variation of the function | ||
| − | On the other hand, by increasing the number of projections, arbitrarily good approximations to the unknown function can be found. | ||
==Reconstruction algorithms.== | ==Reconstruction algorithms.== | ||
| − | The identity | + | The identity <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298079.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298080.png" /> denotes convolution (cf. [[Convolution of functions|Convolution of functions]]), is the basis of filtered backprojection. One chooses <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298081.png" /> in such a way that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298082.png" /> is a low-pass filter approaching a delta-distribution, so that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298083.png" /> is recovered by a convolution followed by backprojection (both operations properly discretized). Variants exist where the backprojection is performed before the filtering procedure. The central slice theorem is the basis of Fourier reconstruction. Here the projections <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298084.png" /> are Fourier transformed with respect to the last variable, which is allowed by the inverse <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298085.png" />-dimensional Fourier transform. To make use of the fast Fourier transform (cf. [[Fourier transform, discrete|Fourier transform, discrete]]), one first has to interpolate from a polar grid to a Cartesian grid. Analytic series expansion methods are based upon discretization of the inversion formulas for the individual expansion coefficients of the unknown function. Algebraic reconstruction techniques use iterative methods from numerical linear algebra (cf. [[Iteration methods|Iteration methods]]) applied to the system of linear equations resulting from a full discretization of Radon's integral equation. |
| − | where | ||
| − | denotes convolution (cf. [[Convolution of functions|Convolution of functions]]), is the basis of filtered backprojection. One chooses | ||
| − | in such a way that | ||
| − | is a low-pass filter approaching a delta-distribution, so that | ||
| − | is recovered by a convolution followed by backprojection (both operations properly discretized). Variants exist where the backprojection is performed before the filtering procedure. The central slice theorem is the basis of Fourier reconstruction. Here the projections | ||
| − | are Fourier transformed with respect to the last variable, which is allowed by the inverse | ||
| − | dimensional Fourier transform. To make use of the fast Fourier transform (cf. [[Fourier transform, discrete|Fourier transform, discrete]]), one first has to interpolate from a polar grid to a Cartesian grid. Analytic series expansion methods are based upon discretization of the inversion formulas for the individual expansion coefficients of the unknown function. Algebraic reconstruction techniques use iterative methods from numerical linear algebra (cf. [[Iteration methods|Iteration methods]]) applied to the system of linear equations resulting from a full discretization of Radon's integral equation. | ||
Problems in computerized tomography are ill-posed (cf. [[Ill-posed problems|Ill-posed problems]]). There may be no solution if the data are not in the range of the transform, or the solution may be non-unique, problems which can be overcome by using the concept of generalized inverses. Moreover, the operator which maps the transform to the solution may not be continuous. In that case one uses regularization procedures, such as Tikhonov–Phillips regularization, iterative methods with appropriate stopping criteria or truncated [[singular value decomposition]]s [[#References|[a4]]], [[#References|[a15]]]. Limited data problems in particular are severely ill-posed. In this context one also may apply various statistical estimation procedures with or without the use of a-priori information, often resulting in iterative algorithms. | Problems in computerized tomography are ill-posed (cf. [[Ill-posed problems|Ill-posed problems]]). There may be no solution if the data are not in the range of the transform, or the solution may be non-unique, problems which can be overcome by using the concept of generalized inverses. Moreover, the operator which maps the transform to the solution may not be continuous. In that case one uses regularization procedures, such as Tikhonov–Phillips regularization, iterative methods with appropriate stopping criteria or truncated [[singular value decomposition]]s [[#References|[a4]]], [[#References|[a15]]]. Limited data problems in particular are severely ill-posed. In this context one also may apply various statistical estimation procedures with or without the use of a-priori information, often resulting in iterative algorithms. | ||
==Generalizations.== | ==Generalizations.== | ||
| − | Generalized Radon transforms can be defined, where the integration is over subspaces or, more generally, over manifolds [[#References|[a6]]], [[#References|[a8]]], [[#References|[a12]]]. A related problem for such spaces is the orbital integral problem, i.e. recovering a function from its integrals over (generalized) spheres. In acoustic or diffraction tomography, which is a special inverse scattering problem, | + | Generalized Radon transforms can be defined, where the integration is over subspaces or, more generally, over manifolds [[#References|[a6]]], [[#References|[a8]]], [[#References|[a12]]]. A related problem for such spaces is the orbital integral problem, i.e. recovering a function from its integrals over (generalized) spheres. In acoustic or diffraction tomography, which is a special inverse scattering problem, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298086.png" /> can be recovered from data on semi-circles through the origin (see [[#References|[a14]]], [[#References|[a18]]], [[#References|[a21]]]). Another variant is the attenuated Radon transform, which differs from the standard Radon transform by the presence of an exponential weighting factor under the integral sign. For the use of the Radon transform in the area of partial differential equations, see [[#References|[a8]]], [[#References|[a13]]]. One more generalization of Radon's problem is the reconstruction of a measure from its projections, i.e. from lower-dimensional measures induced by some measurable mapping. For the Radon transform in the complex domain, see [[#References|[a6]]]. Finite Radon transforms have also been studied. |
| − | can be recovered from data on semi-circles through the origin (see [[#References|[a14]]], [[#References|[a18]]], [[#References|[a21]]]). Another variant is the attenuated Radon transform, which differs from the standard Radon transform by the presence of an exponential weighting factor under the integral sign. For the use of the Radon transform in the area of partial differential equations, see [[#References|[a8]]], [[#References|[a13]]]. One more generalization of Radon's problem is the reconstruction of a measure from its projections, i.e. from lower-dimensional measures induced by some measurable mapping. For the Radon transform in the complex domain, see [[#References|[a6]]]. Finite Radon transforms have also been studied. | ||
==Applications.== | ==Applications.== | ||
| − | Tomography is a widely used method to reconstruct cross-sections of the interior structure of an object without having to cut or damage the object. In this context one usually speaks of computerized (computed, computer assisted) tomography, since for actually performing the reconstructions in practice one needs to use a digital computer. Through the interaction of a physical agens or "probe" — varying from | + | Tomography is a widely used method to reconstruct cross-sections of the interior structure of an object without having to cut or damage the object. In this context one usually speaks of computerized (computed, computer assisted) tomography, since for actually performing the reconstructions in practice one needs to use a digital computer. Through the interaction of a physical agens or "probe" — varying from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t092/t092980/t09298087.png" />-rays, gamma rays, visible light, electrons, or neutrons to ultrasound waves or nuclear magnetic resonance signals — with the object, one obtains line or plane integrals of the internal distribution to be reconstructed. One of the most prominent examples of computerized tomography occurs in diagnostic medicine, where the method is used to produce images of the interior of human organs. In industry the method is used for non-destructive testing. Other applications arise in radio astronomy, radar theory, electron microscopy, aerodynamics, geophysics, and oceanography, see [[#References|[a3]]], [[#References|[a10]]], [[#References|[a14]]] for references. Finite Radon transforms are used in various areas such as the theory of error-correcting codes and lattice theory. |
| − | rays, gamma rays, visible light, electrons, or neutrons to ultrasound waves or nuclear magnetic resonance signals — with the object, one obtains line or plane integrals of the internal distribution to be reconstructed. One of the most prominent examples of computerized tomography occurs in diagnostic medicine, where the method is used to produce images of the interior of human organs. In industry the method is used for non-destructive testing. Other applications arise in radio astronomy, radar theory, electron microscopy, aerodynamics, geophysics, and oceanography, see [[#References|[a3]]], [[#References|[a10]]], [[#References|[a14]]] for references. Finite Radon transforms are used in various areas such as the theory of error-correcting codes and lattice theory. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R.L. Bryant (ed.) V. Guillemin (ed.) S. Helgason (ed.) R.O. Wells jr. (ed.) , ''Integral geometry'' , Amer. Math. Soc. (1987)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> Y. Censor, T. Elfving, G.T. (eds.) Herman, "Linear algebra in image reconstruction from projections" ''Lin. Alg. Appl.'' , '''130''' (1990) pp. 1–305 (special issue)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> S.R. Deans, "The Radon transform and some of its applications" , Wiley (1983)</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> H.W. Engl (ed.) C.W. Groetsch (ed.) , ''Inverse and ill-posed problems'' , Acad. Press (1987)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> P. Funk, "Über Flächen mit lauter geschlossenen geodätischen Linien" ''Math. Ann.'' , '''74''' (1913) pp. 287–300</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> I.M. Gel'fand, M.I. Graev, N.Ya. Vilenkin, "Generalized functions" , '''5. Integral geometry and representation theory''' , Acad. Press (1966) (Translated from Russian)</TD></TR><TR><TD valign="top">[a7a]</TD> <TD valign="top"> A.B. Goncharev, "Integral geometry and three-dimensional reconstruction of randomly oriented identical particles from their electron microphotos" ''Acta Appl. Math.'' , '''11''' (1988) pp. 199–211</TD></TR><TR><TD valign="top">[a7b]</TD> <TD valign="top"> A.B. Goncharev, "Methods of integral geometry and recovering a function with compact support from its projections in unknown directions" ''Acta Appl. Math.'' , '''11''' (1988) pp. 213–222</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> S. Helgason, "The Radon transform" , Birkhäuser (1980)</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> S. Helgason, "Groups and geometric analysis" , Acad. Press (1984) pp. Chapt. II, Sect. 4</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> G.T. Herman, "Image reconstruction from projections, the fundamentals of computerized tomography" , Acad. Press (1980)</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> G.T. Herman (ed.) F. Natterer (ed.) , ''Mathematical aspects of computerized tomography, Proc. Oberwolfach, February 10–16, 1980'' , Springer (1981)</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> F. John, "Bestimmung einer Funktion aus ihren Integralen über gewisse Mannigfaltigkeiten" ''Math. Ann.'' , '''109''' (1934) pp. 488–520</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> F. John, "Plane waves and spherical means applied to partial differential equations" , Interscience (1955)</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> A.C. Kak, A.M. Slaney, "Principles of computerized tomography imaging" , IEEE (1988)</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> A.K. Louis, "Inverse und schlecht gestellte Probleme" , Teubner (1989)</TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> F. Natterer, "The mathematics of computerized tomography" , Teubner & Wiley (1986)</TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> J. Radon, "Über die Bestimmung von Funktionen durch ihre Integralwerte längs gewisser Mannigfaltigkeiten" ''Ber. Sächs. Akad. Wissenschaft. Leipzig Math. Phys. Kl.'' , '''69''' (1917) pp. 262–267</TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> P.C. Sabatier, "Basic methods of tomography and inverse problems" , A. Hilger (1987)</TD></TR><TR><TD valign="top">[a19]</TD> <TD valign="top"> L.A. Shepp (ed.) , ''Computed tomography'' , ''Proc. Symp. Appl. Math.'' , '''27''' , Amer. Math. Soc. (1983)</TD></TR><TR><TD valign="top">[a20]</TD> <TD valign="top"> K.T. Smith, D.C. Solmon, S.L. Wagner, "Practical and mathematical aspects of the problem of reconstructing a function from radiographs" ''Bull. Amer. Math. Soc. (N.S.)'' , '''83''' (1977) pp. 1227–1270</TD></TR><TR><TD valign="top">[a21]</TD> <TD valign="top"> H. Stark, "Image recovery: theory and application" , Acad. Press (1987)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R.L. Bryant (ed.) V. Guillemin (ed.) S. Helgason (ed.) R.O. Wells jr. (ed.) , ''Integral geometry'' , Amer. Math. Soc. (1987)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> Y. Censor, T. Elfving, G.T. (eds.) Herman, "Linear algebra in image reconstruction from projections" ''Lin. Alg. Appl.'' , '''130''' (1990) pp. 1–305 (special issue)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> S.R. Deans, "The Radon transform and some of its applications" , Wiley (1983)</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> H.W. Engl (ed.) C.W. Groetsch (ed.) , ''Inverse and ill-posed problems'' , Acad. Press (1987)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> P. Funk, "Über Flächen mit lauter geschlossenen geodätischen Linien" ''Math. Ann.'' , '''74''' (1913) pp. 287–300</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> I.M. Gel'fand, M.I. Graev, N.Ya. Vilenkin, "Generalized functions" , '''5. Integral geometry and representation theory''' , Acad. Press (1966) (Translated from Russian)</TD></TR><TR><TD valign="top">[a7a]</TD> <TD valign="top"> A.B. Goncharev, "Integral geometry and three-dimensional reconstruction of randomly oriented identical particles from their electron microphotos" ''Acta Appl. Math.'' , '''11''' (1988) pp. 199–211</TD></TR><TR><TD valign="top">[a7b]</TD> <TD valign="top"> A.B. Goncharev, "Methods of integral geometry and recovering a function with compact support from its projections in unknown directions" ''Acta Appl. Math.'' , '''11''' (1988) pp. 213–222</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> S. Helgason, "The Radon transform" , Birkhäuser (1980)</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> S. Helgason, "Groups and geometric analysis" , Acad. Press (1984) pp. Chapt. II, Sect. 4</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> G.T. Herman, "Image reconstruction from projections, the fundamentals of computerized tomography" , Acad. Press (1980)</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> G.T. Herman (ed.) F. Natterer (ed.) , ''Mathematical aspects of computerized tomography, Proc. Oberwolfach, February 10–16, 1980'' , Springer (1981)</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> F. John, "Bestimmung einer Funktion aus ihren Integralen über gewisse Mannigfaltigkeiten" ''Math. Ann.'' , '''109''' (1934) pp. 488–520</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> F. John, "Plane waves and spherical means applied to partial differential equations" , Interscience (1955)</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> A.C. Kak, A.M. Slaney, "Principles of computerized tomography imaging" , IEEE (1988)</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> A.K. Louis, "Inverse und schlecht gestellte Probleme" , Teubner (1989)</TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> F. Natterer, "The mathematics of computerized tomography" , Teubner & Wiley (1986)</TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> J. Radon, "Über die Bestimmung von Funktionen durch ihre Integralwerte längs gewisser Mannigfaltigkeiten" ''Ber. Sächs. Akad. Wissenschaft. Leipzig Math. Phys. Kl.'' , '''69''' (1917) pp. 262–267</TD></TR><TR><TD valign="top">[a18]</TD> <TD valign="top"> P.C. Sabatier, "Basic methods of tomography and inverse problems" , A. Hilger (1987)</TD></TR><TR><TD valign="top">[a19]</TD> <TD valign="top"> L.A. Shepp (ed.) , ''Computed tomography'' , ''Proc. Symp. Appl. Math.'' , '''27''' , Amer. Math. Soc. (1983)</TD></TR><TR><TD valign="top">[a20]</TD> <TD valign="top"> K.T. Smith, D.C. Solmon, S.L. Wagner, "Practical and mathematical aspects of the problem of reconstructing a function from radiographs" ''Bull. Amer. Math. Soc. (N.S.)'' , '''83''' (1977) pp. 1227–1270</TD></TR><TR><TD valign="top">[a21]</TD> <TD valign="top"> H. Stark, "Image recovery: theory and application" , Acad. Press (1987)</TD></TR></table> | ||
Revision as of 14:53, 7 June 2020
Reconstruction from projections, i.e. the recovery of a function from its line or (hyper)plane integrals. Important issues are existence, uniqueness and stability of inversion procedures, as well as the development of efficient numerical algorithms. In tomography a fundamental role is played by the Radon transform on Euclidean space. Let 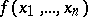 be a continuous function of the real variables
be a continuous function of the real variables 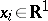 that is decreasing sufficiently fast at infinity,
that is decreasing sufficiently fast at infinity,  ;
; 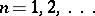 . Let
. Let 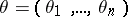 be a unit vector in
be a unit vector in 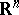 ,
,  a real number and
a real number and 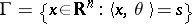 a hyperplane, where
a hyperplane, where 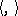 denotes the Euclidean inner product. The integral of
denotes the Euclidean inner product. The integral of  over the hyperplane
over the hyperplane 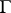 ,
,
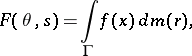 | (a1) |
where 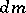 is the Euclidean measure on
is the Euclidean measure on 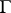 , is called the Radon transform of the function
, is called the Radon transform of the function 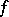 . The Radon operator
. The Radon operator  maps the space
maps the space 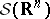 of rapidly-decreasing
of rapidly-decreasing 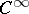 -functions on
-functions on 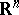 to the space
to the space 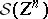 of rapidly-decreasing
of rapidly-decreasing 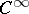 -functions on
-functions on 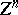 , where
, where 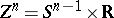 is the unit cylinder in
is the unit cylinder in 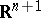 and
and  the unit sphere in
the unit sphere in  . The integral
. The integral 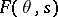 , with
, with 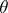 and
and  fixed, is called a projection. The Radon transform is connected to the Fourier transform through the central slice theorem:
fixed, is called a projection. The Radon transform is connected to the Fourier transform through the central slice theorem:
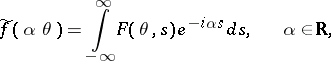 | (a2) |
where 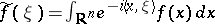 denotes the
denotes the  -dimensional Fourier transform of
-dimensional Fourier transform of  . To the Radon operator is associated a dual or backprojection operator
. To the Radon operator is associated a dual or backprojection operator 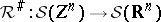 , by
, by
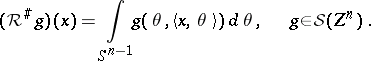 | (a3) |
Whereas 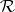 integrates over all points in a hyperplane,
integrates over all points in a hyperplane, 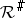 integrates over all hyperplanes through a point. Thus
integrates over all hyperplanes through a point. Thus 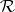 ,
, 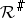 form a dual pair in the sense of integral geometry (see [a1], [a8], [a9]). Related transforms are the
form a dual pair in the sense of integral geometry (see [a1], [a8], [a9]). Related transforms are the  -plane transform, which integrates a function over
-plane transform, which integrates a function over 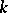 -dimensional subspaces (the case
-dimensional subspaces (the case 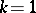 is called the
is called the 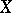 -ray transform; the case
-ray transform; the case 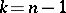 is identical to the Radon transform), and the divergent beam transform, which integrates over half-lines.
is identical to the Radon transform), and the divergent beam transform, which integrates over half-lines.
Inversion.
The inversion formula of the Radon transform takes two different forms, depending on whether the dimension is even or odd [a12], [a17]:
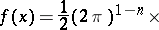 |
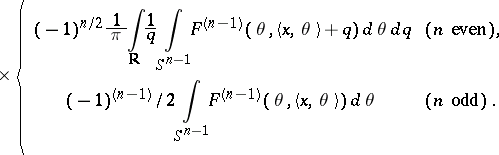 |
Here  denotes the
denotes the 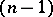 -st derivative of
-st derivative of 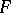 with respect to its last (scalar) argument. So, for
with respect to its last (scalar) argument. So, for  odd only local information in a neighbourhood of the point
odd only local information in a neighbourhood of the point  is needed, whereas for even
is needed, whereas for even  the integrals along all hyperplanes meeting the support of the function are required. In his 1917 paper, J. Radon [a17] also discussed the problem of determining a function on the hyperbolic plane from its integrals over all geodesics. Previously, a similar method had been used by P. Funk [a5] for the elliptic case of reconstructing an even function on the sphere from the integrals along the great circles. A unified form of the inversion formula for odd and even
the integrals along all hyperplanes meeting the support of the function are required. In his 1917 paper, J. Radon [a17] also discussed the problem of determining a function on the hyperbolic plane from its integrals over all geodesics. Previously, a similar method had been used by P. Funk [a5] for the elliptic case of reconstructing an even function on the sphere from the integrals along the great circles. A unified form of the inversion formula for odd and even  is given by
is given by
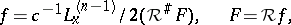 | (a4) |
where 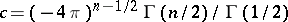 (with
(with 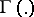 the gamma-function and
the gamma-function and 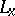 the Laplace operator (acting on
the Laplace operator (acting on  )), see [a8]. Similar expressions for the dual transform exist. Inversion formulas for the divergent beam and
)), see [a8]. Similar expressions for the dual transform exist. Inversion formulas for the divergent beam and 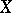 -ray transforms are analogous to those for
-ray transforms are analogous to those for 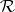 . Other inversion formulas for the Radon transform are obtained by analytic series expansion methods, see [a3].
. Other inversion formulas for the Radon transform are obtained by analytic series expansion methods, see [a3].
From the inversion formulas it follows that 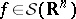 is uniquely determined by
is uniquely determined by 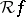 . If the transform is only known on a subset of the domain, this may no longer be true. Some of these so-called incomplete data problems are as follows (cf. Potential theory, inverse problems in). i) The exterior problem, i.e. recovering a function in the exterior of some ball from integrals over lines or planes outside that ball. This problem is uniquely solvable, if
. If the transform is only known on a subset of the domain, this may no longer be true. Some of these so-called incomplete data problems are as follows (cf. Potential theory, inverse problems in). i) The exterior problem, i.e. recovering a function in the exterior of some ball from integrals over lines or planes outside that ball. This problem is uniquely solvable, if 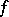 is decaying fast enough at infinity. ii) The interior problem, i.e. determining
is decaying fast enough at infinity. ii) The interior problem, i.e. determining 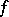 inside some ball from integrals inside that ball. A unique solution exists for odd dimension only. iii) The limited angle problem, where
inside some ball from integrals inside that ball. A unique solution exists for odd dimension only. iii) The limited angle problem, where 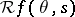 is given only for
is given only for 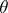 in a subset of a half-sphere. Uniqueness holds as long as the set of directions is such that no non-trivial homogeneous polynomial vanishes on it. iv) Problems with a finite number of directions. The solution is non-unique to a very large extent. In fact, for any finite set of directions and any function
in a subset of a half-sphere. Uniqueness holds as long as the set of directions is such that no non-trivial homogeneous polynomial vanishes on it. iv) Problems with a finite number of directions. The solution is non-unique to a very large extent. In fact, for any finite set of directions and any function 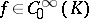 , with
, with 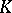 a compact set in
a compact set in 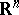 , and any compact set
, and any compact set 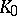 in the interior of
in the interior of 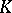 , one can find a function
, one can find a function  which coincides with
which coincides with  on
on 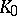 and for which all the line integrals along the given directions are identically zero, see [a20]. Such (highly oscillatory) functions
and for which all the line integrals along the given directions are identically zero, see [a20]. Such (highly oscillatory) functions 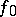 , sometimes called "ghostghosts" , form the null-space of the transform for finitely many projections. To resolve this indeterminacy one has to put restrictions on the variation of the function
, sometimes called "ghostghosts" , form the null-space of the transform for finitely many projections. To resolve this indeterminacy one has to put restrictions on the variation of the function  . On the other hand, by increasing the number of projections, arbitrarily good approximations to the unknown function can be found.
. On the other hand, by increasing the number of projections, arbitrarily good approximations to the unknown function can be found.
Reconstruction algorithms.
The identity 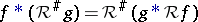 , where
, where  denotes convolution (cf. Convolution of functions), is the basis of filtered backprojection. One chooses
denotes convolution (cf. Convolution of functions), is the basis of filtered backprojection. One chooses 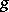 in such a way that
in such a way that 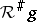 is a low-pass filter approaching a delta-distribution, so that
is a low-pass filter approaching a delta-distribution, so that 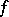 is recovered by a convolution followed by backprojection (both operations properly discretized). Variants exist where the backprojection is performed before the filtering procedure. The central slice theorem is the basis of Fourier reconstruction. Here the projections
is recovered by a convolution followed by backprojection (both operations properly discretized). Variants exist where the backprojection is performed before the filtering procedure. The central slice theorem is the basis of Fourier reconstruction. Here the projections  are Fourier transformed with respect to the last variable, which is allowed by the inverse
are Fourier transformed with respect to the last variable, which is allowed by the inverse  -dimensional Fourier transform. To make use of the fast Fourier transform (cf. Fourier transform, discrete), one first has to interpolate from a polar grid to a Cartesian grid. Analytic series expansion methods are based upon discretization of the inversion formulas for the individual expansion coefficients of the unknown function. Algebraic reconstruction techniques use iterative methods from numerical linear algebra (cf. Iteration methods) applied to the system of linear equations resulting from a full discretization of Radon's integral equation.
-dimensional Fourier transform. To make use of the fast Fourier transform (cf. Fourier transform, discrete), one first has to interpolate from a polar grid to a Cartesian grid. Analytic series expansion methods are based upon discretization of the inversion formulas for the individual expansion coefficients of the unknown function. Algebraic reconstruction techniques use iterative methods from numerical linear algebra (cf. Iteration methods) applied to the system of linear equations resulting from a full discretization of Radon's integral equation.
Problems in computerized tomography are ill-posed (cf. Ill-posed problems). There may be no solution if the data are not in the range of the transform, or the solution may be non-unique, problems which can be overcome by using the concept of generalized inverses. Moreover, the operator which maps the transform to the solution may not be continuous. In that case one uses regularization procedures, such as Tikhonov–Phillips regularization, iterative methods with appropriate stopping criteria or truncated singular value decompositions [a4], [a15]. Limited data problems in particular are severely ill-posed. In this context one also may apply various statistical estimation procedures with or without the use of a-priori information, often resulting in iterative algorithms.
Generalizations.
Generalized Radon transforms can be defined, where the integration is over subspaces or, more generally, over manifolds [a6], [a8], [a12]. A related problem for such spaces is the orbital integral problem, i.e. recovering a function from its integrals over (generalized) spheres. In acoustic or diffraction tomography, which is a special inverse scattering problem, 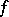 can be recovered from data on semi-circles through the origin (see [a14], [a18], [a21]). Another variant is the attenuated Radon transform, which differs from the standard Radon transform by the presence of an exponential weighting factor under the integral sign. For the use of the Radon transform in the area of partial differential equations, see [a8], [a13]. One more generalization of Radon's problem is the reconstruction of a measure from its projections, i.e. from lower-dimensional measures induced by some measurable mapping. For the Radon transform in the complex domain, see [a6]. Finite Radon transforms have also been studied.
can be recovered from data on semi-circles through the origin (see [a14], [a18], [a21]). Another variant is the attenuated Radon transform, which differs from the standard Radon transform by the presence of an exponential weighting factor under the integral sign. For the use of the Radon transform in the area of partial differential equations, see [a8], [a13]. One more generalization of Radon's problem is the reconstruction of a measure from its projections, i.e. from lower-dimensional measures induced by some measurable mapping. For the Radon transform in the complex domain, see [a6]. Finite Radon transforms have also been studied.
Applications.
Tomography is a widely used method to reconstruct cross-sections of the interior structure of an object without having to cut or damage the object. In this context one usually speaks of computerized (computed, computer assisted) tomography, since for actually performing the reconstructions in practice one needs to use a digital computer. Through the interaction of a physical agens or "probe" — varying from 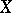 -rays, gamma rays, visible light, electrons, or neutrons to ultrasound waves or nuclear magnetic resonance signals — with the object, one obtains line or plane integrals of the internal distribution to be reconstructed. One of the most prominent examples of computerized tomography occurs in diagnostic medicine, where the method is used to produce images of the interior of human organs. In industry the method is used for non-destructive testing. Other applications arise in radio astronomy, radar theory, electron microscopy, aerodynamics, geophysics, and oceanography, see [a3], [a10], [a14] for references. Finite Radon transforms are used in various areas such as the theory of error-correcting codes and lattice theory.
-rays, gamma rays, visible light, electrons, or neutrons to ultrasound waves or nuclear magnetic resonance signals — with the object, one obtains line or plane integrals of the internal distribution to be reconstructed. One of the most prominent examples of computerized tomography occurs in diagnostic medicine, where the method is used to produce images of the interior of human organs. In industry the method is used for non-destructive testing. Other applications arise in radio astronomy, radar theory, electron microscopy, aerodynamics, geophysics, and oceanography, see [a3], [a10], [a14] for references. Finite Radon transforms are used in various areas such as the theory of error-correcting codes and lattice theory.
References
| [a1] | R.L. Bryant (ed.) V. Guillemin (ed.) S. Helgason (ed.) R.O. Wells jr. (ed.) , Integral geometry , Amer. Math. Soc. (1987) |
| [a2] | Y. Censor, T. Elfving, G.T. (eds.) Herman, "Linear algebra in image reconstruction from projections" Lin. Alg. Appl. , 130 (1990) pp. 1–305 (special issue) |
| [a3] | S.R. Deans, "The Radon transform and some of its applications" , Wiley (1983) |
| [a4] | H.W. Engl (ed.) C.W. Groetsch (ed.) , Inverse and ill-posed problems , Acad. Press (1987) |
| [a5] | P. Funk, "Über Flächen mit lauter geschlossenen geodätischen Linien" Math. Ann. , 74 (1913) pp. 287–300 |
| [a6] | I.M. Gel'fand, M.I. Graev, N.Ya. Vilenkin, "Generalized functions" , 5. Integral geometry and representation theory , Acad. Press (1966) (Translated from Russian) |
| [a7a] | A.B. Goncharev, "Integral geometry and three-dimensional reconstruction of randomly oriented identical particles from their electron microphotos" Acta Appl. Math. , 11 (1988) pp. 199–211 |
| [a7b] | A.B. Goncharev, "Methods of integral geometry and recovering a function with compact support from its projections in unknown directions" Acta Appl. Math. , 11 (1988) pp. 213–222 |
| [a8] | S. Helgason, "The Radon transform" , Birkhäuser (1980) |
| [a9] | S. Helgason, "Groups and geometric analysis" , Acad. Press (1984) pp. Chapt. II, Sect. 4 |
| [a10] | G.T. Herman, "Image reconstruction from projections, the fundamentals of computerized tomography" , Acad. Press (1980) |
| [a11] | G.T. Herman (ed.) F. Natterer (ed.) , Mathematical aspects of computerized tomography, Proc. Oberwolfach, February 10–16, 1980 , Springer (1981) |
| [a12] | F. John, "Bestimmung einer Funktion aus ihren Integralen über gewisse Mannigfaltigkeiten" Math. Ann. , 109 (1934) pp. 488–520 |
| [a13] | F. John, "Plane waves and spherical means applied to partial differential equations" , Interscience (1955) |
| [a14] | A.C. Kak, A.M. Slaney, "Principles of computerized tomography imaging" , IEEE (1988) |
| [a15] | A.K. Louis, "Inverse und schlecht gestellte Probleme" , Teubner (1989) |
| [a16] | F. Natterer, "The mathematics of computerized tomography" , Teubner & Wiley (1986) |
| [a17] | J. Radon, "Über die Bestimmung von Funktionen durch ihre Integralwerte längs gewisser Mannigfaltigkeiten" Ber. Sächs. Akad. Wissenschaft. Leipzig Math. Phys. Kl. , 69 (1917) pp. 262–267 |
| [a18] | P.C. Sabatier, "Basic methods of tomography and inverse problems" , A. Hilger (1987) |
| [a19] | L.A. Shepp (ed.) , Computed tomography , Proc. Symp. Appl. Math. , 27 , Amer. Math. Soc. (1983) |
| [a20] | K.T. Smith, D.C. Solmon, S.L. Wagner, "Practical and mathematical aspects of the problem of reconstructing a function from radiographs" Bull. Amer. Math. Soc. (N.S.) , 83 (1977) pp. 1227–1270 |
| [a21] | H. Stark, "Image recovery: theory and application" , Acad. Press (1987) |
Tomography. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tomography&oldid=49470