Difference between revisions of "Symplectic space"
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
Ulf Rehmann (talk | contribs) m (Undo revision 48934 by Ulf Rehmann (talk)) Tag: Undo |
||
| Line 1: | Line 1: | ||
| − | < | + | An odd-dimensional projective space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918501.png" /> over a field <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918502.png" /> endowed with an involutory relation which is a null polarity; it is denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918503.png" />. |
| − | s0918501.png | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | + | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918504.png" />. The absolute null polarity in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918505.png" /> can always be written in the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918506.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918507.png" /> is a skew-symmetric matrix <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918508.png" />. In vector form, the absolute null polarity can be written in the form <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s0918509.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185010.png" /> is a skew-symmetric operator whose matrix, in a suitable basis, reduces to the form | |
| − | |||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185011.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
In this case the absolute null polarity takes the canonical form | In this case the absolute null polarity takes the canonical form | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185012.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
The absolute null polarity induces a bilinear form, written in canonical form as follows: | The absolute null polarity induces a bilinear form, written in canonical form as follows: | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185013.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | Collineations of | + | Collineations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185014.png" /> that commute with its null polarity are called symplectic transformations; the operators defining these collineations are called symplectic. The above canonical form of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185015.png" /> defines the square matrix of order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185016.png" /> of a symplectic operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185017.png" /> whose elements satisfy the conditions |
| − | that commute with its null polarity are called symplectic transformations; the operators defining these collineations are called symplectic. The above canonical form of | ||
| − | defines the square matrix of order | ||
| − | of a symplectic operator | ||
| − | whose elements satisfy the conditions | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185018.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | where | + | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185019.png" /> is the Kronecker delta. Such a matrix is called symplectic; its determinant is equal to one. The symplectic transformations form a group, which is a Lie group. |
| − | is the Kronecker delta. Such a matrix is called symplectic; its determinant is equal to one. The symplectic transformations form a group, which is a Lie group. | ||
| − | Every point of the space | + | Every point of the space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185020.png" /> lies in its polar hyperplane with respect to the absolute null polarity. One can also define polar subspaces in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185021.png" />. The manifold of self-polar <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185022.png" />-spaces of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185023.png" /> is called its absolute linear complex. In this context, a symplectic group is also called a (linear) complex group. |
| − | lies in its polar hyperplane with respect to the absolute null polarity. One can also define polar subspaces in | ||
| − | The manifold of self-polar | ||
| − | spaces of | ||
| − | is called its absolute linear complex. In this context, a symplectic group is also called a (linear) complex group. | ||
| − | Every pair of straight lines, and their polar | + | Every pair of straight lines, and their polar <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185024.png" />-spaces in the null polarity, define a unique symplectic invariant in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185025.png" /> with respect to the group of symplectic transformations of this space. Through every point of each line there passes a transversal of this line and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185026.png" />-spaces, which defines a projective quadruple of points. This is the geometrical interpretation of a symplectic invariant, which asserts the equality of the cross ratios of these quadruples of points. |
| − | spaces in the null polarity, define a unique symplectic invariant in | ||
| − | with respect to the group of symplectic transformations of this space. Through every point of each line there passes a transversal of this line and | ||
| − | spaces, which defines a projective quadruple of points. This is the geometrical interpretation of a symplectic invariant, which asserts the equality of the cross ratios of these quadruples of points. | ||
| − | The symplectic | + | The symplectic <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185027.png" />-dimensional space admits an interpretation in hyperbolic space and this indicates, among other things, a connection between symplectic and hyperbolic spaces. Thus, the group of symplectic transformations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185028.png" /> is isomorphic to the group of motions of the hyperbolic space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185029.png" />. In this interpretation, the symplectic invariant is related to the distance between points in hyperbolic space. |
| − | dimensional space admits an interpretation in hyperbolic space and this indicates, among other things, a connection between symplectic and hyperbolic spaces. Thus, the group of symplectic transformations of | ||
| − | is isomorphic to the group of motions of the hyperbolic space | ||
| − | In this interpretation, the symplectic invariant is related to the distance between points in hyperbolic space. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> B.A. Rozenfel'd, "Non-Euclidean spaces" , Moscow (1969) (In Russian) {{MR|}} {{ZBL|0767.53035}} {{ZBL|0713.53012}} {{ZBL|0702.53009}} </TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> B.A. Rozenfel'd, "Non-Euclidean spaces" , Moscow (1969) (In Russian) {{MR|}} {{ZBL|0767.53035}} {{ZBL|0713.53012}} {{ZBL|0702.53009}} </TD></TR></table> | ||
| + | |||
| + | |||
====Comments==== | ====Comments==== | ||
| − | The notation | + | The notation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185030.png" /> for the symplectic geometry in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185031.png" /> is not usual. By <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185032.png" /> one denotes the symplectic group in the linear space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185033.png" /> provided with an alternating (i.e. skew-symmetric) bilinear form. The corresponding group of projectivities in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185034.png" /> is denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185035.png" />; this is the group referred to in the main article above, and it is called the projective symplectic group. |
| − | for the symplectic geometry in | ||
| − | is not usual. By | ||
| − | one denotes the symplectic group in the linear space | ||
| − | provided with an alternating (i.e. skew-symmetric) bilinear form. The corresponding group of projectivities in | ||
| − | is denoted by | ||
| − | this is the group referred to in the main article above, and it is called the projective symplectic group. | ||
| − | The polar subspaces, or isotropic subspaces, as they are also called, in a projective space with a null polarity form an example of what is called a polar geometry (cf. also [[Polar space|Polar space]]; see ). In J. Tits' theory of buildings, the symplectic spaces interpreted as polar geometries are buildings of type | + | The polar subspaces, or isotropic subspaces, as they are also called, in a projective space with a null polarity form an example of what is called a polar geometry (cf. also [[Polar space|Polar space]]; see ). In J. Tits' theory of buildings, the symplectic spaces interpreted as polar geometries are buildings of type <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185036.png" /> (see [[#References|[a2]]] and [[Tits building|Tits building]]). |
| − | see [[#References|[a2]]] and [[Tits building|Tits building]]). | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1a]</TD> <TD valign="top"> F.D. Veldkamp, "Polar geometry" ''Indag. Math.'' , '''21''' (1959) pp. 512–551 {{MR|0125472}} {{ZBL|0090.11902}} </TD></TR><TR><TD valign="top">[a1b]</TD> <TD valign="top"> F.D. Veldkamp, "Polar geometry" ''Indag. Math.'' , '''22''' (1960) pp. 207–212 {{MR|0158301}} {{MR|0125472}} {{ZBL|0094.15602}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J. Tits, "Buildings of spherical type and finite BN-pairs" , ''Lect. notes in math.'' , '''386''' , Springer (1974) {{MR|0470099}} {{ZBL|0295.20047}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> R. Baer, "Linear algebra and projective geometry" , Acad. Press (1952) {{MR|0052795}} {{ZBL|0049.38103}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1963) {{MR|}} {{ZBL|0221.20056}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> E. Artin, "Geometric algebra" , Interscience (1957) pp. Chapt. II {{MR|1529733}} {{MR|0082463}} {{ZBL|0077.02101}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> O.T. O'Meara, "Symplectic groups" , Amer. Math. Soc. (1978) {{MR|}} {{ZBL|0422.15014}} {{ZBL|0414.20037}} {{ZBL|0393.47029}} {{ZBL|0383.20001}} {{ZBL|0378.22015}} {{ZBL|0366.22014}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> H. Weyl, "The classical groups" , Princeton Univ. Press (1946) pp. 120 {{MR|1488158}} {{MR|0000255}} {{ZBL|1024.20502}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> H.S.M. Coxeter, "Non-Euclidean geometry" , Univ. Toronto Press (1965) pp. 69–70 {{MR|1628013}} {{MR|1531918}} {{MR|0087965}} {{MR|0006835}} {{ZBL|0909.51003}} {{ZBL|0077.13903}} {{ZBL|0060.32807}} {{ZBL|68.0322.02}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> V. Guillemin, S. Sternberg, "Symplectic techniques in physics" , Cambridge Univ. Press (1984) {{MR|0770935}} {{ZBL|0576.58012}} </TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> A.J. Hahn, O.T. O'Meara, "The classical groups and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185037.png" />-theory" , Springer (1989) {{MR|1007302}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> P. Libermann, C.-M. Marle, "Symplectic geometry and analytical mechanics" , Reidel (1987) (Translated from French) {{MR|0882548}} {{ZBL|0643.53002}} </TD></TR></table> | <table><TR><TD valign="top">[a1a]</TD> <TD valign="top"> F.D. Veldkamp, "Polar geometry" ''Indag. Math.'' , '''21''' (1959) pp. 512–551 {{MR|0125472}} {{ZBL|0090.11902}} </TD></TR><TR><TD valign="top">[a1b]</TD> <TD valign="top"> F.D. Veldkamp, "Polar geometry" ''Indag. Math.'' , '''22''' (1960) pp. 207–212 {{MR|0158301}} {{MR|0125472}} {{ZBL|0094.15602}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> J. Tits, "Buildings of spherical type and finite BN-pairs" , ''Lect. notes in math.'' , '''386''' , Springer (1974) {{MR|0470099}} {{ZBL|0295.20047}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> R. Baer, "Linear algebra and projective geometry" , Acad. Press (1952) {{MR|0052795}} {{ZBL|0049.38103}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1963) {{MR|}} {{ZBL|0221.20056}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> E. Artin, "Geometric algebra" , Interscience (1957) pp. Chapt. II {{MR|1529733}} {{MR|0082463}} {{ZBL|0077.02101}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> O.T. O'Meara, "Symplectic groups" , Amer. Math. Soc. (1978) {{MR|}} {{ZBL|0422.15014}} {{ZBL|0414.20037}} {{ZBL|0393.47029}} {{ZBL|0383.20001}} {{ZBL|0378.22015}} {{ZBL|0366.22014}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> H. Weyl, "The classical groups" , Princeton Univ. Press (1946) pp. 120 {{MR|1488158}} {{MR|0000255}} {{ZBL|1024.20502}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> H.S.M. Coxeter, "Non-Euclidean geometry" , Univ. Toronto Press (1965) pp. 69–70 {{MR|1628013}} {{MR|1531918}} {{MR|0087965}} {{MR|0006835}} {{ZBL|0909.51003}} {{ZBL|0077.13903}} {{ZBL|0060.32807}} {{ZBL|68.0322.02}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> V. Guillemin, S. Sternberg, "Symplectic techniques in physics" , Cambridge Univ. Press (1984) {{MR|0770935}} {{ZBL|0576.58012}} </TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> A.J. Hahn, O.T. O'Meara, "The classical groups and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s091/s091850/s09185037.png" />-theory" , Springer (1989) {{MR|1007302}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> P. Libermann, C.-M. Marle, "Symplectic geometry and analytical mechanics" , Reidel (1987) (Translated from French) {{MR|0882548}} {{ZBL|0643.53002}} </TD></TR></table> | ||
Revision as of 14:53, 7 June 2020
An odd-dimensional projective space  over a field
over a field  endowed with an involutory relation which is a null polarity; it is denoted by
endowed with an involutory relation which is a null polarity; it is denoted by 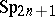 .
.
Let 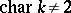 . The absolute null polarity in
. The absolute null polarity in  can always be written in the form
can always be written in the form  , where
, where 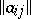 is a skew-symmetric matrix
is a skew-symmetric matrix 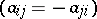 . In vector form, the absolute null polarity can be written in the form
. In vector form, the absolute null polarity can be written in the form 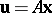 , where
, where  is a skew-symmetric operator whose matrix, in a suitable basis, reduces to the form
is a skew-symmetric operator whose matrix, in a suitable basis, reduces to the form
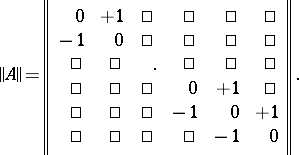 |
In this case the absolute null polarity takes the canonical form
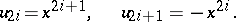 |
The absolute null polarity induces a bilinear form, written in canonical form as follows:
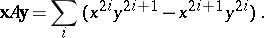 |
Collineations of  that commute with its null polarity are called symplectic transformations; the operators defining these collineations are called symplectic. The above canonical form of
that commute with its null polarity are called symplectic transformations; the operators defining these collineations are called symplectic. The above canonical form of 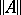 defines the square matrix of order
defines the square matrix of order  of a symplectic operator
of a symplectic operator  whose elements satisfy the conditions
whose elements satisfy the conditions
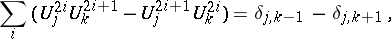 |
where 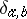 is the Kronecker delta. Such a matrix is called symplectic; its determinant is equal to one. The symplectic transformations form a group, which is a Lie group.
is the Kronecker delta. Such a matrix is called symplectic; its determinant is equal to one. The symplectic transformations form a group, which is a Lie group.
Every point of the space  lies in its polar hyperplane with respect to the absolute null polarity. One can also define polar subspaces in
lies in its polar hyperplane with respect to the absolute null polarity. One can also define polar subspaces in 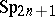 . The manifold of self-polar
. The manifold of self-polar  -spaces of
-spaces of  is called its absolute linear complex. In this context, a symplectic group is also called a (linear) complex group.
is called its absolute linear complex. In this context, a symplectic group is also called a (linear) complex group.
Every pair of straight lines, and their polar  -spaces in the null polarity, define a unique symplectic invariant in
-spaces in the null polarity, define a unique symplectic invariant in  with respect to the group of symplectic transformations of this space. Through every point of each line there passes a transversal of this line and
with respect to the group of symplectic transformations of this space. Through every point of each line there passes a transversal of this line and  -spaces, which defines a projective quadruple of points. This is the geometrical interpretation of a symplectic invariant, which asserts the equality of the cross ratios of these quadruples of points.
-spaces, which defines a projective quadruple of points. This is the geometrical interpretation of a symplectic invariant, which asserts the equality of the cross ratios of these quadruples of points.
The symplectic  -dimensional space admits an interpretation in hyperbolic space and this indicates, among other things, a connection between symplectic and hyperbolic spaces. Thus, the group of symplectic transformations of
-dimensional space admits an interpretation in hyperbolic space and this indicates, among other things, a connection between symplectic and hyperbolic spaces. Thus, the group of symplectic transformations of 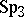 is isomorphic to the group of motions of the hyperbolic space
is isomorphic to the group of motions of the hyperbolic space 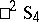 . In this interpretation, the symplectic invariant is related to the distance between points in hyperbolic space.
. In this interpretation, the symplectic invariant is related to the distance between points in hyperbolic space.
References
| [1] | B.A. Rozenfel'd, "Non-Euclidean spaces" , Moscow (1969) (In Russian) Zbl 0767.53035 Zbl 0713.53012 Zbl 0702.53009 |
Comments
The notation 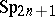 for the symplectic geometry in
for the symplectic geometry in 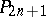 is not usual. By
is not usual. By 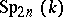 one denotes the symplectic group in the linear space
one denotes the symplectic group in the linear space 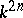 provided with an alternating (i.e. skew-symmetric) bilinear form. The corresponding group of projectivities in
provided with an alternating (i.e. skew-symmetric) bilinear form. The corresponding group of projectivities in 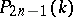 is denoted by
is denoted by 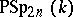 ; this is the group referred to in the main article above, and it is called the projective symplectic group.
; this is the group referred to in the main article above, and it is called the projective symplectic group.
The polar subspaces, or isotropic subspaces, as they are also called, in a projective space with a null polarity form an example of what is called a polar geometry (cf. also Polar space; see ). In J. Tits' theory of buildings, the symplectic spaces interpreted as polar geometries are buildings of type 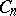 (see [a2] and Tits building).
(see [a2] and Tits building).
References
| [a1a] | F.D. Veldkamp, "Polar geometry" Indag. Math. , 21 (1959) pp. 512–551 MR0125472 Zbl 0090.11902 |
| [a1b] | F.D. Veldkamp, "Polar geometry" Indag. Math. , 22 (1960) pp. 207–212 MR0158301 MR0125472 Zbl 0094.15602 |
| [a2] | J. Tits, "Buildings of spherical type and finite BN-pairs" , Lect. notes in math. , 386 , Springer (1974) MR0470099 Zbl 0295.20047 |
| [a3] | R. Baer, "Linear algebra and projective geometry" , Acad. Press (1952) MR0052795 Zbl 0049.38103 |
| [a4] | J.A. Dieudonné, "La géométrie des groups classiques" , Springer (1963) Zbl 0221.20056 |
| [a5] | E. Artin, "Geometric algebra" , Interscience (1957) pp. Chapt. II MR1529733 MR0082463 Zbl 0077.02101 |
| [a6] | O.T. O'Meara, "Symplectic groups" , Amer. Math. Soc. (1978) Zbl 0422.15014 Zbl 0414.20037 Zbl 0393.47029 Zbl 0383.20001 Zbl 0378.22015 Zbl 0366.22014 |
| [a7] | H. Weyl, "The classical groups" , Princeton Univ. Press (1946) pp. 120 MR1488158 MR0000255 Zbl 1024.20502 |
| [a8] | H.S.M. Coxeter, "Non-Euclidean geometry" , Univ. Toronto Press (1965) pp. 69–70 MR1628013 MR1531918 MR0087965 MR0006835 Zbl 0909.51003 Zbl 0077.13903 Zbl 0060.32807 Zbl 68.0322.02 |
| [a9] | V. Guillemin, S. Sternberg, "Symplectic techniques in physics" , Cambridge Univ. Press (1984) MR0770935 Zbl 0576.58012 |
| [a10] | A.J. Hahn, O.T. O'Meara, "The classical groups and 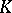 -theory" , Springer (1989) MR1007302 -theory" , Springer (1989) MR1007302 |
| [a11] | P. Libermann, C.-M. Marle, "Symplectic geometry and analytical mechanics" , Reidel (1987) (Translated from French) MR0882548 Zbl 0643.53002 |
Symplectic space. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Symplectic_space&oldid=49464