Difference between revisions of "Möbius function"
Ulf Rehmann (talk | contribs) m (moved Moebius function to Möbius function over redirect: accented title) |
|||
| Line 1: | Line 1: | ||
| + | |||
An [[Arithmetic function|arithmetic function]] of natural argument: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642801.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642802.png" /> if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642803.png" /> is divisible by the square of a prime number, otherwise <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642804.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642805.png" /> is the number of prime factors of the number <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642806.png" />. This function was introduced by A. Möbius in 1832. | An [[Arithmetic function|arithmetic function]] of natural argument: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642801.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642802.png" /> if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642803.png" /> is divisible by the square of a prime number, otherwise <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642804.png" />, where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642805.png" /> is the number of prime factors of the number <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m0642806.png" />. This function was introduced by A. Möbius in 1832. | ||
| Line 6: | Line 7: | ||
where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m06428010.png" /> is a constant. The fact that the mean value tends to zero as <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m06428011.png" /> implies an asymptotic law for the [[Distribution of prime numbers|distribution of prime numbers]] in the natural series. | where <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m06428010.png" /> is a constant. The fact that the mean value tends to zero as <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/m/m064/m064280/m06428011.png" /> implies an asymptotic law for the [[Distribution of prime numbers|distribution of prime numbers]] in the natural series. | ||
| + | |||
| + | The Möbius function is related to the Riemann zeros via the formula | ||
| + | |||
| + | \begin{equation} \sum_{n=1}^{\infty}\frac{\mu(n)}{\sqrt{n}} g \log n = \sum_t \frac{h(t)}{\zeta'(1/2+it)}+2\sum_{n=1}^\infty \frac{ (-1)^{n} (2\pi )^{2n}}{(2n)! \zeta(2n+1)}\int_{-\infty}^{\infty}g(x) e^{-x(2n+1/2)} \, dx,\end {equation} | ||
====References==== | ====References==== | ||
Revision as of 16:07, 21 June 2013
An arithmetic function of natural argument:  ,
, 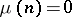 if
if  is divisible by the square of a prime number, otherwise
is divisible by the square of a prime number, otherwise  , where
, where  is the number of prime factors of the number
is the number of prime factors of the number  . This function was introduced by A. Möbius in 1832.
. This function was introduced by A. Möbius in 1832.
The Möbius function is a multiplicative arithmetic function;  if
if  . It is used in the study of other arithmetic functions; it appears in inversion formulas (see, e.g. Möbius series). The following estimate is known for the mean value of the Möbius function [2]:
. It is used in the study of other arithmetic functions; it appears in inversion formulas (see, e.g. Möbius series). The following estimate is known for the mean value of the Möbius function [2]:
 |
where  is a constant. The fact that the mean value tends to zero as
is a constant. The fact that the mean value tends to zero as 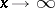 implies an asymptotic law for the distribution of prime numbers in the natural series.
implies an asymptotic law for the distribution of prime numbers in the natural series.
The Möbius function is related to the Riemann zeros via the formula
\begin{equation} \sum_{n=1}^{\infty}\frac{\mu(n)}{\sqrt{n}} g \log n = \sum_t \frac{h(t)}{\zeta'(1/2+it)}+2\sum_{n=1}^\infty \frac{ (-1)^{n} (2\pi )^{2n}}{(2n)! \zeta(2n+1)}\int_{-\infty}^{\infty}g(x) e^{-x(2n+1/2)} \, dx,\end {equation}
References
| [1] | I.M. Vinogradov, "Elements of number theory" , Dover, reprint (1954) (Translated from Russian) |
| [2] | A. Walfisz, "Weylsche Exponentialsummen in der neueren Zahlentheorie" , Deutsch. Verlag Wissenschaft. (1963) |
Comments
The multiplicative arithmetic functions form a group under the convolution product 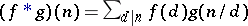 . The Möbius function is in fact the inverse of the constant multiplicative function
. The Möbius function is in fact the inverse of the constant multiplicative function 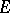 (defined by
(defined by 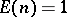 for all
for all  ) under this convolution product. From this there follows many "inversion formulas" , cf. e.g. Möbius series.
) under this convolution product. From this there follows many "inversion formulas" , cf. e.g. Möbius series.
References
| [a1] | G.H. Hardy, E.M. Wright, "An introduction to the theory of numbers" , Clarendon Press (1979) |
Möbius function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=M%C3%B6bius_function&oldid=29872