Zero

A (real or complex) number having the property that any number does not change if zero is added to it. It is denoted by the symbol . The product of any number with zero is zero:

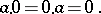If the product of two numbers is zero, then one of them is zero (that is, 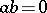implies or 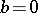). Division by zero is not defined. A direct generalization of this concept is that of the zero of an Abelian group.

The zero of an Abelian group 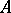(in additive notation) is an element, also denoted by , satisfying 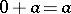for all 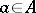. It is uniquely determined.

The zero of a ring (in particular, of a skew-field, i.e. division ring, or a field) is the zero of its additive group. The zero of a ring (like the number ) has the property of absorption under multiplication: 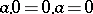. However, in an arbitrary ring the product of two non-zero elements may be zero. Such elements are called zero divisors (cf. Zero divisor). Fields, skew-fields and integral domains do not have zero divisors.

A left zero of a semi-group 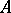(in multiplicative notation) is an element 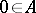such that 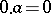for all . A right zero is defined by the dual property. If a semi-group has a two-sided zero (an element which is both a left and a right zero), then this element is unique. The zero of a ring is also the zero of its multiplicative semi-group.

The zero of a lattice is its minimal element, if this exists. A complete lattice always has a zero: the intersection of all elements.

A zero of an algebraic system is an element picked out by a nullary operation (see Algebraic operation; Algebraic system). In the majority of examples considered above the zero is unique in the given system and even forms a one-element subsystem.

A zero is also called a null element.

For a zero object of a category, see Null object of a category.

The set of zeros of a function taking values in an Abelian group (ring, field, skew-field) 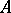is the collection of values of the variables 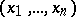for which .

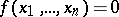Comments

A subset of a topological space 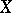is called a zero set if it is the set of zeros of some continuous real-valued function on 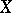. Zero sets are an object of study in algebraic geometry (zero sets of systems of polynomials) and local analytic geometry (zero sets of systems of holomorphic functions and mappings).

References

[a1]	N. Jacobson, "Basic algebra" , 1 , Freeman (1974) MR0356989 Zbl 0284.16001