Difference between revisions of "Canonical class"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 10: | Line 10: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) {{MR|0447223}} {{ZBL|0362.14001}} </TD></TR></table> |
| Line 18: | Line 18: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> S. Iitaka, "Algebraic geometry, an introduction to birational geometry of algebraic varieties" , Springer (1982) {{MR|}} {{ZBL|0491.14006}} </TD></TR></table> |
Revision as of 21:50, 30 March 2012
The class 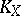 of divisors, with respect to linear equivalence on an algebraic variety
of divisors, with respect to linear equivalence on an algebraic variety 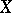 , which are divisors of differential forms
, which are divisors of differential forms 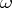 of maximal degree. If
of maximal degree. If 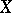 is a non-singular algebraic variety and
is a non-singular algebraic variety and 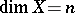 , then in local coordinates
, then in local coordinates  a form
a form 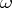 can be written as
can be written as
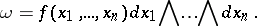 |
The divisor 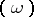 of
of  is locally equal to the divisor
is locally equal to the divisor 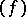 of this rational function
of this rational function 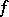 . This construction does not depend on the choice of local coordinates and gives the divisor
. This construction does not depend on the choice of local coordinates and gives the divisor 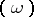 of
of 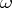 on all of
on all of 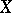 . Since for any other form
. Since for any other form 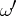 of the same degree as
of the same degree as 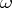 ,
, 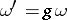 , it follows that
, it follows that 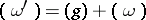 , and corresponding divisors are equivalent. The canonical class
, and corresponding divisors are equivalent. The canonical class 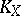 thus constructed is the first Chern class of the sheaf
thus constructed is the first Chern class of the sheaf 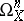 of regular differential forms of degree
of regular differential forms of degree  . Its numerical characteristics (degree, index, self-intersections, etc.) are effectively calculable invariants of the algebraic variety.
. Its numerical characteristics (degree, index, self-intersections, etc.) are effectively calculable invariants of the algebraic variety.
If 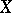 is a non-singular projective curve of genus
is a non-singular projective curve of genus 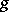 , then
, then 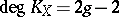 . For elliptic curves and, more generally, for Abelian varieties,
. For elliptic curves and, more generally, for Abelian varieties, 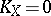 . If
. If 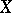 is a non-singular hypersurface of degree
is a non-singular hypersurface of degree  in projective space
in projective space 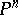 , then
, then 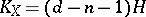 , where
, where 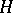 is a hyperplane section of it.
is a hyperplane section of it.
See also Canonical imbedding.
References
| [1] | I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) MR0447223 Zbl 0362.14001 |
Comments
References
| [a1] | S. Iitaka, "Algebraic geometry, an introduction to birational geometry of algebraic varieties" , Springer (1982) Zbl 0491.14006 |
Canonical class. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Canonical_class&oldid=23774