Difference between revisions of "Santaló formula"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Santaló formula to Santalo formula: ascii title) |
(No difference)
| |
Revision as of 18:54, 24 March 2012
A formula describing the Liouville measure on the unit tangent bundle of a Riemannian manifold in terms of the geodesic flow and the measure of a codimension-one submanifold (see [a5] and [a6], Chap. 19).
Let  be an
be an  -dimensional Riemannian manifold, let
-dimensional Riemannian manifold, let  be the unit tangent bundle of
be the unit tangent bundle of  , let
, let  be the Liouville measure on
be the Liouville measure on  , and let
, and let  be the geodesic flow. One way to define
be the geodesic flow. One way to define  is to start with the standard contact form
is to start with the standard contact form  (cf. Contact structure) and define
(cf. Contact structure) and define 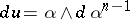 . Liouville's theorem says that
. Liouville's theorem says that 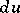 is invariant under the geodesic flow
is invariant under the geodesic flow  (since
(since 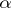 is). Locally,
is). Locally, 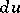 is just the product measure
is just the product measure 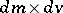 where
where 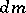 is the Riemannian volume form and
is the Riemannian volume form and 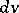 is the standard volume form on the unit
is the standard volume form on the unit  -sphere.
-sphere.
For any (locally defined) codimension-one submanifold 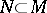 , let
, let 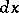 be the Riemannian volume element of the submanifold. Let
be the Riemannian volume element of the submanifold. Let 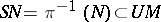 , and, for each
, and, for each  , let
, let  be a unit normal to
be a unit normal to 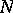 at
at  . Then there is a smooth mapping
. Then there is a smooth mapping 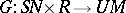 , given by
, given by  . Santaló's formula says:
. Santaló's formula says:
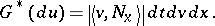 |
The formula is used to convert integrals over subsets 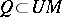 of the unit tangent bundle to iterated integrals, first over a fixed unit-speed geodesic (say parametrized on
of the unit tangent bundle to iterated integrals, first over a fixed unit-speed geodesic (say parametrized on 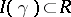 ) and then over the space
) and then over the space 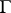 of geodesics which are parametrized by their intersections with a fixed codimension-one submanifold and endowed with the measure
of geodesics which are parametrized by their intersections with a fixed codimension-one submanifold and endowed with the measure 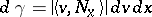 , i.e.
, i.e.
 |
One of the most important applications is to the study of Riemannian manifolds with smooth boundary. In this case 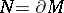 ,
, 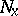 is the inwardly pointing unit normal vector and
is the inwardly pointing unit normal vector and  . For any
. For any 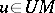 , set
, set 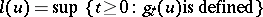 . Note that
. Note that 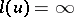 means that
means that 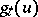 is defined for all
is defined for all 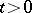 . Let
. Let 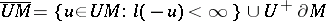 , i.e.
, i.e.  means
means 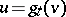 for some some
for some some  and some
and some 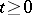 . In this setting, Santaló's formula takes the form:
. In this setting, Santaló's formula takes the form:
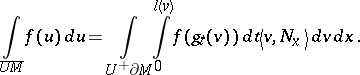 |
One immediate application, by simply putting 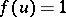 , is:
, is:
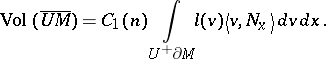 |
Since the Liouville measure is locally a product measure, in the special case 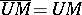 this says
this says 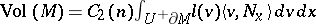 .
.
The formula is often used to prove isoperimetric and rigidity results. A sample of such applications can be found in the references. See [a1] for Santaló's formula for time-like geodesic flow on Lorentzian surfaces.
References
| [a1] | L. Andersson, M. Dahl, R. Howard, "Boundary and lens rigidity of Lorentzian surfaces" Trans. Amer. Math. Soc. , 348 : 6 (1996) pp. 2307–2329 |
| [a2] | C. Croke, "A sharp four-dimensional isoperimetric inequality" Comment. Math. Helv. , 59 : 2 (1984) pp. 187–192 |
| [a3] | C. Croke, "Some isoperimetric inequalities and eigenvalue estimates" Ann. Sci. École Norm. Sup. , 13 : 4 (1980) pp. 419–435 |
| [a4] | C. Croke, N. Dairbekov, V. Sharafutdinov, "Local boundary rigidity of a compact Riemannian manifold with curvature bounded above" Trans. Amer. Math. Soc. (to appear) |
| [a5] | L.A. Santaló, "Measure of sets of geodesics in a Riemannian space and applications to integral formulas in elliptic and hyperbolic spaces" Summa Brasil. Math. , 3 (1952) pp. 1–11 |
| [a6] | L.A. Santaló, "Integral geometry and geometric probability (With a foreword by Mark Kac)" , Encyclopedia Math. Appl. , 1 , Addison-Wesley (1976) |
Santaló formula. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Santal%C3%B3_formula&oldid=23001