Difference between revisions of "Fishburn-Shepp inequality"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Fishburn–Shepp inequality to Fishburn-Shepp inequality: ascii title) |
(No difference)
| |
Revision as of 18:52, 24 March 2012
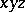 inequality
inequality
An inequality for linear extensions of a finite partially ordered set 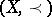 . Elements
. Elements 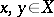 are incomparable if
are incomparable if  and neither
and neither 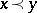 nor
nor  . Denote by
. Denote by 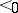 a generic linear order extension of
a generic linear order extension of 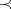 on
on 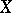 , let
, let 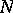 be the number of linear extensions
be the number of linear extensions 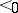 , and let
, and let 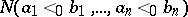 be the number of linear extensions in which
be the number of linear extensions in which  for
for 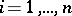 .
.
The Fishburn–Shepp inequality says that if  ,
, 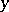 and
and  are mutually incomparable members of
are mutually incomparable members of 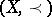 , then
, then
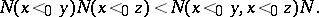 |
This was first proved for  in [a3], then for
in [a3], then for 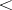 in [a2]. The Ahlswede–Daykin inequality [a1] plays a key role in the proof.
in [a2]. The Ahlswede–Daykin inequality [a1] plays a key role in the proof.
The inequality is also written as
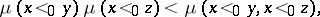 |
where 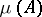 denotes the probability that a randomly chosen linear extension
denotes the probability that a randomly chosen linear extension 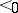 of
of 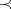 satisfies
satisfies 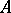 . When written as
. When written as 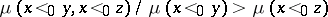 , one sees that the probability of
, one sees that the probability of 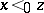 increases when it is true that also
increases when it is true that also 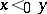 . Some plausible related inequalities are false [a3].
. Some plausible related inequalities are false [a3].
See also Correlation inequalities; FKG inequality; Holley inequality.
References
| [a1] | R. Ahlswede, D.E. Daykin, "An inequality for the weights of two families, their unions and intersections" Z. Wahrscheinlichkeitsth. verw. Gebiete , 43 (1978) pp. 183–185 |
| [a2] | P.C. Fishburn, "A correlational inequality for linear extensions of a poset" Order , 1 (1984) pp. 127–137 |
| [a3] | L.A. Shepp, "The XYZ conjecture and the FKG inequality" Ann. of Probab. , 10 (1982) pp. 824–827 |
Fishburn-Shepp inequality. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Fishburn-Shepp_inequality&oldid=22427