Banach-Stone theorem

Stone–Banach theorem

For a compact Hausdorff space , let 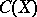be the Banach space of all continuous scalar-valued functions on 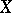, with the usual -norm:

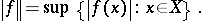If 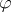is a surjective homeomorphism from a compact space onto a compact space 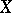and is a continuous and unimodular scalar-valued function on 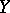, then

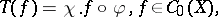(a1)

defines a linear isometry from onto . The Banach–Stone theorem asserts that any linear surjective isometry 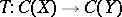is of the above form. Here, if is not necessarily compact, then 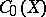is the space of continuous functions that vanish at infinity (i.e. the functions such that for all there is a compact set 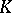such that for ). A unimodular function is one for which 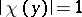for all .

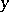The theorem was proved in the real case for compact metric spaces by S. Banach [a2]; M.H. Stone [a10] proved that the assumption of metrizability was superfluous. Subsequently the theorem was extended to spaces of both real- or complex-valued functions defined on a locally compact Hausdorff space.

The theorem has been extended further into several directions:

1) a Banach space 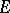has the Banach–Stone property if the Banach space 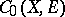of 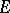-valued continuous functions is isometric with 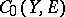if and only if and are homeomorphic; the class of Banach spaces with this property includes the strictly convex Banach spaces and the Banach spaces with strictly convex dual (see e.g. [a3], [a8]);

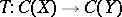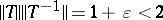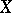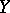2) if there is an isomorphism such that , then and are homeomorphic (and consequently 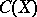and 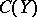are isometric) [a1], [a4], where is the best bound [a5];

3) the theorem holds for several classes of subspaces of the spaces 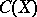, the most important one being the class of uniform algebras (cf. also Uniform algebra).

A joint approach to the last two extensions gave rise to the perturbation theory of uniform algebras. A Banach algebra 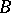is an -perturbation (or -metric perturbation) of a Banach algebra 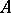if there is an isomorphism such that 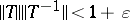. In such a situation the algebras , must share several important properties [a7], [a9]; if the algebra 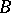is forced to be isometrically isomorphic with 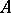, the algebra is called stable. Examples of stable uniform algebras include 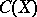, 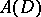and 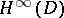, with the unit disc.

Isometries of several other classes of Banach spaces have been shown to follow the same general pattern, similar to (a1); one often refers to such results also as Banach–Stone theorems (for a given class of spaces; see e.g. [a6]).

References

[a1]	D. Amir, "On isomorphisms of continuous function spaces" Israel J. Math. , 3 (1965) pp. 205–210
[a2]	S. Banach, "Théorie des opérations linéaires" , PWN (1932)
[a3]	E. Behrends, "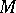-structure and the Banach–Stone theorem" , Lecture Notes in Mathematics , 736 , Springer (1979)
[a4]	M. Cambern, "On isomorphisms with small bound" Proc. Amer. Math. Soc. , 18 (1967) pp. 1062–1066
[a5]	H.B. Cohen, "A bound-two isomorphism between 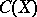Banach spaces" Proc. Amer. Math. Soc. , 50 (1975) pp. 215–217
[a6]	R.J. Fleming, J.E. Jamison, "Isometries on Banach spaces: a survey" , Analysis, Geometry and Groups: a Riemann Legacy Volume , Hadronic Press (1993) pp. 52–123
[a7]	K. Jarosz, "Perturbations of Banach algebras" , Lecture Notes in Mathematics , 1120 , Springer (1985)
[a8]	K. Jarosz, "Small isomorphisms of 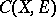spaces" Pacific J. Math. , 138 : 2 (1989) pp. 295–315
[a9]	R. Rochberg, "Deformation of uniform algebras on Riemann surfaces" Pacific J. Math. , 121 : 1 (1986) pp. 135–181
[a10]	M.H. Stone, "Applications of the theory of Boolean rings to general topology" Trans. Amer. Math. Soc. , 41 (1937) pp. 375–481