Lie algebra, reductive

A finite-dimensional Lie algebra over a field of characteristic 0 whose adjoint representation is completely reducible (cf. Adjoint representation of a Lie group; Representation of a Lie algebra). The property that a Lie algebra is reductive is equivalent to any of the following properties:

1) the radical 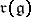of coincides with the centre ;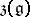

2) 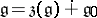, where is a semi-simple ideal of ;

3) 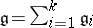, where the 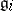are prime ideals;

4) admits a faithful completely-reducible finite-dimensional linear representation.

The property that a Lie algebra is reductive is preserved by both extension and restriction of the ground field .

An important class of reductive Lie algebras over are the compact Lie algebras (see Lie group, compact). A Lie group with a reductive Lie algebra is often called a reductive Lie group. A Lie algebra over is reductive if and only if it is isomorphic to the Lie algebra of a reductive algebraic group over .

A generalization of the concept of a reductive Lie algebra is the following. A subalgebra 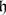of a finite-dimensional Lie algebra over is said to be reductive in if the adjoint representation 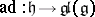is completely reducible. In this case is a reductive Lie algebra. If 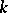is algebraically closed, then for a subalgebra of to be reductive it is necessary and sufficient that 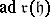consists of semi-simple linear transformations.

References

[1]	J.-P. Serre, "Lie algebras and Lie groups" , Benjamin (1965) (Translated from French) MR0218496 Zbl 0132.27803
[2]	J.-P. Serre, "Algèbres de Lie semi-simples complexes" , Benjamin (1966) MR0215886 Zbl 0144.02105
[3]	N. Bourbaki, "Elements of mathematics. Lie groups and Lie algebras" , Addison-Wesley (1975) (Translated from French) MR0682756 Zbl 0319.17002