Algebraic independence

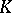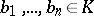A concept in the theory of field extensions (cf. Extension of a field). Let be some extension of a field . The elements are called algebraically independent over 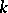if for each polynomial 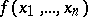with coefficients from 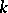which is not identically equal to zero, 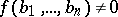. Otherwise, the elements 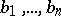are called algebraically dependent. An infinite set of elements is called algebraically independent if each one of its finite subsets is algebraically independent; otherwise it is called algebraically dependent. The definition of algebraic independence may be extended to the case where 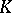is a ring, and a subring [1].

Algebraic independence of numbers.

Complex numbers are called algebraically independent if they are algebraically independent over the field of algebraic numbers, i.e. if for any polynomial 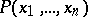with algebraic coefficients, not all of which are zero, the relationship is valid. Otherwise, are called algebraically dependent. The concept of algebraic independence of numbers is a generalization of the concept of transcendency of a number (the case 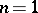). If several numbers are algebraically independent, each one of them is transcendental. It is usually very difficult to prove that given numbers are algebraically independent. The existing analytic methods of the theory of transcendental numbers give a solution of this problem for the values of certain classes of analytic functions. For instance, it was found that the values of the exponential function 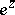, the arguments of which are algebraic and linearly independent over the field of rational numbers, are algebraically independent. A similar result was obtained for Bessel functions (cf. Siegel method). Several general theorems were also established concerning algebraic independence of the values in algebraic points of 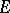-functions which satisfy linear differential equations with coefficients from the field of rational functions [2], [3]. Algebraic independence has been proved for numbers 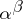and 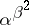, where 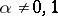is an algebraic number and 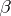is a cubic irrationality. A number of theorems have also been proved which deal with the algebraic non-expressibility of numbers; a concept close to that of algebraic independence.

It is possible to impart a quantitative facet to the qualitative concept of algebraic independence if the measure of algebraic independence (cf. Algebraic independence, measure of) of these numbers is considered. The analytic methods referred to above yield estimates from below for the measure of algebraic independence of certain classes of numbers. General theorems treating the estimation of the measure of algebraic independence of the values of 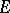-functions have been established [3].

References

[1]	S. Lang, "Algebra" , Addison-Wesley (1974) MR0799862 MR0783636 MR0760079 Zbl 0712.00001
[2]	N.I. Fel'dman, A.B. Shidlovskii, "The development and present state of the theory of transcendental numbers" Russian Math. Surveys , 22 : 3 (1967) pp. 1–79 Uspekhi Mat. Nauk , 22 : 3 (1967) pp. 3–81
[3]	A.B. Shidlovskii, "On arithmetic properties of values of analytic functions" Trudy Mat. Inst. Steklov. , 132 (1973) pp. 169–202 (In Russian) Zbl 0288.10012

Comments

The above-mentioned theorem on the algebraic independence of the values of 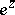is known as the Lindemann–Weierstrass theorem. Schanuel's conjecture, "transcendence degreetranscendence degree x1…xn,ex1…exn≥ n for any Q-linear independent x1…xnC" , is still (1986) unproved. An analogue of the Lindemann–Weierstrass theorem for values of the Weierstrass -function has been proved recently [a1], [a2] by using Gel'fond–Schneider-type arguments and zero estimates for polynomials on algebraic group varieties. Another method in algebraic independence is Mahler's method for values of functions satisfying functional equations [a3].

References

[a1]	G. Wüstholz, "Ueber das abelsche Analogon des Lindemannsche Satzes I" Inv. Math. , 72 (1983) pp. 363–388
[a2]	P. Philippon, "Variétés abéliennes et indépendence algébrique II" Inv. Math. , 72 (1983) pp. 389–405
[a3]	J. Loxton, A.J. van der Poorten, "Transcendence and algebraic independence by a method of Mahler" A. Baker (ed.) D. Masser (ed.) , Transcendence theory , Acad. Press (1977) pp. Chapt. 15 MR0476660 Zbl 0378.10020