Difference between revisions of "Poincaré-Dulac theorem"
Ulf Rehmann (talk | contribs) m (moved Poincare-Dulac theorem to Poincaré-Dulac theorem: accented title) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 40: | Line 40: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> H. Poincaré, , ''Oeuvres'' , '''1''' , Gauthier-Villars (1951) pp. UL-CXXXII {{MR|1787680}} {{MR|1401792}} {{MR|1401348}} {{MR|1401791}} {{MR|0392476}} {{MR|0392475}} {{MR|1554897}} {{ZBL|0894.01021}} {{ZBL|0894.01020}} {{ZBL|0894.01019}} {{ZBL|0072.24103}} {{ZBL|0059.00104}} {{ZBL|0059.00103}} {{ZBL|0049.44102}} {{ZBL|0041.37403}} {{ZBL|0041.37402}} {{ZBL|46.0004.01}} {{ZBL|36.0022.04}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> H. Dulac, "Recherches sur les points singuliers des equations différentielles" ''J. Ecole Polytechn. Ser. II'' , '''9''' (1904) pp. 1–25</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> K.-T. Chen, "Equivalence and decomposition of vectorfields about an elementary critical point" ''Amer. J. Math.'' , '''85''' (1963) pp. 693–722</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> F. Bruhat, "Travaux de Sternberg" ''Sém. Bourbaki'' , '''13''' (1960–1961) pp. Exp. 2187</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> S. Sternberg, "On the structure of a local homeomorphism" ''Amer. J. Math.'' , '''80''' (1958) pp. 623–631</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> M. Nagumo, K. Isé, "On the normal forms of differential equations in the neighbourhood of an equilibrium point" ''Osaka Math. J.'' , '''9''' (1957) pp. 221–234</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> P. Hartman, "On the local linearization of differential equations" ''Proc. Amer. Math. Soc.'' , '''14''' (1963) pp. 568–573</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> D. Arnal, M. Ben Ammar, G. Pinczon, "The Poincaré–Dulac theorem for nonlinear representations of nilpotent Lie algebras" ''Lett. Math. Phys.'' , '''8''' (1984) pp. 467–476</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> V.I. Arnol'd, "Geometrical methods in the theory of ordinary differential equations" , Springer (1977) pp. Chapt. V (Translated from Russian)</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> A.D. Bryuno, "Analytic forms of differential equations" ''Trans. Moscow Math. Soc.'' , '''25''' (1971) pp. 131–288 ''Trudy Moskov. Mat. Obshch.'' , '''25''' (1971) pp. 119–262</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> L.R. Hunt, R. Su, "Linear equivalents of nonlinear time-varying systems" , ''Internat. Symp. Math. Th. Networks and Systems Santa Monica, 1983'' , '''4''' , Western Periodicals (1981) pp. 119–123</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> B. Jakubczyk, W. Respondek, "On the linearization of control systems" ''Bull. Acad. Polon. Sci. Ser. Math. Astron. Phys.'' , '''28''' (1980) pp. 517–522</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> E.S. Livingston, D.L. Elliott, "Linearization of families of vectorfields" ''J. Diff. Equations'' , '''55''' (1984) pp. 289–299</TD></TR></table> |
Revision as of 17:00, 15 April 2012
Consider a (formal) differential equation in  -variables,
-variables,
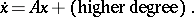 | (a1) |
A collection of eigen values 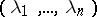 is said to be resonant if there is a relation of the form
is said to be resonant if there is a relation of the form
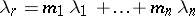 |
for some  , with
, with 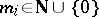 ,
, 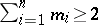 . The Poincaré theorem on canonical forms for formal differential equations says that if the eigen values of the matrix
. The Poincaré theorem on canonical forms for formal differential equations says that if the eigen values of the matrix 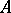 in (a1) are non-resonant, then there is a formal substitution of variables of the form
in (a1) are non-resonant, then there is a formal substitution of variables of the form 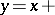 (higher degree) which makes (a1) take the form
(higher degree) which makes (a1) take the form
 | (a2) |
Part of the Poincaré–Dulac theorem says that there is for any equation of the form (a1) a formal change of variables 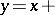 (higher degree) which transforms (a1) into an equation of the form
(higher degree) which transforms (a1) into an equation of the form
 | (a3) |
where  is a power series of which all monomials are resonant. Here a monomial
is a power series of which all monomials are resonant. Here a monomial 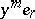 , where
, where 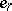 is the
is the  -th element of the standard basis, is called resonant if
-th element of the standard basis, is called resonant if  , where the
, where the 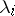 are the eigen values of
are the eigen values of 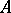 .
.
A point 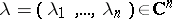 (a collection of eigen values) belongs to the Poincaré domain if 0 is not in the convex hull of the
(a collection of eigen values) belongs to the Poincaré domain if 0 is not in the convex hull of the 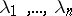 ; the complementary set of all
; the complementary set of all 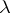 such that
such that  is in the convex hull of the
is in the convex hull of the 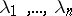 is called the Siegel domain. The second part of the Poincaré–Dulac theorem now says that if the right-hand side of (a1) is holomorphic and the eigen value set
is called the Siegel domain. The second part of the Poincaré–Dulac theorem now says that if the right-hand side of (a1) is holomorphic and the eigen value set  of
of  is in the Poincaré domain, then there is a holomorphic change of variables
is in the Poincaré domain, then there is a holomorphic change of variables 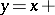 (higher degree) taking (a1) to a canonical form (a3), with
(higher degree) taking (a1) to a canonical form (a3), with 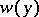 a polynomial in
a polynomial in  consisting of resonant monomials.
consisting of resonant monomials.
A point  is said to be of type
is said to be of type  , where
, where 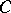 is a constant, if for all
is a constant, if for all 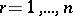 ,
,
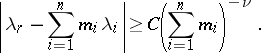 |
The Siegel theorem says that if the eigen values of 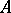 constitute a vector of type
constitute a vector of type 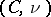 and (a1) is holomorphic, then in a neighbourhood of zero (a1) is holomorphically equivalent to (a2), i.e. there is a holomorphic change of coordinates taking (a1) to (a2).
and (a1) is holomorphic, then in a neighbourhood of zero (a1) is holomorphically equivalent to (a2), i.e. there is a holomorphic change of coordinates taking (a1) to (a2).
In the differentiable (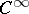 -) case there are related results, [a3]. Consider a
-) case there are related results, [a3]. Consider a  vector field
vector field 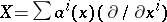 (or the corresponding autonomous system of differential equations
(or the corresponding autonomous system of differential equations  ). A critical point of
). A critical point of 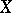 , i.e. a point
, i.e. a point 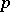 such that
such that  ,
,  , is called an elementary critical point
, is called an elementary critical point
 |
if the real part of each eigen value of the matrix 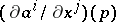 is non-zero. Let
is non-zero. Let  be a
be a 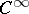 vector field with 0 as an elementary critical point. Then in a neighbourhood of zero,
vector field with 0 as an elementary critical point. Then in a neighbourhood of zero, 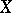 decomposes as a sum
decomposes as a sum 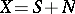 of
of 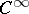 vector fields
vector fields 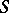 and
and 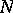 satisfying
satisfying 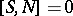 , and with respect to a suitable coordinate system
, and with respect to a suitable coordinate system 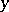 ,
,  is of the form
is of the form 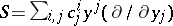 with the matrix
with the matrix 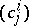 similar to a diagonal matrix, and the linear part of
similar to a diagonal matrix, and the linear part of 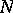 can be represented by a nilpotent matrix (Chen's decomposition theorem). This is a non-linear
can be represented by a nilpotent matrix (Chen's decomposition theorem). This is a non-linear 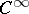 analogue of the decomposition of a matrix into commuting semi-simple and nilpotent parts, cf. Jordan decomposition. Now let
analogue of the decomposition of a matrix into commuting semi-simple and nilpotent parts, cf. Jordan decomposition. Now let 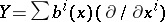 be a second vector field with 0 as an elementary critical point and let
be a second vector field with 0 as an elementary critical point and let  and
and 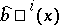 be the Taylor series of
be the Taylor series of  and
and 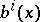 around 0. Then there exists a
around 0. Then there exists a 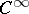 transformation around 0 which carries
transformation around 0 which carries 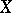 to
to 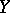 if and only if there exists a formal transformation which carries the formal vector field
if and only if there exists a formal transformation which carries the formal vector field 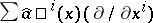 to the formal vector field
to the formal vector field  .
.
A 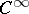 -linearization result due to S. Sternberg says the following [a4], [a5]. If the matrix of linear terms of the equations
-linearization result due to S. Sternberg says the following [a4], [a5]. If the matrix of linear terms of the equations 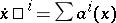 ,
, 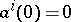 , is semi-simple and the set of eigen values of this matrix is non-resonant, then there is a
, is semi-simple and the set of eigen values of this matrix is non-resonant, then there is a 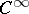 change of coordinates which linearizes the equations. For results in the
change of coordinates which linearizes the equations. For results in the  and
and 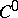 case cf. [a6], [a7].
case cf. [a6], [a7].
The Poincaré–Dulac theorem can be seen as a result on canonical forms of non-linear representations of the one-dimensional nilpotent Lie algebra  . In this form it generalizes to arbitrary nilpotent Lie algebras. Let
. In this form it generalizes to arbitrary nilpotent Lie algebras. Let 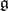 be a finite-dimensional nilpotent Lie algebra over
be a finite-dimensional nilpotent Lie algebra over 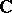 (cf. Lie algebra, nilpotent). Let
(cf. Lie algebra, nilpotent). Let  be the Lie algebra of formal vector fields
be the Lie algebra of formal vector fields 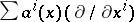 ,
, 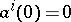 . A formal non-linear representation of
. A formal non-linear representation of 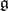 is a homomorphism
is a homomorphism 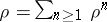 of
of 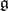 in
in 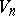 (where
(where 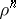 is the homogeneous part of degree
is the homogeneous part of degree  in
in 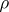 ). Such a representation is holomorphic if for each
). Such a representation is holomorphic if for each 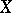 the series
the series 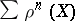 converges in some neighbourhood of
converges in some neighbourhood of  . Then
. Then 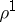 is a linear representation of
is a linear representation of 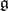 , called the linear part of
, called the linear part of 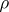 . A formal vector field
. A formal vector field  is called resonant with respect to a linear representation
is called resonant with respect to a linear representation 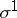 of
of 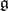 if
if 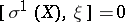 for all
for all  . The representation
. The representation 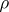 is normal if each
is normal if each 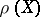 is resonant with respect to the semi-simple part (cf. Jordan decomposition) of the linear representation
is resonant with respect to the semi-simple part (cf. Jordan decomposition) of the linear representation 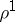 . The Poincaré–Dulac theorem for nilpotent Lie algebras, [a8], now says that
. The Poincaré–Dulac theorem for nilpotent Lie algebras, [a8], now says that 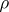 is a holomorphic non-linear representation of a nilpotent Lie algebra
is a holomorphic non-linear representation of a nilpotent Lie algebra 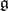 over
over 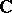 , and if
, and if 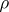 satisfies the Poincaré condition, then
satisfies the Poincaré condition, then  is holomorphically equivalent to a polynomial normal representation. In this setting the Poincaré condition (i.e., belonging to the Poincaré domain) takes the form that
is holomorphically equivalent to a polynomial normal representation. In this setting the Poincaré condition (i.e., belonging to the Poincaré domain) takes the form that  does not belong to the convex hull of the weights (cf. Weight of a representation of a Lie algebra) of the linear part
does not belong to the convex hull of the weights (cf. Weight of a representation of a Lie algebra) of the linear part 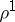 of
of 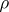 .
.
For rather complete accounts of the Poincaré–Dulac and Siegel theorems cf. [a9], [a10].
In control theory one studies equations  with a control parameter
with a control parameter  ; for instance,
; for instance, 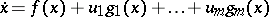 . This naturally leads to linearization problems for families of vector fields. In this setting more general notions of equivalence, involving, in particular, feedback laws
. This naturally leads to linearization problems for families of vector fields. In this setting more general notions of equivalence, involving, in particular, feedback laws  , are also natural (linearization by feedback). A selection of references is [a11]–[a13].
, are also natural (linearization by feedback). A selection of references is [a11]–[a13].
References
| [a1] | H. Poincaré, , Oeuvres , 1 , Gauthier-Villars (1951) pp. UL-CXXXII MR1787680 MR1401792 MR1401348 MR1401791 MR0392476 MR0392475 MR1554897 Zbl 0894.01021 Zbl 0894.01020 Zbl 0894.01019 Zbl 0072.24103 Zbl 0059.00104 Zbl 0059.00103 Zbl 0049.44102 Zbl 0041.37403 Zbl 0041.37402 Zbl 46.0004.01 Zbl 36.0022.04 |
| [a2] | H. Dulac, "Recherches sur les points singuliers des equations différentielles" J. Ecole Polytechn. Ser. II , 9 (1904) pp. 1–25 |
| [a3] | K.-T. Chen, "Equivalence and decomposition of vectorfields about an elementary critical point" Amer. J. Math. , 85 (1963) pp. 693–722 |
| [a4] | F. Bruhat, "Travaux de Sternberg" Sém. Bourbaki , 13 (1960–1961) pp. Exp. 2187 |
| [a5] | S. Sternberg, "On the structure of a local homeomorphism" Amer. J. Math. , 80 (1958) pp. 623–631 |
| [a6] | M. Nagumo, K. Isé, "On the normal forms of differential equations in the neighbourhood of an equilibrium point" Osaka Math. J. , 9 (1957) pp. 221–234 |
| [a7] | P. Hartman, "On the local linearization of differential equations" Proc. Amer. Math. Soc. , 14 (1963) pp. 568–573 |
| [a8] | D. Arnal, M. Ben Ammar, G. Pinczon, "The Poincaré–Dulac theorem for nonlinear representations of nilpotent Lie algebras" Lett. Math. Phys. , 8 (1984) pp. 467–476 |
| [a9] | V.I. Arnol'd, "Geometrical methods in the theory of ordinary differential equations" , Springer (1977) pp. Chapt. V (Translated from Russian) |
| [a10] | A.D. Bryuno, "Analytic forms of differential equations" Trans. Moscow Math. Soc. , 25 (1971) pp. 131–288 Trudy Moskov. Mat. Obshch. , 25 (1971) pp. 119–262 |
| [a11] | L.R. Hunt, R. Su, "Linear equivalents of nonlinear time-varying systems" , Internat. Symp. Math. Th. Networks and Systems Santa Monica, 1983 , 4 , Western Periodicals (1981) pp. 119–123 |
| [a12] | B. Jakubczyk, W. Respondek, "On the linearization of control systems" Bull. Acad. Polon. Sci. Ser. Math. Astron. Phys. , 28 (1980) pp. 517–522 |
| [a13] | E.S. Livingston, D.L. Elliott, "Linearization of families of vectorfields" J. Diff. Equations , 55 (1984) pp. 289–299 |
Poincaré-Dulac theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Poincar%C3%A9-Dulac_theorem&oldid=23472