Difference between revisions of "Poincaré return map"
Ulf Rehmann (talk | contribs) m (moved Poincaré return map to Poincare return map: ascii title) |
Ulf Rehmann (talk | contribs) m (moved Poincare return map to Poincaré return map over redirect: accented title) |
(No difference)
| |
Revision as of 07:55, 26 March 2012
successor mapping, of a smooth or at least continuous flow (continuous-time dynamical system) 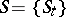 and a hypersurface
and a hypersurface 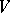 transversal to it
transversal to it
A mapping 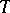 that assigns to a point
that assigns to a point 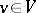 that point of the intersection of
that point of the intersection of 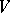 and the positive semi-trajectory of the flow starting at
and the positive semi-trajectory of the flow starting at  that comes first in time (it is defined for those
that comes first in time (it is defined for those  for which such an intersection exists). (The hypersurface
for which such an intersection exists). (The hypersurface 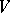 is called a section, an intersecting surface or a transversal.) If the dimension
is called a section, an intersecting surface or a transversal.) If the dimension 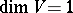 (so
(so  is a flow in the plane or on a two-dimensional surface,
is a flow in the plane or on a two-dimensional surface, 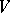 is called a contactless arc) and if
is called a contactless arc) and if 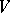 is parametrized by a numerical parameter
is parametrized by a numerical parameter  , then the shift of points of
, then the shift of points of 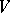 under the Poincaré return map is described by some numerical function in one variable (if
under the Poincaré return map is described by some numerical function in one variable (if  corresponds to the parameter value
corresponds to the parameter value  , then
, then 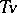 corresponds to the parameter value
corresponds to the parameter value 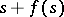 ), which is called the successor function. This mapping was used for the first time by H. Poincaré (see ), that is why it is called the Poincaré return map.
), which is called the successor function. This mapping was used for the first time by H. Poincaré (see ), that is why it is called the Poincaré return map.
If all semi-trajectories intersect 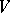 , then the Poincaré return map (in this case defined on the whole of
, then the Poincaré return map (in this case defined on the whole of 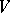 ) determines the behaviour of all trajectories of the flow to a considerable extent. However, such "global" sections are far from being common (in particular, a Hamiltonian system on a manifold of constant energy which does not pass through critical points of the Hamiltonian, i.e. through equilibrium positions (cf. Equilibrium position), does not have closed — as manifolds — global sections, see [3], Chapt. 8, Sect. 4.7).
) determines the behaviour of all trajectories of the flow to a considerable extent. However, such "global" sections are far from being common (in particular, a Hamiltonian system on a manifold of constant energy which does not pass through critical points of the Hamiltonian, i.e. through equilibrium positions (cf. Equilibrium position), does not have closed — as manifolds — global sections, see [3], Chapt. 8, Sect. 4.7).
For a non-autonomous system with a periodic right-hand side,
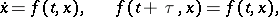 | (*) |
there exists an analogue of the Poincaré return map: to the point  corresponds the point
corresponds the point 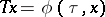 , where
, where 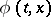 is the solution of (*) with initial value
is the solution of (*) with initial value 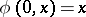 . This "map of the shift by a period" can be considered even formally as a Poincaré return map if (*) is considered as an autonomous system in "cylindrical" phase space. The map
. This "map of the shift by a period" can be considered even formally as a Poincaré return map if (*) is considered as an autonomous system in "cylindrical" phase space. The map 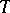 is defined everywhere if the solutions of (*) are defined for all
is defined everywhere if the solutions of (*) are defined for all 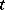 .
.
More often one has to deal with a "local" section — it is cut only by a part of the trajectories and often only part of the trajectories intersecting it return again to 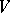 . As an example one can consider a small smooth "surface element" of codimension 1 intersecting transversally some periodic trajectory
. As an example one can consider a small smooth "surface element" of codimension 1 intersecting transversally some periodic trajectory 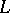 . In this case the Poincaré return map is defined near
. In this case the Poincaré return map is defined near 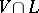 and characterizes the behaviour of the trajectories near
and characterizes the behaviour of the trajectories near  .
.
In the theory of foliations one can also introduce a Poincaré return map (see [2]), which is a generalization of the above example (and includes the Poincaré return map for ordinary differential equations in a complex domain).
References
| [1a] | H. Poincaré, "Mémoire sur les courbes définiés par une équation differentielle" J. de Math. , 7 (1881) pp. 375–422 |
| [1b] | H. Poincaré, "Mémoire sur les courbes définiés par une équation differentielle" J. de Math. , 8 (1882) pp. 251–296 |
| [1c] | H. Poincaré, "Mémoire sur les courbes définiés par une équation differentielle" J. de Math. , 1 (1885) pp. 167–244 |
| [1d] | H. Poincaré, "Mémoire sur les courbes définiés par une équation differentielle" J. de Math. , 2 (1886) pp. 151–217 |
| [2] | I. Tamura, "Topology of foliations" , Iwanami Shoten (1976) (In Japanese) |
| [3] | C. Godbillion, "Géométrie différentielle et mécanique analytique" , Hermann (1969) |
Comments
The return map 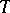 faithfully reflects many properties of the flow
faithfully reflects many properties of the flow  . For example, a point
. For example, a point 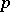 which is periodic under
which is periodic under 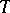 is necessarily periodic under
is necessarily periodic under 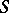 , with a possibly different period. Moreover, the
, with a possibly different period. Moreover, the 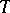 -orbit of
-orbit of 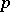 is asymptotically stable if and only if the
is asymptotically stable if and only if the 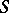 -orbit has the same property. In many ways the discrete-time dynamics of
-orbit has the same property. In many ways the discrete-time dynamics of 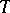 are easier to analyze than the continuous-time dynamics of the original flow
are easier to analyze than the continuous-time dynamics of the original flow 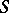 . Poincaré exploited these ideas in his study of homoclinic orbits in the three-body problem [a7].
. Poincaré exploited these ideas in his study of homoclinic orbits in the three-body problem [a7].
Every diffeomorphism 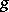 of a smooth manifold
of a smooth manifold 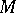 can be identified with a return map:
can be identified with a return map: 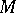 is obtained from
is obtained from 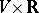 by the identifications
by the identifications  ; the flow
; the flow 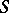 on
on 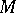 is induced by the flow
is induced by the flow 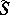 on
on 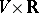 given by
given by 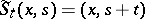 ; then
; then 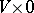 is a global section with return map
is a global section with return map  . By this construction many results proved for flows can be applied to diffeomorphisms.
. By this construction many results proved for flows can be applied to diffeomorphisms.
For the case when all semi-trajectories intersect 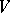 see [a8].
see [a8].
The "cylindrical" phase space mentioned above is defined as follows. Consider the autonomous system associated with (*), i.e.,
 | (a1) |
Identify the point 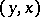 with
with  for every
for every  in the domain of
in the domain of 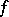 ; note that the latter is of the form
; note that the latter is of the form  , where
, where 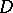 is a subset of
is a subset of 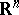 (when (*) is defined in
(when (*) is defined in 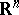 ). Then (a1) defines a dynamical system on the "cylinder"
). Then (a1) defines a dynamical system on the "cylinder"  , where
, where 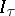 is the closed interval
is the closed interval 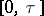 with the end-points identified, i.e.,
with the end-points identified, i.e.,  is a circle. The mapping
is a circle. The mapping 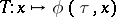 considered above now coincides with the Poincaré map of the system (a1) on
considered above now coincides with the Poincaré map of the system (a1) on 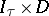 in the hypersurface
in the hypersurface 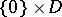 .
.
For the existence of global sections, see e.g. [a2], Sect. IV.2, and [a3]. In the context of more general transformation groups one speaks of "global sliceglobal slices" ; see e.g. [a1]. As to the existence of local sections in non-differentiable dynamical systems, see [a4], Sect. VI.2. In the theory of foliations one can recover the generalization of the Poincaré return map in the generators of the (leaf) holonomy groups. See e.g. [a6].
For applications of the Poincaré return map in the theory of differential equations (behaviour near a periodic orbit), see e.g. [a5] (so-called "Floquet theoryFloquet theory" ).
References
| [a1] | H. Abels, "Parallelizability of proper actions, global 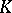 -slices and maximal compact subgroups" Math. Ann. , 212 (1974) pp. 1–19 -slices and maximal compact subgroups" Math. Ann. , 212 (1974) pp. 1–19 |
| [a2] | N.P. Bhatia, G.P. Szegö, "Stability theory of dynamical systems" , Springer (1970) |
| [a3] | O. Hajek, "Parallelizability revisited" Proc. Amer. Math. Soc. , 27 (1971) pp. 77–84 |
| [a4] | O. Hajek, "Dynamical systems in the plane" , Acad. Press (1968) |
| [a5] | P. Hartman, "Ordinary differential equations" , Birkhäuser (1982) |
| [a6] | G. Hector, U. Hirsch, "Introduction to the geometry of foliations" , Vieweg (1981) |
| [a7] | H. Poincaré, "Les méthodes nouvelles de la mécanique céleste" , I , Gauthier-Villars (1899) |
| [a8] | D. Fried, "The geometry of cross-sections to flows" Topology , 21 (1982) pp. 353–371 |
Poincaré return map. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Poincar%C3%A9_return_map&oldid=22939