Lindelöf summation method

A semi-continuous method for summing series of numbers and functions (cf. Summation methods), defined by the system of functions

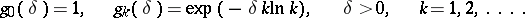The series

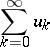is summable by the Lindelöf summation method to the sum if

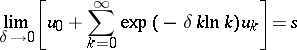and the series under the limit sign converges. The method was introduced by E. Lindelöf [1] for the summation of power series.

The Lindelöf summation method is regular (see Regular summation methods) and is used as a tool for the analytic continuation of functions. If is the principal branch of an analytic function, regular at the origin and representable by a series

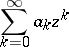for small , then this series is summable by the Lindelöf summation method to in the whole star of the function (cf. Star of a function element), and it is uniformly summable in every closed bounded domain contained in the interior of the star.

Of the summation methods determined by a transformation of a sequence into a sequence by semi-continuous matrices of type

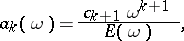where

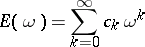is an entire function, Lindelöf considered the case when

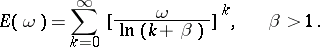A matrix 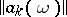constructed from an entire function of this kind is called a Lindelöf matrix.

References

[1]	E. Lindelöf, J. Math. , 9 (1903) pp. 213–221
[2]	E. Lindelöf, "Le calcul des résidus et ses applications à la théorie des fonctions" , Gauthier-Villars (1905)
[3]	G.H. Hardy, "Divergent series" , Clarendon Press (1949)
[4]	R.G. Cooke, "Infinite matrices and sequence spaces" , Macmillan (1950)