Difference between revisions of "Arithmetic group"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 4: | Line 4: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> A. Borel, "Ensembles fundamentaux pour les groups arithmétiques et formes automorphes" , Fac. Sci. Paris (1967)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Borel, Harish-Chandra, "Arithmetic subgroups of algebraic groups" ''Ann. of Math.'' , '''75''' (1962) pp. 485–535</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> , ''Arithmetic groups and discontinuous subgroups'' , ''Proc. Symp. Pure Math.'' , '''9''' , Amer. Math. Soc. (1966)</TD></TR></table> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> A. Borel, "Ensembles fundamentaux pour les groups arithmétiques et formes automorphes" , Fac. Sci. Paris (1967) {{MR|}} {{ZBL|}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Borel, Harish-Chandra, "Arithmetic subgroups of algebraic groups" ''Ann. of Math.'' , '''75''' (1962) pp. 485–535 {{MR|0147566}} {{ZBL|0107.14804}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> , ''Arithmetic groups and discontinuous subgroups'' , ''Proc. Symp. Pure Math.'' , '''9''' , Amer. Math. Soc. (1966) {{MR|}} {{ZBL|}} </TD></TR></table> |
| Line 14: | Line 14: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> A. Borel, "Arithmetic properties of linear algebraic groups" , ''Proc. Internat. Congress mathematicians (Stockholm, 1962)'' , Inst. Mittag-Leffler (1963) pp. 10–22</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> A. Borel, "Introduction aux groupes arithmétiques" , Hermann (1969)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J.E. Humphreys, "Arithmetic groups" , Springer (1980)</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> A. Borel, "Arithmetic properties of linear algebraic groups" , ''Proc. Internat. Congress mathematicians (Stockholm, 1962)'' , Inst. Mittag-Leffler (1963) pp. 10–22 {{MR|0175901}} {{ZBL|0134.16502}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> A. Borel, "Introduction aux groupes arithmétiques" , Hermann (1969) {{MR|0244260}} {{ZBL|0186.33202}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J.E. Humphreys, "Arithmetic groups" , Springer (1980) {{MR|0584623}} {{ZBL|0426.20029}} </TD></TR></table> |
Revision as of 10:02, 24 March 2012
A subgroup  of a linear algebraic group
of a linear algebraic group  defined over the field
defined over the field  of rational numbers, that satisfies the following condition: There exists a faithful rational representation
of rational numbers, that satisfies the following condition: There exists a faithful rational representation  defined over
defined over  (cf. Representation theory) such that
(cf. Representation theory) such that  is commensurable with
is commensurable with  , where
, where  is the ring of integers (two subgroups
is the ring of integers (two subgroups  and
and 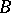 of a group
of a group  are called commensurable if
are called commensurable if  is of finite index in
is of finite index in  and in
and in  ). This condition is then also satisfied for any other faithful representation defined over
). This condition is then also satisfied for any other faithful representation defined over 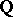 . More generally, an arithmetic group is a subgroup of an algebraic group
. More generally, an arithmetic group is a subgroup of an algebraic group  , defined over a global field
, defined over a global field  , that is commensurable with the group
, that is commensurable with the group  of
of  -points of
-points of 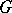 , where
, where  is the ring of integers of
is the ring of integers of 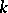 . An arithmetic group
. An arithmetic group 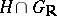 is a discrete subgroup of
is a discrete subgroup of  .
.
If 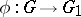 is a
is a  -epimorphism of algebraic groups, then the image
-epimorphism of algebraic groups, then the image  of any arithmetic group
of any arithmetic group  is an arithmetic group in
is an arithmetic group in 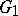 [1]. The name arithmetic group is sometimes also given to an abstract group that is isomorphic to an arithmetic subgroup of some algebraic group. Thus, if
[1]. The name arithmetic group is sometimes also given to an abstract group that is isomorphic to an arithmetic subgroup of some algebraic group. Thus, if  is an algebraic number field, the group
is an algebraic number field, the group  , where
, where 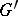 is obtained from
is obtained from 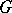 by restricting the field of definition from
by restricting the field of definition from 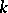 to
to  , is called an arithmetic group. In the theory of Lie groups the name arithmetic subgroups is also given to images of arithmetic subgroups of the group of real points of
, is called an arithmetic group. In the theory of Lie groups the name arithmetic subgroups is also given to images of arithmetic subgroups of the group of real points of  under the factorization of
under the factorization of 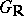 by compact normal subgroups.
by compact normal subgroups.
References
| [1] | A. Borel, "Ensembles fundamentaux pour les groups arithmétiques et formes automorphes" , Fac. Sci. Paris (1967) |
| [2] | A. Borel, Harish-Chandra, "Arithmetic subgroups of algebraic groups" Ann. of Math. , 75 (1962) pp. 485–535 MR0147566 Zbl 0107.14804 |
| [3] | , Arithmetic groups and discontinuous subgroups , Proc. Symp. Pure Math. , 9 , Amer. Math. Soc. (1966) |
Comments
Useful additional references are [a1]–[a3]. [a2] is an elementary introduction to the theory of arithmetic groups.
Conjectures of A. Selberg and I.I. Pyatetskii-Shapiro roughly state that for most semi-simple Lie groups discrete subgroups of finite co-volume are necessarily arithmetic. G.A. Margulis settled this question completely and, in particular, proved the conjectures in question. See Discrete subgroup for more detail.
References
| [a1] | A. Borel, "Arithmetic properties of linear algebraic groups" , Proc. Internat. Congress mathematicians (Stockholm, 1962) , Inst. Mittag-Leffler (1963) pp. 10–22 MR0175901 Zbl 0134.16502 |
| [a2] | A. Borel, "Introduction aux groupes arithmétiques" , Hermann (1969) MR0244260 Zbl 0186.33202 |
| [a3] | J.E. Humphreys, "Arithmetic groups" , Springer (1980) MR0584623 Zbl 0426.20029 |
Arithmetic group. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Arithmetic_group&oldid=18554