Parabolic subalgebra

A subalgebra of a finite-dimensional Lie algebra 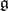over an algebraically closed field that contains a Borel subalgebra, i.e. a maximal solvable subalgebra of (cf. also Lie algebra, solvable). If is a finite-dimensional Lie algebra over an arbitrary field , then a subalgebra of it is also called a parabolic subalgebra if is a parabolic subalgebra of , where is the algebraic closure of the field 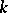. If 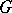is an irreducible linear algebraic group over a field of characteristic 0 and 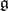is its Lie algebra, then a subalgebra 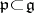is a parabolic subalgebra in if and only if it coincides with the Lie algebra of some parabolic subgroup of .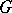

Examples of parabolic subalgebras in the Lie algebra of all square matrices of order over a field 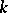are the subalgebras of type 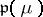( 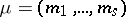is an arbitrary set of natural numbers with sum equal to ), where the algebra consists of all upper-triangular block-diagonal matrices with as diagonal blocks square matrices of orders .

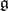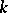Let be a reductive finite-dimensional Lie algebra (cf. Lie algebra, reductive) over a field of characteristic 0, let be a maximal diagonalizable subalgebra of over , let 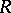be the system of -roots of 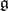relative to 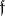(cf. Root system), let 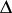be a basis (a set of simple roots) of 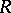, and let 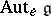be the group of elementary automorphisms of , i.e. the group generated by the automorphisms of the form 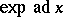, where is a nilpotent element of 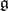. Then every parabolic subalgebra of the Lie algebra 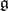is transformed by some automorphism from 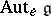into one of the standard parabolic subalgebras of the type

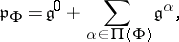where 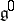is the centralizer of the subalgebra 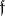in 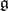, 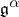is the root subspace of the Lie algebra 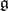corresponding to the root 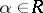, 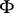is an arbitrary subset of the set 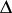, and 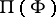is the set of those roots in 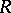whose decomposition into the sum of simple roots from 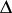contains elements of only with non-negative coefficients. 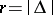In this way the number of classes of parabolic subalgebras conjugate with respect to turns out to be , where is the 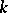-rank of the semi-simple Lie algebra 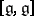. In addition, if , then 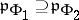. In particular, 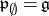, and 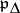is the minimal parabolic subalgebra of .

All non-reductive maximal subalgebras of finite-dimensional reductive Lie algebras over a field of characteristic 0 are parabolic subalgebras (see [2], [3], [5]).

References

[1]	N. Bourbaki, "Groupes et algèbres de Lie" , Hermann (1975) pp. Chapts. VII-VIII MR0682756 MR0573068 MR0271276 MR0240238 MR0132805 Zbl 0329.17002
[2]	F.I. Karpelevich, "On non-semi-simple maximal subalgebras of semi-simple Lie algebras" Dokl. Akad. Nauk USSR , 76 (1951) pp. 775–778 (In Russian)
[3]	V.V. Morozov, "Proof of the regularity theorem" Uspekhi Mat. Nauk , 11 (1956) pp. 191–194 (In Russian)
[4]	G.D. Mostow, "On maximal subgroups of real Lie groups" Ann. of Math. , 74 (1961) pp. 503–517 MR0142687 Zbl 0109.02301
[5]	A. Borel, J. Tits, "Eléments unipotents et sous-groupes paraboliques de groupes réductifs I" Invent. Math. , 12 (1971) pp. 95–104 MR0294349 Zbl 0238.20055