Implicit function (in algebraic geometry)

A function given by an algebraic equation. Let be a polynomial in and (with complex coefficients, say). Then the variety of zeros of this polynomial can be regarded as the graph of a correspondence . This correspondence, allowing for a certain impreciseness, is also called the function given implicitly by the equation . Generally speaking, is many-valued and not defined everywhere and so is not a function in the usual sense. There are two ways of turning this correspondence into a function. The first, which goes back to B. Riemann, consists in assuming that the domain of definition of the implicit function is not but the variety , which is a finite-sheeted covering of . This device leads to the highly important concept of a Riemann surface. In this approach the notion of an implicit function interlinks with that of an algebraic function.

The other approach consists in representing locally as the graph of a single-valued function. Various implicit-function theorems assert that there are open sets and for which is the graph of a smooth function (in one sense or another) (see Implicit function). However, the open sets and 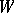are, as a rule, not open in the Zariski topology and have no meaning in algebraic geometry. Therefore, one modifies this method in the following manner. A formal germ (or branch) at a point of the implicit function given by the equation is defined as a formal power series such that . Quite generally, a power series satisfying a polynomial equation is said to be algebraic. An algebraic power series converges in a certain neighbourhood of .

Let be a local Noetherian ring with maximal ideal . An element of the completion of is said to be algebraic over if 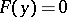for some polynomial . The set of elements of that are algebraic over forms a ring . The following version of the implicit-function theorem shows that there are sufficiently many algebraic functions. Let

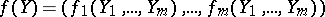be a collection of polynomials from and let be elements of the residue class field (the bar above a letter means reduction ) such that:

1) ;

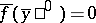2) .

Then there exist elements algebraic over such that and . In other words, is a Hensel ring.

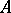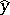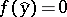Another result of this type is Artin's approximation theorem (see [2]). Let be a local ring that is the localization of an algebra of finite type over a field. Next, let be a system of polynomial equations with coefficients in (or in ) and let be a vector with coefficients in such that . Then there is a vector 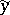with components in , arbitrarily close to 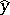and such that . There is also a version [3] of this theorem for systems of analytic equations.

References

[1]	M. Artin, "Algebraic spaces" , Yale Univ. Press (1971) MR0427316 MR0407012 Zbl 0232.14003 Zbl 0226.14001 Zbl 0216.05501
[2]	M. Artin, "Algebraic approximation of structures over complete local rings" Publ. Math. IHES , 36 (1969) pp. 23–58 MR0268188 Zbl 0181.48802
[3]	M. Artin, "On the solution of algebraic equations" Invent. Math. , 5 (1968) pp. 277–291