Difference between revisions of "Wedderburn-Mal'tsev theorem"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Wedderburn–Mal'tsev theorem to Wedderburn-Mal'tsev theorem: ascii title) |
(No difference)
| |
Revision as of 18:54, 24 March 2012
Let  be a finite-dimensional associative algebra (cf. Associative rings and algebras) over a field
be a finite-dimensional associative algebra (cf. Associative rings and algebras) over a field  with radical
with radical  , and let the quotient algebra
, and let the quotient algebra  be a separable algebra (for algebras over a field of characteristic zero this is always true). Then
be a separable algebra (for algebras over a field of characteristic zero this is always true). Then  can be decomposed (as a linear space) into a direct sum of the radical
can be decomposed (as a linear space) into a direct sum of the radical  and some semi-simple subalgebra
and some semi-simple subalgebra  :
:
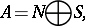 |
and if there exists another decomposition  , where
, where  is a semi-simple subalgebra, then there exists an automorphism
is a semi-simple subalgebra, then there exists an automorphism 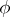 of the algebra
of the algebra  which maps
which maps  onto
onto 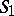 (the automorphism
(the automorphism  is inner, i.e. there exist elements
is inner, i.e. there exist elements  such that
such that 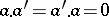 and
and 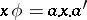 for all
for all 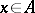 , where
, where 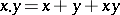 ). The existence of this decomposition was shown by J.H.M. Wedderburn [1] and the uniqueness, up to an automorphism of the semi-simple term, was proved by A.I. Mal'tsev [2]. This theorem, together with Wedderburn's theorem (cf. Associative rings and algebras) on the structure of semi-simple algebras constitutes the central part of the classical theory of finite-dimensional algebras.
). The existence of this decomposition was shown by J.H.M. Wedderburn [1] and the uniqueness, up to an automorphism of the semi-simple term, was proved by A.I. Mal'tsev [2]. This theorem, together with Wedderburn's theorem (cf. Associative rings and algebras) on the structure of semi-simple algebras constitutes the central part of the classical theory of finite-dimensional algebras.
References
| [1] | J.H.M. Wedderburn, "On hypercomplex numbers" Proc. London Math. Soc. (2) , 6 (1908) pp. 77–118 |
| [2] | A.I. Mal'tsev, "On the representation of an algebra as a direct sum of the radical and a semi-simple subalgebra" Dokl. Akad. Nauk SSSR , 36 : 1 (1942) pp. 42–45 (In Russian) |
| [3] | A.A. Albert, "Structure of algebras" , Amer. Math. Soc. (1939) |
| [4] | C.W. Curtis, I. Reiner, "Representation theory of finite groups and associative algebras" , Interscience (1962) |
Comments
A similar theorem holds for Lie algebras. Let  be a finite-dimensional Lie algebra over a field of characteristic zero with radical
be a finite-dimensional Lie algebra over a field of characteristic zero with radical  . Then there exists a semi-simple subalgebra
. Then there exists a semi-simple subalgebra  of
of  such that
such that 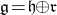 . Such a decomposition is called a Levi decomposition and
. Such a decomposition is called a Levi decomposition and  is called a Levi factor or Levi subalgebra. It is unique up to inner automorphisms.
is called a Levi factor or Levi subalgebra. It is unique up to inner automorphisms.
References
| [a1] | N. Jacobson, "Lie algebras" , Dover, reprint (1962) pp. 91ff ((also: Dover, reprint, 1979)) |
Wedderburn-Mal'tsev theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Wedderburn-Mal%27tsev_theorem&oldid=15934