Budan-Fourier theorem

The number of roots of an algebraic equation

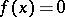comprised in an interval 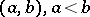, is equal to or is smaller, by an even number, than 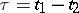, where 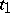is the number of changes in sign in the series of derivatives of the polynomial at the point , i.e. in the series

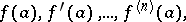while 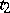is the number of changes in sign in this series at the point 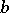. Each multiple root is counted according to its multiplicity. Established by F. Budan (1822) and J. Fourier (1820).

References

[1]	, Encyclopaedia of elementary mathematics , 2. Algebra , Moscow-Leningrad (1951) pp. 331 (In Russian)

Comments

An application of the Budan–Fourier theorem in numerical analysis may be found in [a1], where it is used in the interpolation by spline functions.

References

[a1]	C. de Boor, I.J. Schoenberg, "Cardinal interpolation and spline functions VIII. The Budan–Fourier theorem for splines and applications." K. Bohmer (ed.) G. Meinardus (ed.) W. Schempp (ed.) , Spline functions , Lect. notes in math. , 501 , Springer (1976)
[a2]	A.S. Householder, "Unique triangularization of a nonsymmetric matrix" J. Assoc. Comp. Mach. , 5 (1958) pp. 339–342