Difference between revisions of "Lamé function"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (moved Lamé function to Lame function: ascii title) |
(No difference)
| |
Revision as of 18:53, 24 March 2012
ellipsoidal harmonic function
A function of special form satisfying the Lamé equation. If the Lamé equation in algebraic form,
 | (*) |
 |
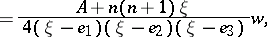where 
is natural number and 
, 
, 
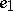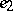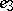, and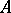 
are constants, has a solution of one of the following forms:
 |
 |
 |
 |
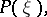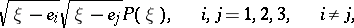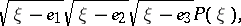where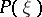 
is a polynomial with leading coefficient one, then this solution is called a Lamé function of degree 
of the first kind and the first, second, third, or fourth form, respectively.
For fixed even 
there are always values of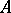 
(eigen values) such that there are 
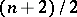Lamé functions of the first form and 
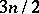of the third form, with polynomials 
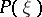of degree 
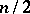and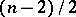 
, respectively. For fixed odd 
there are always values of 
such that there are 
Lamé functions of the second form and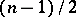 
of the fourth form, with polynomials 
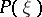of degree 
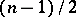and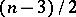 
, respectively. For a given natural number 
there are altogether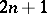 
linearly independent Lamé functions.
Solutions of equation (*) that are linearly independent with the Lamé functions of the first kind and are obtained by means of the Liouville–Ostrogradski formula are called Lamé functions of the second kind.
For references see Lamé equation.
Lamé function. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Lam%C3%A9_function&oldid=15271