Difference between revisions of "Affine variety"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 12: | Line 12: | ||
====Comments==== | ====Comments==== | ||
| − | Frequently the name | + | Frequently the name "varietyvariety" means a reduced and irreducible scheme of finite type over an algebraically closed field. |
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) {{MR|0447223}} {{ZBL|0362.14001}} </TD></TR></table> |
Revision as of 21:49, 30 March 2012
affine algebraic variety
A generalization of the concept of an affine algebraic set. An affine variety is a reduced affine scheme  of finite type over a field
of finite type over a field  , i.e.
, i.e. 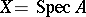 , where
, where 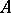 is a commutative
is a commutative 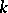 -algebra of finite type without nilpotent elements. The affine variety
-algebra of finite type without nilpotent elements. The affine variety 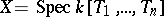 , where
, where  is the ring of polynomials over
is the ring of polynomials over 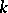 , is called affine space over
, is called affine space over 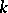 and is denoted by
and is denoted by  . An affine scheme is an affine variety if and only if it is isomorphic to a reduced closed subscheme of an affine space. Each system of generators
. An affine scheme is an affine variety if and only if it is isomorphic to a reduced closed subscheme of an affine space. Each system of generators  of a
of a 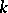 -algebra
-algebra 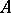 defines a surjective homomorphism
defines a surjective homomorphism 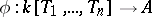 , defined by the formula
, defined by the formula 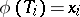 . Let
. Let 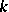 be the algebraic closure of
be the algebraic closure of 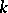 . The subset of the set
. The subset of the set 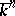 consisting of the common zeros of all the polynomials of the ideal
consisting of the common zeros of all the polynomials of the ideal 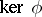 is an affine algebraic set over
is an affine algebraic set over 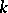 . The coordinate ring of such an affine algebraic set is isomorphic to the ring
. The coordinate ring of such an affine algebraic set is isomorphic to the ring 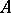 . Each affine algebraic set over
. Each affine algebraic set over  in turn defines an algebraic variety
in turn defines an algebraic variety 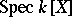 , where
, where 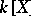 is the coordinate ring of
is the coordinate ring of 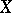 . The set of points of an affine variety is in a one-to-one correspondence with the irreducible subvarieties of the corresponding affine algebraic set.
. The set of points of an affine variety is in a one-to-one correspondence with the irreducible subvarieties of the corresponding affine algebraic set.
To each affine variety 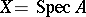 a functor on the category of
a functor on the category of 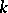 -algebras is assigned. It is defined by the correspondence:
-algebras is assigned. It is defined by the correspondence:
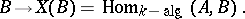 |
If 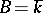 (respectively, if
(respectively, if 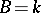 ), the elements of the set
), the elements of the set 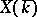 (respectively,
(respectively, 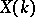 ) are called geometric (respectively, rational) points of
) are called geometric (respectively, rational) points of 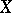 . The set
. The set 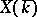 is in a bijective correspondence with the set of maximal ideals
is in a bijective correspondence with the set of maximal ideals 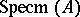 of the ring
of the ring 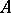 , and with the set of points of an algebraic set
, and with the set of points of an algebraic set  whose coordinate ring is isomorphic to
whose coordinate ring is isomorphic to 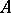 . The spectral topology in the space
. The spectral topology in the space  induces on the everywhere-dense subset
induces on the everywhere-dense subset 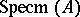 a topology which corresponds to the Zariski topology on
a topology which corresponds to the Zariski topology on 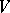 .
.
Comments
Frequently the name "varietyvariety" means a reduced and irreducible scheme of finite type over an algebraically closed field.
References
| [a1] | I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) MR0447223 Zbl 0362.14001 |
Affine variety. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Affine_variety&oldid=15053