Difference between revisions of "Frobenius endomorphism"
From Encyclopedia of Mathematics
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 4: | Line 4: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> R. Hartshorne, "Algebraic geometry" , Springer (1977) {{MR|0463157}} {{ZBL|0367.14001}} </TD></TR></table> |
| Line 12: | Line 12: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> P. Gabriel, "Etude infinitésimal des schémas en groupes" M. Demazure (ed.) A. Grothendieck (ed.) , ''SGA 3. Exp. VII'' , ''Lect. notes in math.'' , '''151''' , Springer (1970)</TD></TR></table> |
Revision as of 21:52, 30 March 2012
An endomorphism 
of a scheme 
over the finite field 
of 
elements such that 
is the identity mapping on 
, and the mapping of the structure sheaf 
is that of raising to the 
-th power. The Frobenius endomorphism is a purely-inseparable morphism and has zero differential. For an affine variety 
defined over 
, the Frobenius endomorphism 
takes the point 
to 
.
The number of geometric points of 
that are defined over 
is the same as the number of fixed points of 
, which enables one to use the Lefschetz formula to determine the number of such points.
References
| [1] | R. Hartshorne, "Algebraic geometry" , Springer (1977) MR0463157 Zbl 0367.14001 |
Comments
Here, 
is the set of 
-points of 
, i.e. the set of points of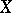 
that are defined over 
.
References
| [a1] | P. Gabriel, "Etude infinitésimal des schémas en groupes" M. Demazure (ed.) A. Grothendieck (ed.) , SGA 3. Exp. VII , Lect. notes in math. , 151 , Springer (1970) |
How to Cite This Entry:
Frobenius endomorphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Frobenius_endomorphism&oldid=12821
Frobenius endomorphism. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Frobenius_endomorphism&oldid=12821
This article was adapted from an original article by L.V. Kuz'min (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article