Difference between revisions of "Topology of manifolds"
Ulf Rehmann (talk | contribs) m (tex encoded by computer) |
Ulf Rehmann (talk | contribs) m (Undo revision 48993 by Ulf Rehmann (talk)) Tag: Undo |
||
| Line 1: | Line 1: | ||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
| − | |||
The branch of the theory of manifolds (cf. [[Manifold|Manifold]]) concerned with the study of relations between different types of manifolds. | The branch of the theory of manifolds (cf. [[Manifold|Manifold]]) concerned with the study of relations between different types of manifolds. | ||
The most important types of finite-dimensional manifolds and relations between them are illustrated in (1). | The most important types of finite-dimensional manifolds and relations between them are illustrated in (1). | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932301.png" /></td> <td valign="top" style="width:5%;text-align:right;">(1)</td></tr></table> | |
| − | Here | + | Here <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932302.png" /> is the category of differentiable (smooth) manifolds; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932303.png" /> is the category of piecewise-linear (combinatorial) manifolds; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932304.png" /> is the category of topological manifolds that are polyhedra; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932305.png" /> is the category of topological manifolds admitting a topological decomposition into handles; <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932306.png" /> is the category of Lipschitz manifolds (with Lipschitz transition mappings between local charts); <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932307.png" /> is the category of topological manifolds (Hausdorff and with a countable base); <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932308.png" /> is the category of polyhedral homology manifolds without boundary (polyhedra, the boundary of the star of each vertex of which has the homology of the sphere of corresponding dimension); <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t0932309.png" /> is the category of generalized manifolds (finite-dimensional absolute neighbourhood retracts <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323010.png" /> that are homology manifolds without boundary, i.e. with the property that for any point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323011.png" /> the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323012.png" /> is isomorphic to the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323013.png" />); <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323014.png" /> is the category of Poincaré spaces (finite-dimensional absolute neighbourhood retracts <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323015.png" /> for which there exists a number <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323016.png" /> and an element <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323017.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323018.png" /> when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323019.png" />, and the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323020.png" /> is an isomorphism for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323021.png" />); and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323022.png" /> is the category of Poincaré polyhedra (the subcategory of the preceding category consisting of polyhedra). |
| − | is the category of differentiable (smooth) manifolds; | ||
| − | is the category of piecewise-linear (combinatorial) manifolds; | ||
| − | is the category of topological manifolds that are polyhedra; | ||
| − | is the category of topological manifolds admitting a topological decomposition into handles; | ||
| − | is the category of Lipschitz manifolds (with Lipschitz transition mappings between local charts); | ||
| − | is the category of topological manifolds (Hausdorff and with a countable base); | ||
| − | is the category of polyhedral homology manifolds without boundary (polyhedra, the boundary of the star of each vertex of which has the homology of the sphere of corresponding dimension); | ||
| − | is the category of generalized manifolds (finite-dimensional absolute neighbourhood retracts | ||
| − | that are homology manifolds without boundary, i.e. with the property that for any point | ||
| − | the group | ||
| − | is isomorphic to the group | ||
| − | |||
| − | is the category of Poincaré spaces (finite-dimensional absolute neighbourhood retracts | ||
| − | for which there exists a number | ||
| − | and an element | ||
| − | such that | ||
| − | when | ||
| − | and the mapping | ||
| − | is an isomorphism for all | ||
| − | and | ||
| − | is the category of Poincaré polyhedra (the subcategory of the preceding category consisting of polyhedra). | ||
| − | The arrows of (1), apart from the 3 lower ones and the arrows | + | The arrows of (1), apart from the 3 lower ones and the arrows <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323023.png" />, denote functors with the structure of forgetting functors. The arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323024.png" /> expresses Whitehead's theorem on the triangulability of smooth manifolds. In dimensions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323025.png" /> this arrow is reversible (an arbitrary <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323026.png" />-manifold is smoothable) but in dimensions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323027.png" /> there are non-smoothable <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323028.png" />-manifolds and even <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323029.png" />-manifolds that are homotopy inequivalent to any smooth manifold. The imbedding <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323030.png" /> is also irreversible in the same strong sense (there exist polyhedral manifolds of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323031.png" /> that are homotopy inequivalent to any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323032.png" />-manifold). Here already for the sphere <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323033.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323034.png" />, there exist triangulations in which it is not a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323035.png" />-manifold. |
| − | denote functors with the structure of forgetting functors. The arrow | ||
| − | expresses Whitehead's theorem on the triangulability of smooth manifolds. In dimensions | ||
| − | this arrow is reversible (an arbitrary | ||
| − | manifold is smoothable) but in dimensions | ||
| − | there are non-smoothable | ||
| − | manifolds and even | ||
| − | manifolds that are homotopy inequivalent to any smooth manifold. The imbedding | ||
| − | is also irreversible in the same strong sense (there exist polyhedral manifolds of dimension | ||
| − | that are homotopy inequivalent to any | ||
| − | manifold). Here already for the sphere | ||
| − | |||
| − | there exist triangulations in which it is not a | ||
| − | manifold. | ||
| − | The arrow | + | The arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323036.png" /> expresses the fact that every <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323037.png" />-manifold has a handle decomposition. |
| − | expresses the fact that every | ||
| − | manifold has a handle decomposition. | ||
| − | The arrow | + | The arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323038.png" /> expresses the theorem on the existence of a Lipschitz structure on an arbitrary <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323039.png" />-manifold. |
| − | expresses the theorem on the existence of a Lipschitz structure on an arbitrary | ||
| − | manifold. | ||
| − | The arrow | + | The arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323040.png" /> is reversible if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323041.png" /> and irreversible if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323042.png" /> (an arbitrary topological manifold of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323043.png" /> admits a handle decomposition and there exist four-dimensional topological manifolds for which this is not true). |
| − | is reversible if | ||
| − | and irreversible if | ||
| − | an arbitrary topological manifold of dimension | ||
| − | admits a handle decomposition and there exist four-dimensional topological manifolds for which this is not true). | ||
| − | Similarly, if | + | Similarly, if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323044.png" /> the arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323045.png" /> is reversible (and moreover in a unique way). |
| − | the arrow | ||
| − | is reversible (and moreover in a unique way). | ||
| − | The question on the reversibility of the arrow | + | The question on the reversibility of the arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323046.png" /> gives the classical unsolved problem on the triangulability of arbitrary topological manifolds. |
| − | gives the classical unsolved problem on the triangulability of arbitrary topological manifolds. | ||
| − | The arrow | + | The arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323047.png" /> is irreversible in the strong sense (there exist Poincaré polyhedra that are homotopy inequivalent to any homology manifold). |
| − | is irreversible in the strong sense (there exist Poincaré polyhedra that are homotopy inequivalent to any homology manifold). | ||
| − | The arrow | + | The arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323048.png" /> expresses a theorem on the homotopy equivalence of an arbitrary homology manifold of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323049.png" /> to a topological manifold. |
| − | expresses a theorem on the homotopy equivalence of an arbitrary homology manifold of dimension | ||
| − | to a topological manifold. | ||
| − | The arrow | + | The arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323050.png" /> expresses the theorem on the homotopy equivalence of an arbitrary topological manifold to a polyhedron. |
| − | expresses the theorem on the homotopy equivalence of an arbitrary topological manifold to a polyhedron. | ||
| − | The imbedding | + | The imbedding <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323051.png" /> expresses that an arbitrary topological manifold is an <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323052.png" />. |
| − | expresses that an arbitrary topological manifold is an | ||
| − | The similar question for the arrows | + | The similar question for the arrows <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323053.png" /> has been solved using the theory of stable bundles (respectively, vector, piecewise-linear, topological, and spherical bundles), i.e. by examining the homotopy classes of mappings of a manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323054.png" /> into the corresponding classifying spaces BO, BPL, BTOP, BG. |
| − | has been solved using the theory of stable bundles (respectively, vector, piecewise-linear, topological, and spherical bundles), i.e. by examining the homotopy classes of mappings of a manifold | ||
| − | into the corresponding classifying spaces BO, BPL, BTOP, BG. | ||
There exist canonical composition mappings | There exist canonical composition mappings | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323055.png" /></td> <td valign="top" style="width:5%;text-align:right;">(2)</td></tr></table> | |
| − | |||
| − | |||
of which the homotopy fibres and the homotopy fibres of their compositions are denoted, respectively, by the symbols | of which the homotopy fibres and the homotopy fibres of their compositions are denoted, respectively, by the symbols | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323056.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | |||
| − | For every manifold | + | For every manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323057.png" /> from a category <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323058.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323059.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323060.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323061.png" /> there exists a normal stable bundle, i.e. a canonical mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323062.png" /> from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323063.png" /> into the corresponding classifying space. |
| − | from a category | ||
| − | |||
| − | |||
| − | |||
| − | there exists a normal stable bundle, i.e. a canonical mapping | ||
| − | from | ||
| − | into the corresponding classifying space. | ||
| − | In the transition from a "narrow" category of manifolds to a "wider" one, for example, from smooth to piecewise-linear, the mapping | + | In the transition from a "narrow" category of manifolds to a "wider" one, for example, from smooth to piecewise-linear, the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323064.png" /> is composed with the corresponding mappings (2). Hence, for example, for a PL-manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323065.png" /> there exists a smooth manifold PL-homeomorphic to it (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323066.png" /> is said to be smoothable) only if the lifting problem (3), the homotopy obstruction to the solution of which lies in the groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323067.png" />, is solvable: |
| − | is composed with the corresponding mappings (2). Hence, for example, for a PL-manifold | ||
| − | there exists a smooth manifold PL-homeomorphic to it ( | ||
| − | is said to be smoothable) only if the lifting problem (3), the homotopy obstruction to the solution of which lies in the groups | ||
| − | is solvable: | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323068.png" /></td> <td valign="top" style="width:5%;text-align:right;">(3)</td></tr></table> | |
| − | Here it turns out that the solvability of (3) is not only necessary but also sufficient for the smoothability of a PL-manifold | + | Here it turns out that the solvability of (3) is not only necessary but also sufficient for the smoothability of a PL-manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323069.png" /> (and all non-equivalent smoothings are in bijective correspondence with the set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323070.png" /> of homotopy classes of mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323071.png" />). |
| − | and all non-equivalent smoothings are in bijective correspondence with the set | ||
| − | of homotopy classes of mappings | ||
| − | By replacing | + | By replacing <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323072.png" /> by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323073.png" />, the same holds for the smoothability of topological manifolds <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323074.png" /> of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323075.png" />, and also (by replacing <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323076.png" /> by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323077.png" />) for their <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323078.png" />-triangulations. The homotopy group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323079.png" /> is isomorphic to the group of classes of oriented diffeomorphic smooth manifolds obtained by glueing the boundaries of two <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323080.png" />-dimensional spheres. This group is finite for all <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323081.png" /> (and is even trivial for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323082.png" />). Therefore, the number of non-equivalent smoothings of an arbitrary PL-manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323083.png" /> is finite and is bounded above by the number |
| − | by | ||
| − | the same holds for the smoothability of topological manifolds | ||
| − | of dimension | ||
| − | and also (by replacing | ||
| − | by | ||
| − | for their | ||
| − | triangulations. The homotopy group | ||
| − | is isomorphic to the group of classes of oriented diffeomorphic smooth manifolds obtained by glueing the boundaries of two | ||
| − | dimensional spheres. This group is finite for all | ||
| − | and is even trivial for | ||
| − | Therefore, the number of non-equivalent smoothings of an arbitrary PL-manifold | ||
| − | is finite and is bounded above by the number | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323084.png" /></td> </tr></table> | |
| − | |||
| − | |||
| − | |||
| − | The homotopy group | + | The homotopy group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323085.png" /> vanishes, with one exception: <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323086.png" />. Thus, the existence of a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323087.png" />-triangulation of a topological manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323088.png" /> of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323089.png" /> is determined by the vanishing of a certain cohomology class <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323090.png" />, while the set of non-equivalent <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323091.png" />-triangulations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323092.png" /> is in bijective correspondence with the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323093.png" />. |
| − | vanishes, with one exception: | ||
| − | Thus, the existence of a | ||
| − | triangulation of a topological manifold | ||
| − | of dimension | ||
| − | is determined by the vanishing of a certain cohomology class | ||
| − | while the set of non-equivalent | ||
| − | triangulations of | ||
| − | is in bijective correspondence with the group | ||
| − | The group | + | The group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323094.png" /> coincides with the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323095.png" /> if <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323096.png" /> and differs from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323097.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323098.png" /> by the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t09323099.png" />. The number of non-equivalent smoothings of a topological manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230100.png" /> of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230101.png" /> is finite and is bounded above by the number <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230102.png" />. |
| − | coincides with the group | ||
| − | if | ||
| − | and differs from | ||
| − | for | ||
| − | by the group | ||
| − | The number of non-equivalent smoothings of a topological manifold | ||
| − | of dimension | ||
| − | is finite and is bounded above by the number | ||
Similar results are not valid for Poincaré polyhedra. | Similar results are not valid for Poincaré polyhedra. | ||
| − | + | <table class="eq" style="width:100%;"> <tr><td valign="top" style="width:94%;text-align:center;"><img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230103.png" /></td> <td valign="top" style="width:5%;text-align:right;">(4)</td></tr></table> | |
| − | Of course, the existence of a lifting, for example, in (4) is a necessary condition for the existence of a PL-manifold homotopy equivalent to the Poincaré polyhedron | + | Of course, the existence of a lifting, for example, in (4) is a necessary condition for the existence of a PL-manifold homotopy equivalent to the Poincaré polyhedron <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230104.png" />, but, generally speaking, it ensures (for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230105.png" />) only the existence of a PL-manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230106.png" /> and a mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230107.png" /> of degree 1 such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230108.png" />. The transformation of this manifold into a manifold that is homotopy equivalent to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230109.png" /> requires the technique of [[Surgery|surgery]] (reconstruction), initially developed by S.P. Novikov for the case when <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230110.png" /> is a simply-connected smooth manifold of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230111.png" />. For simply-connected <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230112.png" /> this technique has been generalized to the case under consideration. Thus, for a simply-connected Poincaré polyhedron <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230113.png" /> a PL-manifold of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230114.png" /> homotopy equivalent to it exists if and only a lifting (4) exists. The problem of the existence of topological or smooth manifolds that are homotopy equivalent to an (even simply-connected) Poincaré polyhedron is still more complicated. |
| − | but, generally speaking, it ensures (for | ||
| − | only the existence of a PL-manifold | ||
| − | and a mapping | ||
| − | of degree 1 such that | ||
| − | The transformation of this manifold into a manifold that is homotopy equivalent to | ||
| − | requires the technique of [[Surgery|surgery]] (reconstruction), initially developed by S.P. Novikov for the case when | ||
| − | is a simply-connected smooth manifold of dimension | ||
| − | For simply-connected | ||
| − | this technique has been generalized to the case under consideration. Thus, for a simply-connected Poincaré polyhedron | ||
| − | a PL-manifold of dimension | ||
| − | homotopy equivalent to it exists if and only a lifting (4) exists. The problem of the existence of topological or smooth manifolds that are homotopy equivalent to an (even simply-connected) Poincaré polyhedron is still more complicated. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[1]</TD> <TD valign="top"> S.P. Novikov, "On manifolds with free abelian group and their application" ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''30''' (1966) pp. 207–246 (In Russian)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> J. Madsen, R.J. Milgram, "The classifying spaces for surgery and cobordism of manifolds" , Princeton Univ. Press (1979)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> F. Latour, "Double suspension d'une sphere d'homologie [d'après R. Edwards]" , ''Sem. Bourbaki Exp. 515'' , ''Lect. notes in math.'' , '''710''' , Springer (1979) pp. 169–186</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> M.H. Freedman, "The topology of four-dimensional manifolds" ''J. Differential Geom.'' , '''17''' (1982) pp. 357–453</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> F. Quinn, "Ends of maps III. Dimensions 4 and 5" ''J. Differential Geom.'' , '''17''' (1982) pp. 503–521</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> R. Mandelbaum, "Four-dimensional topology: an introduction" ''Bull. Amer. Math. Soc.'' , '''2''' (1980) pp. 1–159</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> R. Lashof, "The immersion approach to triangulation and smoothing" A. Liulevicius (ed.) , ''Algebraic Topology (Madison, 1970)'' , ''Proc. Symp. Pure Math.'' , '''22''' , Amer. Math. Soc. (1971) pp. 131–164</TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> R.D. Edwards, "Approximating certain cell-like maps by homeomorphisms" ''Notices Amer. Math. Soc.'' , '''24''' : 7 (1977) pp. A649</TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> F. Quinn, "The topological characterization of manifolds" ''Abstracts Amer. Math. Soc.'' , '''1''' : 7 (1980) pp. 613–614</TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> J.W. Cannon, "The recognition problem: what is a topological manifold" ''Bull. Amer. Math. Soc.'' , '''84''' : 5 (1978) pp. 832–866</TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> M. Spivak, "Spaces satisfying Poincaré duality" ''Topology'' , '''6''' (1967) pp. 77–101</TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> N.H. Kuiper, "A short history of triangulation and related matters" P.C. Baayen (ed.) D. van Dulst (ed.) J. Oosterhoff (ed.) , ''Bicentennial Congress Wisk. Genootschap (Amsterdam 1978)'' , ''Math. Centre Tracts'' , '''100''' , CWI (1979) pp. 61–79</TD></TR></table> | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> S.P. Novikov, "On manifolds with free abelian group and their application" ''Izv. Akad. Nauk SSSR Ser. Mat.'' , '''30''' (1966) pp. 207–246 (In Russian)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> J. Madsen, R.J. Milgram, "The classifying spaces for surgery and cobordism of manifolds" , Princeton Univ. Press (1979)</TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> F. Latour, "Double suspension d'une sphere d'homologie [d'après R. Edwards]" , ''Sem. Bourbaki Exp. 515'' , ''Lect. notes in math.'' , '''710''' , Springer (1979) pp. 169–186</TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> M.H. Freedman, "The topology of four-dimensional manifolds" ''J. Differential Geom.'' , '''17''' (1982) pp. 357–453</TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> F. Quinn, "Ends of maps III. Dimensions 4 and 5" ''J. Differential Geom.'' , '''17''' (1982) pp. 503–521</TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> R. Mandelbaum, "Four-dimensional topology: an introduction" ''Bull. Amer. Math. Soc.'' , '''2''' (1980) pp. 1–159</TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> R. Lashof, "The immersion approach to triangulation and smoothing" A. Liulevicius (ed.) , ''Algebraic Topology (Madison, 1970)'' , ''Proc. Symp. Pure Math.'' , '''22''' , Amer. Math. Soc. (1971) pp. 131–164</TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> R.D. Edwards, "Approximating certain cell-like maps by homeomorphisms" ''Notices Amer. Math. Soc.'' , '''24''' : 7 (1977) pp. A649</TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> F. Quinn, "The topological characterization of manifolds" ''Abstracts Amer. Math. Soc.'' , '''1''' : 7 (1980) pp. 613–614</TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> J.W. Cannon, "The recognition problem: what is a topological manifold" ''Bull. Amer. Math. Soc.'' , '''84''' : 5 (1978) pp. 832–866</TD></TR><TR><TD valign="top">[11]</TD> <TD valign="top"> M. Spivak, "Spaces satisfying Poincaré duality" ''Topology'' , '''6''' (1967) pp. 77–101</TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> N.H. Kuiper, "A short history of triangulation and related matters" P.C. Baayen (ed.) D. van Dulst (ed.) J. Oosterhoff (ed.) , ''Bicentennial Congress Wisk. Genootschap (Amsterdam 1978)'' , ''Math. Centre Tracts'' , '''100''' , CWI (1979) pp. 61–79</TD></TR></table> | ||
| + | |||
| + | |||
====Comments==== | ====Comments==== | ||
| − | It was found recently [[#References|[a1]]] that the behaviour of smooth manifolds of dimension | + | It was found recently [[#References|[a1]]] that the behaviour of smooth manifolds of dimension <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230115.png" /> is radically different from those in dimensions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230116.png" />. Among very numerous recent results one has: |
| − | is radically different from those in dimensions | ||
| − | Among very numerous recent results one has: | ||
| − | i) There is a countably infinite family of smooth, compact, simply-connected | + | i) There is a countably infinite family of smooth, compact, simply-connected <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230117.png" />-manifolds, all mutually homeomorphic but with distinct smooth structure. |
| − | manifolds, all mutually homeomorphic but with distinct smooth structure. | ||
| − | ii) There is an uncountable family of smooth | + | ii) There is an uncountable family of smooth <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230118.png" />-manifolds, each homeomorphic to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230119.png" /> but with mutually distinct smooth structure. |
| − | manifolds, each homeomorphic to | ||
| − | but with mutually distinct smooth structure. | ||
| − | iii) There are simply-connected smooth | + | iii) There are simply-connected smooth <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230120.png" />-manifolds which are <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230121.png" />-cobordant (cf. [[H-cobordism|<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230122.png" />-cobordism]]) but not diffeomorphic. |
| − | manifolds which are | ||
| − | cobordant (cf. [[H-cobordism| | ||
| − | cobordism]]) but not diffeomorphic. | ||
For the lifting problem (3) see [[#References|[a2]]]–[[#References|[a3]]]. | For the lifting problem (3) see [[#References|[a2]]]–[[#References|[a3]]]. | ||
| − | For the Kirby–Siebenmann theorem, the arrow | + | For the Kirby–Siebenmann theorem, the arrow <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t093/t093230/t093230123.png" />, see also [[#References|[a4]]]. |
| − | see also [[#References|[a4]]]. | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> S.M. Donaldson, "The geometry of 4-manifolds" A.M. Gleason (ed.) , ''Proc. Internat. Congress Mathematicians (Berkeley, 1986)'' , Amer. Math. Soc. (1987) pp. 43–54</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> M.W. Hirsch, B. Mazur, "Smoothings of piecewise-linear manifolds" , Princeton Univ. Press (1974)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> R. Lashof, M. Rothenberg, "Microbundles and smoothing" ''Topology'' , '''3''' (1965) pp. 357–388</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> R.C. Kirby, L.C. Siebenmann, "Foundational essays on topological manifolds, smoothings, and triangulations" , Princeton Univ. Press (1977)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> S.M. Donaldson, "The geometry of 4-manifolds" A.M. Gleason (ed.) , ''Proc. Internat. Congress Mathematicians (Berkeley, 1986)'' , Amer. Math. Soc. (1987) pp. 43–54</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> M.W. Hirsch, B. Mazur, "Smoothings of piecewise-linear manifolds" , Princeton Univ. Press (1974)</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> R. Lashof, M. Rothenberg, "Microbundles and smoothing" ''Topology'' , '''3''' (1965) pp. 357–388</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> R.C. Kirby, L.C. Siebenmann, "Foundational essays on topological manifolds, smoothings, and triangulations" , Princeton Univ. Press (1977)</TD></TR></table> | ||
Revision as of 14:53, 7 June 2020
The branch of the theory of manifolds (cf. Manifold) concerned with the study of relations between different types of manifolds.
The most important types of finite-dimensional manifolds and relations between them are illustrated in (1).
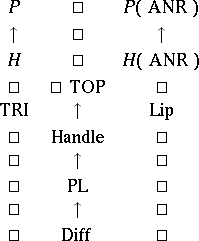 | (1) |
Here  is the category of differentiable (smooth) manifolds;
is the category of differentiable (smooth) manifolds; 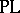 is the category of piecewise-linear (combinatorial) manifolds;
is the category of piecewise-linear (combinatorial) manifolds; 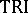 is the category of topological manifolds that are polyhedra;
is the category of topological manifolds that are polyhedra; 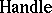 is the category of topological manifolds admitting a topological decomposition into handles;
is the category of topological manifolds admitting a topological decomposition into handles; 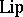 is the category of Lipschitz manifolds (with Lipschitz transition mappings between local charts);
is the category of Lipschitz manifolds (with Lipschitz transition mappings between local charts);  is the category of topological manifolds (Hausdorff and with a countable base);
is the category of topological manifolds (Hausdorff and with a countable base); 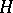 is the category of polyhedral homology manifolds without boundary (polyhedra, the boundary of the star of each vertex of which has the homology of the sphere of corresponding dimension);
is the category of polyhedral homology manifolds without boundary (polyhedra, the boundary of the star of each vertex of which has the homology of the sphere of corresponding dimension); 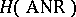 is the category of generalized manifolds (finite-dimensional absolute neighbourhood retracts
is the category of generalized manifolds (finite-dimensional absolute neighbourhood retracts 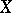 that are homology manifolds without boundary, i.e. with the property that for any point
that are homology manifolds without boundary, i.e. with the property that for any point 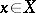 the group
the group 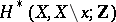 is isomorphic to the group
is isomorphic to the group 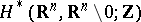 );
);  is the category of Poincaré spaces (finite-dimensional absolute neighbourhood retracts
is the category of Poincaré spaces (finite-dimensional absolute neighbourhood retracts 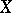 for which there exists a number
for which there exists a number  and an element
and an element 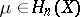 such that
such that  when
when  , and the mapping
, and the mapping 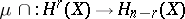 is an isomorphism for all
is an isomorphism for all  ); and
); and 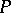 is the category of Poincaré polyhedra (the subcategory of the preceding category consisting of polyhedra).
is the category of Poincaré polyhedra (the subcategory of the preceding category consisting of polyhedra).
The arrows of (1), apart from the 3 lower ones and the arrows  , denote functors with the structure of forgetting functors. The arrow
, denote functors with the structure of forgetting functors. The arrow  expresses Whitehead's theorem on the triangulability of smooth manifolds. In dimensions
expresses Whitehead's theorem on the triangulability of smooth manifolds. In dimensions 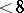 this arrow is reversible (an arbitrary
this arrow is reversible (an arbitrary 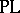 -manifold is smoothable) but in dimensions
-manifold is smoothable) but in dimensions 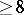 there are non-smoothable
there are non-smoothable 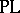 -manifolds and even
-manifolds and even 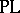 -manifolds that are homotopy inequivalent to any smooth manifold. The imbedding
-manifolds that are homotopy inequivalent to any smooth manifold. The imbedding 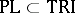 is also irreversible in the same strong sense (there exist polyhedral manifolds of dimension
is also irreversible in the same strong sense (there exist polyhedral manifolds of dimension 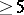 that are homotopy inequivalent to any
that are homotopy inequivalent to any 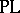 -manifold). Here already for the sphere
-manifold). Here already for the sphere 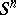 ,
, 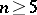 , there exist triangulations in which it is not a
, there exist triangulations in which it is not a  -manifold.
-manifold.
The arrow 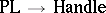 expresses the fact that every
expresses the fact that every 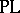 -manifold has a handle decomposition.
-manifold has a handle decomposition.
The arrow 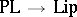 expresses the theorem on the existence of a Lipschitz structure on an arbitrary
expresses the theorem on the existence of a Lipschitz structure on an arbitrary 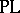 -manifold.
-manifold.
The arrow 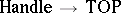 is reversible if
is reversible if  and irreversible if
and irreversible if 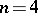 (an arbitrary topological manifold of dimension
(an arbitrary topological manifold of dimension 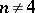 admits a handle decomposition and there exist four-dimensional topological manifolds for which this is not true).
admits a handle decomposition and there exist four-dimensional topological manifolds for which this is not true).
Similarly, if 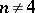 the arrow
the arrow 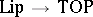 is reversible (and moreover in a unique way).
is reversible (and moreover in a unique way).
The question on the reversibility of the arrow 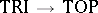 gives the classical unsolved problem on the triangulability of arbitrary topological manifolds.
gives the classical unsolved problem on the triangulability of arbitrary topological manifolds.
The arrow 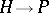 is irreversible in the strong sense (there exist Poincaré polyhedra that are homotopy inequivalent to any homology manifold).
is irreversible in the strong sense (there exist Poincaré polyhedra that are homotopy inequivalent to any homology manifold).
The arrow 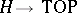 expresses a theorem on the homotopy equivalence of an arbitrary homology manifold of dimension
expresses a theorem on the homotopy equivalence of an arbitrary homology manifold of dimension  to a topological manifold.
to a topological manifold.
The arrow 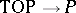 expresses the theorem on the homotopy equivalence of an arbitrary topological manifold to a polyhedron.
expresses the theorem on the homotopy equivalence of an arbitrary topological manifold to a polyhedron.
The imbedding 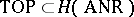 expresses that an arbitrary topological manifold is an
expresses that an arbitrary topological manifold is an 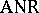 .
.
The similar question for the arrows  has been solved using the theory of stable bundles (respectively, vector, piecewise-linear, topological, and spherical bundles), i.e. by examining the homotopy classes of mappings of a manifold
has been solved using the theory of stable bundles (respectively, vector, piecewise-linear, topological, and spherical bundles), i.e. by examining the homotopy classes of mappings of a manifold 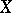 into the corresponding classifying spaces BO, BPL, BTOP, BG.
into the corresponding classifying spaces BO, BPL, BTOP, BG.
There exist canonical composition mappings
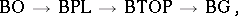 | (2) |
of which the homotopy fibres and the homotopy fibres of their compositions are denoted, respectively, by the symbols
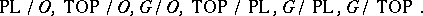 |
For every manifold 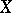 from a category
from a category 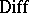 ,
, 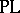 ,
, 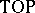 ,
, 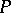 there exists a normal stable bundle, i.e. a canonical mapping
there exists a normal stable bundle, i.e. a canonical mapping 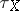 from
from 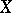 into the corresponding classifying space.
into the corresponding classifying space.
In the transition from a "narrow" category of manifolds to a "wider" one, for example, from smooth to piecewise-linear, the mapping 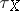 is composed with the corresponding mappings (2). Hence, for example, for a PL-manifold
is composed with the corresponding mappings (2). Hence, for example, for a PL-manifold 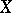 there exists a smooth manifold PL-homeomorphic to it (
there exists a smooth manifold PL-homeomorphic to it (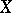 is said to be smoothable) only if the lifting problem (3), the homotopy obstruction to the solution of which lies in the groups
is said to be smoothable) only if the lifting problem (3), the homotopy obstruction to the solution of which lies in the groups  , is solvable:
, is solvable:
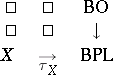 | (3) |
Here it turns out that the solvability of (3) is not only necessary but also sufficient for the smoothability of a PL-manifold 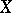 (and all non-equivalent smoothings are in bijective correspondence with the set
(and all non-equivalent smoothings are in bijective correspondence with the set 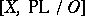 of homotopy classes of mappings
of homotopy classes of mappings 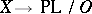 ).
).
By replacing 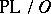 by
by 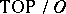 , the same holds for the smoothability of topological manifolds
, the same holds for the smoothability of topological manifolds 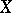 of dimension
of dimension 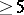 , and also (by replacing
, and also (by replacing 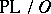 by
by 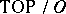 ) for their
) for their 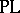 -triangulations. The homotopy group
-triangulations. The homotopy group  is isomorphic to the group of classes of oriented diffeomorphic smooth manifolds obtained by glueing the boundaries of two
is isomorphic to the group of classes of oriented diffeomorphic smooth manifolds obtained by glueing the boundaries of two 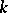 -dimensional spheres. This group is finite for all
-dimensional spheres. This group is finite for all 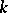 (and is even trivial for
(and is even trivial for 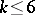 ). Therefore, the number of non-equivalent smoothings of an arbitrary PL-manifold
). Therefore, the number of non-equivalent smoothings of an arbitrary PL-manifold 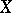 is finite and is bounded above by the number
is finite and is bounded above by the number
 |
The homotopy group  vanishes, with one exception:
vanishes, with one exception: 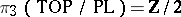 . Thus, the existence of a
. Thus, the existence of a  -triangulation of a topological manifold
-triangulation of a topological manifold 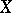 of dimension
of dimension 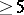 is determined by the vanishing of a certain cohomology class
is determined by the vanishing of a certain cohomology class  , while the set of non-equivalent
, while the set of non-equivalent 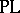 -triangulations of
-triangulations of  is in bijective correspondence with the group
is in bijective correspondence with the group 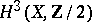 .
.
The group 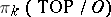 coincides with the group
coincides with the group  if
if 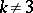 and differs from
and differs from  for
for 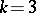 by the group
by the group 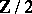 . The number of non-equivalent smoothings of a topological manifold
. The number of non-equivalent smoothings of a topological manifold 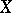 of dimension
of dimension 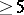 is finite and is bounded above by the number
is finite and is bounded above by the number 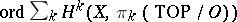 .
.
Similar results are not valid for Poincaré polyhedra.
 | (4) |
Of course, the existence of a lifting, for example, in (4) is a necessary condition for the existence of a PL-manifold homotopy equivalent to the Poincaré polyhedron 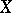 , but, generally speaking, it ensures (for
, but, generally speaking, it ensures (for 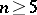 ) only the existence of a PL-manifold
) only the existence of a PL-manifold 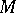 and a mapping
and a mapping  of degree 1 such that
of degree 1 such that 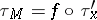 . The transformation of this manifold into a manifold that is homotopy equivalent to
. The transformation of this manifold into a manifold that is homotopy equivalent to 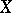 requires the technique of surgery (reconstruction), initially developed by S.P. Novikov for the case when
requires the technique of surgery (reconstruction), initially developed by S.P. Novikov for the case when 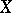 is a simply-connected smooth manifold of dimension
is a simply-connected smooth manifold of dimension 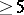 . For simply-connected
. For simply-connected 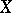 this technique has been generalized to the case under consideration. Thus, for a simply-connected Poincaré polyhedron
this technique has been generalized to the case under consideration. Thus, for a simply-connected Poincaré polyhedron 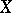 a PL-manifold of dimension
a PL-manifold of dimension 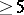 homotopy equivalent to it exists if and only a lifting (4) exists. The problem of the existence of topological or smooth manifolds that are homotopy equivalent to an (even simply-connected) Poincaré polyhedron is still more complicated.
homotopy equivalent to it exists if and only a lifting (4) exists. The problem of the existence of topological or smooth manifolds that are homotopy equivalent to an (even simply-connected) Poincaré polyhedron is still more complicated.
References
| [1] | S.P. Novikov, "On manifolds with free abelian group and their application" Izv. Akad. Nauk SSSR Ser. Mat. , 30 (1966) pp. 207–246 (In Russian) |
| [2] | J. Madsen, R.J. Milgram, "The classifying spaces for surgery and cobordism of manifolds" , Princeton Univ. Press (1979) |
| [3] | F. Latour, "Double suspension d'une sphere d'homologie [d'après R. Edwards]" , Sem. Bourbaki Exp. 515 , Lect. notes in math. , 710 , Springer (1979) pp. 169–186 |
| [4] | M.H. Freedman, "The topology of four-dimensional manifolds" J. Differential Geom. , 17 (1982) pp. 357–453 |
| [5] | F. Quinn, "Ends of maps III. Dimensions 4 and 5" J. Differential Geom. , 17 (1982) pp. 503–521 |
| [6] | R. Mandelbaum, "Four-dimensional topology: an introduction" Bull. Amer. Math. Soc. , 2 (1980) pp. 1–159 |
| [7] | R. Lashof, "The immersion approach to triangulation and smoothing" A. Liulevicius (ed.) , Algebraic Topology (Madison, 1970) , Proc. Symp. Pure Math. , 22 , Amer. Math. Soc. (1971) pp. 131–164 |
| [8] | R.D. Edwards, "Approximating certain cell-like maps by homeomorphisms" Notices Amer. Math. Soc. , 24 : 7 (1977) pp. A649 |
| [9] | F. Quinn, "The topological characterization of manifolds" Abstracts Amer. Math. Soc. , 1 : 7 (1980) pp. 613–614 |
| [10] | J.W. Cannon, "The recognition problem: what is a topological manifold" Bull. Amer. Math. Soc. , 84 : 5 (1978) pp. 832–866 |
| [11] | M. Spivak, "Spaces satisfying Poincaré duality" Topology , 6 (1967) pp. 77–101 |
| [12] | N.H. Kuiper, "A short history of triangulation and related matters" P.C. Baayen (ed.) D. van Dulst (ed.) J. Oosterhoff (ed.) , Bicentennial Congress Wisk. Genootschap (Amsterdam 1978) , Math. Centre Tracts , 100 , CWI (1979) pp. 61–79 |
Comments
It was found recently [a1] that the behaviour of smooth manifolds of dimension 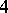 is radically different from those in dimensions
is radically different from those in dimensions 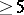 . Among very numerous recent results one has:
. Among very numerous recent results one has:
i) There is a countably infinite family of smooth, compact, simply-connected  -manifolds, all mutually homeomorphic but with distinct smooth structure.
-manifolds, all mutually homeomorphic but with distinct smooth structure.
ii) There is an uncountable family of smooth 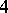 -manifolds, each homeomorphic to
-manifolds, each homeomorphic to 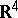 but with mutually distinct smooth structure.
but with mutually distinct smooth structure.
iii) There are simply-connected smooth 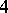 -manifolds which are
-manifolds which are 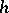 -cobordant (cf.
-cobordant (cf. 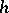 -cobordism) but not diffeomorphic.
-cobordism) but not diffeomorphic.
For the lifting problem (3) see [a2]–[a3].
For the Kirby–Siebenmann theorem, the arrow  , see also [a4].
, see also [a4].
References
| [a1] | S.M. Donaldson, "The geometry of 4-manifolds" A.M. Gleason (ed.) , Proc. Internat. Congress Mathematicians (Berkeley, 1986) , Amer. Math. Soc. (1987) pp. 43–54 |
| [a2] | M.W. Hirsch, B. Mazur, "Smoothings of piecewise-linear manifolds" , Princeton Univ. Press (1974) |
| [a3] | R. Lashof, M. Rothenberg, "Microbundles and smoothing" Topology , 3 (1965) pp. 357–388 |
| [a4] | R.C. Kirby, L.C. Siebenmann, "Foundational essays on topological manifolds, smoothings, and triangulations" , Princeton Univ. Press (1977) |
Topology of manifolds. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Topology_of_manifolds&oldid=48993