Difference between revisions of "Absolute neighbourhood extensor"
(Importing text file) |
(correction; see here: https://eudml.org/doc/212574) |
||
| Line 14: | Line 14: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> K. Borsuk, "Sur les rétractes" ''Fund. Math.'' , '''17''' (1931) pp. 152–170</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> K. Borsuk, "Über eine | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> K. Borsuk, "Sur les rétractes" ''Fund. Math.'' , '''17''' (1931) pp. 152–170</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> K. Borsuk, "Über eine Klasse von lokal zusammenhängenden Räumen" ''Fund. Math.'' , '''19''' (1932) pp. 220–242</TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> K. Borsuk, "Sur les prolongements des transformations continus" ''Fund. Math.'' , '''28''' (1936) pp. 99–110</TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> K. Borsuk, "Theory of retracts" , PWN (1967)</TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> R. Cauty, "Un espace métrique linéaire qui n'est pas un rétracte absolu" ''Fund. Math.'' , '''146''' (1994) pp. 85–99</TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> T.A. Chapman, "Lectures on Hilbert cube manifolds" , ''CBMS'' , '''28''' , Amer. Math. Soc. (1975)</TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> A.N. Dranišnikov, "On the dimension of the product of ANR-compacta" ''Dokl. Akad. Nauk SSSR'' , '''300''' : 5 (1988) pp. 1045–1049</TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> A.N. Dranišnikov, "On a problem of P.S. Alexandrov" ''Mat. Sb.'' , '''135''' (1988) pp. 551–557</TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> J. Dugundji, "An extension of Tietze's theorem" ''Pac. J. Math.'' , '''1''' (1951) pp. 353–367</TD></TR><TR><TD valign="top">[a10]</TD> <TD valign="top"> S.T. Hu, "Theory of retracts" , Wayne State Univ. Press (1965)</TD></TR><TR><TD valign="top">[a11]</TD> <TD valign="top"> S. Lefschetz, "On compact spaces" ''Ann. of Math.'' , '''32''' (1931) pp. 521–538</TD></TR><TR><TD valign="top">[a12]</TD> <TD valign="top"> A.T. Lundell, S. Weingram, "The topology of CW-complexes" , Litton (1969)</TD></TR><TR><TD valign="top">[a13]</TD> <TD valign="top"> E.V. Shchepin, "Finite-dimensional bicompact absolute neighborhood retracts are metrizable" ''Dokl. Akad. Nauk SSSR'' , '''233''' (1977) pp. 304–307 (In Russian)</TD></TR><TR><TD valign="top">[a14]</TD> <TD valign="top"> E.V. Shchepin, "On Tychonoff manifolds" ''Dokl. Akad. Nauk SSSR'' , '''246''' (1979) pp. 551–554 (In Russian)</TD></TR><TR><TD valign="top">[a15]</TD> <TD valign="top"> H. Toruńczyk, "On <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/a/a120/a120020/a12002035.png" />-images of the Hilbert cube and characterizations of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/a/a120/a120020/a12002036.png" />-manifolds" ''Fund. Math.'' , '''106''' (1980) pp. 31–40</TD></TR><TR><TD valign="top">[a16]</TD> <TD valign="top"> H. Toruńczyk, "Characterizing Hilbert space topology" ''Fund. Math.'' , '''111''' (1981) pp. 247–262</TD></TR><TR><TD valign="top">[a17]</TD> <TD valign="top"> J.E. West, "Mapping Hilbert cube manifolds to ANR's: a solution to a conjecture of Borsuk" ''Ann. of Math.'' , '''106''' (1977) pp. 1–18</TD></TR></table> |
Revision as of 10:01, 31 March 2017
ANE
For the time being, assume that all topological spaces under discussion are metrizable (cf. also Metrizable space). A space 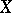 is called an absolute (neighbourhood) extensor, abbreviated AE (respectively, ANE), provided that for every space
is called an absolute (neighbourhood) extensor, abbreviated AE (respectively, ANE), provided that for every space 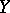 and every closed subspace
and every closed subspace 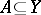 , every continuous function
, every continuous function 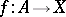 can be extended over
can be extended over  (respectively, over a neighbourhood of
(respectively, over a neighbourhood of 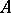 in
in 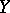 ). The classical Tietze extension theorem (cf. also Extension theorems) implies that familiar spaces such as the real line
). The classical Tietze extension theorem (cf. also Extension theorems) implies that familiar spaces such as the real line 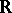 , the unit interval
, the unit interval  and the circle
and the circle 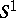 are absolute (neighbourhood) extensors. An absolute (neighbourhood) retract is a space
are absolute (neighbourhood) extensors. An absolute (neighbourhood) retract is a space 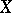 having the property that whenever
having the property that whenever 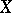 is embedded as a closed subset of a space
is embedded as a closed subset of a space 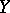 , then it is a (neighbourhood) retract of
, then it is a (neighbourhood) retract of 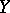 (cf. also Absolute retract for normal spaces; Retract of a topological space). It is a fundamental theorem that every AE (respectively, ANE) is an AR (respectively, ANR), and conversely. The theory of absolute (neighbourhood) retracts was initiated by K. Borsuk in [a1], [a2]. He proved his fundamental homotopy extension theorem in [a3]: If
(cf. also Absolute retract for normal spaces; Retract of a topological space). It is a fundamental theorem that every AE (respectively, ANE) is an AR (respectively, ANR), and conversely. The theory of absolute (neighbourhood) retracts was initiated by K. Borsuk in [a1], [a2]. He proved his fundamental homotopy extension theorem in [a3]: If 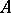 is a closed subspace of a space
is a closed subspace of a space 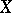 and
and 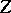 is an ANR and
is an ANR and 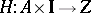 is a homotopy such that
is a homotopy such that 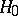 is extendable to a function
is extendable to a function  , then there is a homotopy
, then there is a homotopy 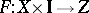 such that
such that  , and for every
, and for every 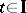 ,
, 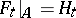 . For more details, see [a4] and [a10].
. For more details, see [a4] and [a10].
In 1951, J. Dugundji [a9] proved that a convex set in a locally convex vector space (cf. also Locally convex space) is an AR. This result was a major improvement over the Tietze extension theorem and was widely applied. The fundamental problem whether the local convexity assumption in this result could be dropped, was solved by R. Cauty [a5] in the negative. His counterexample used in an essential way a theorem of A.N. Dranishnikov [a8] about the existence of an infinite-dimensional compactum with finite cohomological dimension.
There are several topological characterizations of absolute (neighbourhood) retracts. The most useful one is due to S. Lefschetz [a11] and is in terms of partial realizations of polytopes in the space under consideration. It can be shown that if a space 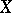 is dominated (cf. also Homotopy type) by a simplicial complex, then it has the homotopy type of another simplicial complex (see [a12]). Since it is not too hard to prove that every ANR is dominated by a simplicial complex (see [a10]), it follows, in particular, that every ANR has the homotopy type of some simplicial complex. But the natural question whether every compact ANR has the homotopy type of a compact simplicial complex, i.e. a finite polyhedron, remained unanswered for a long time. It was finally solved in the affirmative by J.E. West [a17] by using powerful results from T.A. Chapman [a6] in infinite-dimensional topology.
is dominated (cf. also Homotopy type) by a simplicial complex, then it has the homotopy type of another simplicial complex (see [a12]). Since it is not too hard to prove that every ANR is dominated by a simplicial complex (see [a10]), it follows, in particular, that every ANR has the homotopy type of some simplicial complex. But the natural question whether every compact ANR has the homotopy type of a compact simplicial complex, i.e. a finite polyhedron, remained unanswered for a long time. It was finally solved in the affirmative by J.E. West [a17] by using powerful results from T.A. Chapman [a6] in infinite-dimensional topology.
Another fundamental problem about absolute (neighbourhood) retracts was Borsuk's problem of whether for compact absolute (neighbourhood) retracts 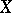 and
and 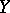 , the topological dimension of
, the topological dimension of 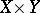 is equal to the sum of the dimensions of
is equal to the sum of the dimensions of 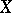 and
and 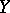 , respectively. This problem was solved by Dranishnikov [a7], who proved that there exist
, respectively. This problem was solved by Dranishnikov [a7], who proved that there exist 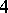 -dimensional compact absolute retracts
-dimensional compact absolute retracts 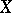 and
and 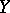 whose product is of dimension
whose product is of dimension 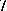 .
.
The theory of absolute (neighbourhood) retracts played a key role in infinite-dimensional topology. The fundamental topological characterization results of manifolds over the Hilbert cube and the Hilbert space, respectively, which are due to H. Toruńczyk [a15], [a16], are stated in terms of absolute (neighbourhood) retracts. Many of the remaining open problems in infinite-dimensional topology have been proven to actually be problems about absolute (neighbourhood) retracts.
The theory of absolute (neighbourhood) retracts in Tikhonov spaces (cf. also Tikhonov space). was mainly considered by E.V. Shchepin. He proved in [a13] that finite-dimensional compact absolute (neighbourhood) retracts are metrizable. He also found, in [a14], a very interesting topological characterization of all Tikhonov cubes of uncountable weight. ANR-theory plays a crucial role in this characterization.
References
| [a1] | K. Borsuk, "Sur les rétractes" Fund. Math. , 17 (1931) pp. 152–170 |
| [a2] | K. Borsuk, "Über eine Klasse von lokal zusammenhängenden Räumen" Fund. Math. , 19 (1932) pp. 220–242 |
| [a3] | K. Borsuk, "Sur les prolongements des transformations continus" Fund. Math. , 28 (1936) pp. 99–110 |
| [a4] | K. Borsuk, "Theory of retracts" , PWN (1967) |
| [a5] | R. Cauty, "Un espace métrique linéaire qui n'est pas un rétracte absolu" Fund. Math. , 146 (1994) pp. 85–99 |
| [a6] | T.A. Chapman, "Lectures on Hilbert cube manifolds" , CBMS , 28 , Amer. Math. Soc. (1975) |
| [a7] | A.N. Dranišnikov, "On the dimension of the product of ANR-compacta" Dokl. Akad. Nauk SSSR , 300 : 5 (1988) pp. 1045–1049 |
| [a8] | A.N. Dranišnikov, "On a problem of P.S. Alexandrov" Mat. Sb. , 135 (1988) pp. 551–557 |
| [a9] | J. Dugundji, "An extension of Tietze's theorem" Pac. J. Math. , 1 (1951) pp. 353–367 |
| [a10] | S.T. Hu, "Theory of retracts" , Wayne State Univ. Press (1965) |
| [a11] | S. Lefschetz, "On compact spaces" Ann. of Math. , 32 (1931) pp. 521–538 |
| [a12] | A.T. Lundell, S. Weingram, "The topology of CW-complexes" , Litton (1969) |
| [a13] | E.V. Shchepin, "Finite-dimensional bicompact absolute neighborhood retracts are metrizable" Dokl. Akad. Nauk SSSR , 233 (1977) pp. 304–307 (In Russian) |
| [a14] | E.V. Shchepin, "On Tychonoff manifolds" Dokl. Akad. Nauk SSSR , 246 (1979) pp. 551–554 (In Russian) |
| [a15] | H. Toruńczyk, "On 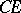 -images of the Hilbert cube and characterizations of -images of the Hilbert cube and characterizations of 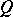 -manifolds" Fund. Math. , 106 (1980) pp. 31–40 -manifolds" Fund. Math. , 106 (1980) pp. 31–40 |
| [a16] | H. Toruńczyk, "Characterizing Hilbert space topology" Fund. Math. , 111 (1981) pp. 247–262 |
| [a17] | J.E. West, "Mapping Hilbert cube manifolds to ANR's: a solution to a conjecture of Borsuk" Ann. of Math. , 106 (1977) pp. 1–18 |
Absolute neighbourhood extensor. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Absolute_neighbourhood_extensor&oldid=11709