Difference between revisions of "Completely-continuous operator"
From Encyclopedia of Mathematics
(Importing text file) |
Wf6humboldt (talk | contribs) |
||
| Line 1: | Line 1: | ||
| − | |||
| − | |||
| − | + | ||
| + | ''Completely-Continuous Operator'' | ||
| + | |||
| + | A bounded linear operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239501.png" />, acting from a [[Banach space|Banach space]] <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239502.png" /> into another space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239503.png" />, that transforms weakly-convergent sequences in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239504.png" /> to norm-convergent sequences in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239505.png" />. Equivalently, an operator <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239508.png" /> is completely-continuous if it maps every relatively weakly compact subset of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239509.png" /> into a relatively compact subset of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c02395010.png" />. It is easy to see that every compact operator is completely continuous, however the converse is false. For example, recall that the Banach space ''X'' = ''l''<sub>1</sub> has the Schur Property, that is weak sequential and norm sequential convergence coincide. It follows that the identity operator from ''X'' to ''X'' is completely-continuous, but it is not compact since ''X'' is infinite-dimensional. If ''X'' is reflexive, then every completely-continuous operator is compact, so the two classes of operators do coincide in that case. In the past, the term "completely-continuous operator" was often used to mean compact operator which has sometimes resulted in confusion. | ||
| + | |||
| + | It can be assumed that the space <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239506.png" /> is separable (for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/c/c023/c023950/c0239507.png" /> this is not a necessary condition; however, the image of a completely-continuous operator is always separable). | ||
| + | |||
| + | |||
| + | The class of compact operators is the most important class of the set of completely-continuous operators (cf. [[Compact operator|Compact operator]]). | ||
| + | |||
====References==== | ====References==== | ||
| Line 11: | Line 18: | ||
====Comments==== | ====Comments==== | ||
| − | + | ||
====References==== | ====References==== | ||
<table><TR><TD valign="top">[a1]</TD> <TD valign="top"> N. Dunford, J.T. Schwartz, "Linear operators. General theory" , '''1''' , Interscience (1958)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> A.E. Taylor, D.C. Lay, "Introduction to functional analysis" , Wiley (1980)</TD></TR></table> | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> N. Dunford, J.T. Schwartz, "Linear operators. General theory" , '''1''' , Interscience (1958)</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> A.E. Taylor, D.C. Lay, "Introduction to functional analysis" , Wiley (1980)</TD></TR></table> | ||
Revision as of 02:14, 31 March 2013
Completely-Continuous Operator
A bounded linear operator 
, acting from a Banach space 
into another space 
, that transforms weakly-convergent sequences in 
to norm-convergent sequences in 
. Equivalently, an operator 
is completely-continuous if it maps every relatively weakly compact subset of 
into a relatively compact subset of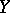 
. It is easy to see that every compact operator is completely continuous, however the converse is false. For example, recall that the Banach space X = l1 has the Schur Property, that is weak sequential and norm sequential convergence coincide. It follows that the identity operator from X to X is completely-continuous, but it is not compact since X is infinite-dimensional. If X is reflexive, then every completely-continuous operator is compact, so the two classes of operators do coincide in that case. In the past, the term "completely-continuous operator" was often used to mean compact operator which has sometimes resulted in confusion.
It can be assumed that the space 
is separable (for 
this is not a necessary condition; however, the image of a completely-continuous operator is always separable).
The class of compact operators is the most important class of the set of completely-continuous operators (cf. Compact operator).
References
| [1] | D. Hilbert, "Grundzüge einer allgemeinen Theorie der linearen Integralgleichungen" , Chelsea, reprint (1953) |
| [2] | F. Riesz, "Sur les opérations fonctionelles linéaires" C.R. Acad. Sci. Paris Sér. I Math. , 149 (1909) pp. 974–977 |
| [3] | S.S. Banach, "Théorie des opérations linéaires" , Hafner (1932) |
Comments
References
| [a1] | N. Dunford, J.T. Schwartz, "Linear operators. General theory" , 1 , Interscience (1958) |
| [a2] | A.E. Taylor, D.C. Lay, "Introduction to functional analysis" , Wiley (1980) |
How to Cite This Entry:
Completely-continuous operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Completely-continuous_operator&oldid=16228
Completely-continuous operator. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Completely-continuous_operator&oldid=16228
This article was adapted from an original article by M.I. Voitsekhovskii (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article