Difference between revisions of "Second fundamental form"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 43: | Line 43: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , Springer (1973)</TD></TR></table> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , Springer (1973) {{MR|0350630}} {{ZBL|0264.53001}} </TD></TR></table> |
Revision as of 12:13, 27 September 2012
of a surface
The quadratic form in the differentials of the coordinates on the surface which characterizes the local structure of the surface in a neighbourhood of an ordinary point. Let the surface be given by the equation
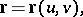 |
where  and
and  are internal coordinates on the surface; let
are internal coordinates on the surface; let
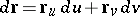 |
be the differential of the position vector  along a chosen direction
along a chosen direction  of displacement from a point
of displacement from a point  to a point
to a point  (see Fig.). Let
(see Fig.). Let
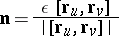 |
be the unit normal vector to the surface at the point 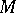 (here
(here 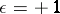 if the vector triplet
if the vector triplet  has right orientation, and
has right orientation, and  in the opposite case). The double principal linear part
in the opposite case). The double principal linear part 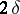 of the deviation
of the deviation  of the point
of the point  on the surface from the tangent plane at the point
on the surface from the tangent plane at the point  is
is
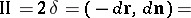 |
 |
it is known as the second fundamental form of the surface.

Figure: s083700a
The coefficients of the second fundamental form are usually denoted by
 |
or, in tensor notation,
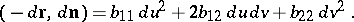 |
The tensor  is called the second fundamental tensor of the surface.
is called the second fundamental tensor of the surface.
See Fundamental forms of a surface for the connection between the second fundamental form and other surface forms.
Comments
References
| [a1] | W. Blaschke, K. Leichtweiss, "Elementare Differentialgeometrie" , Springer (1973) MR0350630 Zbl 0264.53001 |
Second fundamental form. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Second_fundamental_form&oldid=12634