Difference between revisions of "Wald identity"
(Importing text file) |
(→References: Feller: internal link) |
||
| Line 10: | Line 10: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> A. Wald, "Sequential analysis", Wiley (1952)</TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> W. Feller, [[Feller, "An introduction to probability theory and its applications"|"An introduction to probability theory and its applications"]], '''1''', Wiley (1957) pp. Chapt.14</TD></TR></table> |
| − | |||
| − | |||
====Comments==== | ====Comments==== | ||
Revision as of 11:22, 4 May 2012
An identity in sequential analysis which states that the mathematical expectation of the sum 
of a random number 
of independent, identically-distributed random variables 
is equal to the product of the mathematical expectations 
and 
:
 |
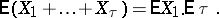A sufficient condition for the Wald identity to be valid is that the mathematical expectations 
and 
in fact exist, and for the random variable 
to be a Markov time (i.e. for any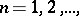 
the event 
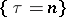is determined by the values of the random variables 
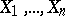or, which is the same thing, the event 
belongs to the 
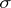-algebra generated by the random variables 
). Wald's identity is a particular case of a fundamental theorem in sequential analysis stating that
 | (*) |
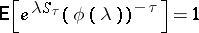for all complex 
for which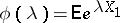 
exists and 
. It was established by A. Wald [1].
References
| [1] | A. Wald, "Sequential analysis", Wiley (1952) |
| [2] | W. Feller, "An introduction to probability theory and its applications", 1, Wiley (1957) pp. Chapt.14 |
Comments
The general result (*) is (also) referred to as Wald's formula.
References
| [a1] | A.V. [A.V. Skorokhod] Skorohod, "Random processes with independent increments" , Kluwer (1991) pp. 23 (Translated from Russian) |
Wald identity. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Wald_identity&oldid=18269