Lyapunov theorem

Lyapunov's theorem in probability theory is a theorem that establishes very general sufficient conditions for the convergence of the distributions of sums of independent random variables to the normal distribution. The precise statement of Lyapunov's theorem is as follows: Suppose that the independent random variables 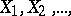have finite means 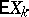, variances and absolute moments 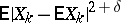, , 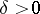and suppose also that 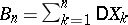is the variance of the sum of 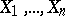. Then if for some ,

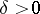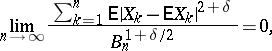(1)

the probability of the inequality

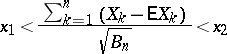(2)

tends to the limit

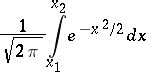(3)

as 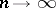, uniformly with respect to all values of 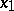and 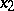. Condition (1) is called the Lyapunov condition. Lyapunov's theorem was stated and proved by A.M. Lyapunov in 1901 and was the final step in research of P.L. Chebyshev, A.A. Markov and Lyapunov on conditions for the applicability of the central limit theorem of probability theory. Later, conditions were established that extend Lyapunov's conditions and that are not only sufficient but also necessary. A final solution of the question in this direction was obtained by S.N. Bernstein [S.N. Bernshtein], J.W. Lindeberg and W. Feller. The power of the method of characteristic functions was demonstrated for the first time in Lyapunov's theorem.

Lyapunov also gave an upper bound (for 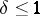) for the absolute value of the difference between the probability of (2) and its approximate value (3). This bound can be expressed in the following form: For ,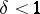

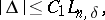and for ,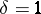

where 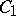and 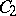are absolute constants and 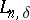is the fraction (the Lyapunov fraction) under the limit sign in (1). See also Berry–Esseen inequality.

References

[1]	A.M. Lyapunov, "Collected works", 1, Moscow-Leningrad (1954) pp. 157–176 (In Russian)
[2]	S.N. Bernshtein, "Probability theory", Moscow-Leningrad (1946) (In Russian)
[3]	W. Feller, "An introduction to probability theory and its applications", 2, Wiley (1966)

References

[a1]	R.G. Laha, V.K. Rohatgi, "Probability theory" , Wiley (1979)

Lyapunov's theorems in potential theory are theorems on the behaviour of potentials and the solution of the Dirichlet problem, obtained by A.M. Lyapunov in 1886–1902 (see ).

The theorem on the body of greatest potential: If there is a homogeneous body 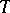in the Euclidean space 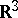, the energy of whose Newton potential, that is, the integral

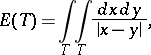attains its greatest value for a given volume, then this body is a ball.

The integral

is the energy of a homogeneous mass distribution of density 1 on the body 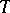. Later, T. Carleman (1919) proved that a body 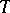for which the energy 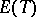attains its greatest value for a given volume actually exists.

First theorem on the normal derivatives of a double-layer potential: Let be a closed Lyapunov surface in 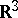, let 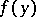be the density of the mass distributed on 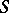, and suppose that one of the following two conditions is satisfied: a) 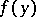is continuous on 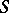, and the exponent in the Lyapunov condition on the angle 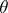between the normals to 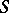at two points , that is, 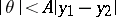(see Lyapunov surfaces and curves); or b) 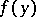is Hölder continuous with exponent 1, that is, 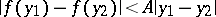; then if for the double-layer potential

one of the normal derivatives 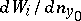inside 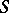or outside 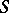at a point 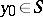exists, then the other also exists, and these derivatives coincide.

Second theorem on the normal derivatives of a double-layer potential: Under the assumptions of the previous theorem, suppose also that the density 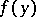satisfies the Lyapunov condition

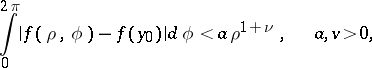where 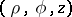are cylindrical coordinates inside the Lyapunov sphere (see Lyapunov surfaces and curves) with origin at a point 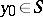and -axis directed along the normal . Then the double-layer potential

has both normal derivatives at .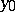

Theorem on the first derivatives of a simple-layer potential: Let 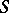be a closed Lyapunov surface and suppose that the density 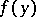is Hölder continuous, that is,

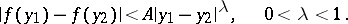Then the first-order partial derivatives 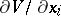, , 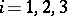, 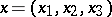of the simple-layer potential

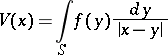are Hölder continuous with the same exponent 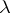in the closed interior domain 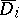and closed exterior domain .

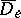In this theorem the Hölder continuity was only stated by Lyapunov; the proof was completed by N.M. Gunther (see [2]).

These theorems served Lyapunov as a basis for the construction of a strict theory of solvability of the Dirichlet problem by the method of integral equations. A monograph of Gunther was devoted to the development of the ideas of Lyapunov (see [2]); for generalizations to potentials of a more general form see [3].

References

[1a]	A.M. Lyapunov, , Collected works , 1 , Moscow (1954) pp. 26–32 (In Russian)
[1b]	A.M. Lyapunov, , Collected works , 1 , Moscow (1954) pp. 33–44 (In Russian)
[1c]	A.M. Lyapunov, "On certain questions connected with the Dirichlet problem" , Collected works , 1 , Moscow (1954) pp. 45–47; 48–100 (In Russian)
[1d]	A.M. Lyapunov, , Collected works , 1 , Moscow (1954) pp. 101–122 (In Russian)
[2]	N.M. Gunther, "Potential theory and its applications to basic problems of mathematical physics" , F. Ungar (1967) (Translated from Russian)
[3]	C. Miranda, "Partial differential equations of elliptic type" , Springer (1970) (Translated from Italian)

E.D. Solomentsev

References

[a1]	J. Král, "Integral operators in potential theory" , Lect. notes in math. , 823 , Springer (1980)