Difference between revisions of "Three-series theorem"
From Encyclopedia of Mathematics
(Importing text file) |
(→References: Feller: internal link) |
||
| Line 16: | Line 16: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> M. Loève, "Probability theory", Princeton Univ. Press (1963) pp. Sect. 16.3</TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> W. Feller, [[Feller, "An introduction to probability theory and its applications"|"An introduction to probability theory and its applications"]], '''2''', Wiley (1971) pp. Sect. IX.9</TD></TR></table> |
Revision as of 09:25, 4 May 2012
Kolmogorov three-series theorem, three-series criterion
For each 
, let 
be the truncation function 
for 
, 
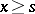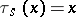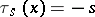for 
, 
for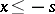 
.
Let 
be independent random variables with distributions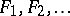 
. Consider the sums 
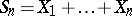, with distributions 
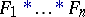. In order that these convolutions 
tend to a proper limit distribution 
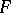as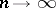 
, it is necessary and sufficient that for all 
,
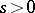 | (a1) |
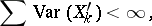 | (a2) |
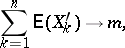 | (a3) |
where 
.
This can be reformulated as the Kolmogorov three-series theorem: The series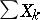 
converges with probability 
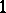if (a1)–(a3) hold, and it converges with probability zero otherwise.
References
| [a1] | M. Loève, "Probability theory", Princeton Univ. Press (1963) pp. Sect. 16.3 |
| [a2] | W. Feller, "An introduction to probability theory and its applications", 2, Wiley (1971) pp. Sect. IX.9 |
How to Cite This Entry:
Three-series theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Three-series_theorem&oldid=16674
Three-series theorem. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Three-series_theorem&oldid=16674