Difference between revisions of "Seifert conjecture"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 11: | Line 11: | ||
An orbit, or a trajectory, of a point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020010.png" /> is the set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020011.png" />. If an orbit is simple closed curve, then it is called circular, closed or periodic. | An orbit, or a trajectory, of a point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020010.png" /> is the set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020011.png" />. If an orbit is simple closed curve, then it is called circular, closed or periodic. | ||
| − | The [[Hopf fibration|Hopf fibration]] is an [[Essential mapping|essential mapping]] from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020012.png" /> onto <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020013.png" /> whose fibres, the inverse images of single points, are simple closed curves. The Seifert conjecture has its roots in a 1950 paper of H. Seifert [[#References|[a8]]], who proved that a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020014.png" /> non-singular vector field on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020015.png" /> possesses a periodic orbit if it is | + | The [[Hopf fibration|Hopf fibration]] is an [[Essential mapping|essential mapping]] from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020012.png" /> onto <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020013.png" /> whose fibres, the inverse images of single points, are simple closed curves. The Seifert conjecture has its roots in a 1950 paper of H. Seifert [[#References|[a8]]], who proved that a <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020014.png" /> non-singular vector field on <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020015.png" /> possesses a periodic orbit if it is "almost parallel" to the fibres of the Hopf fibration. |
The even-dimensional spheres do not admit non-singular vector fields, and a higher-dimensional version of the Seifert conjecture for the odd-dimensional spheres has been established in 1966 by F.W. Wilson [[#References|[a9]]] as follows: Any non-singular vector field on a smooth <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020016.png" />-dimensional manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020017.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020018.png" />, can be modified to a vector field with a set of isolated invariant <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020019.png" />-tori, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020020.png" />, so that for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020021.png" />: | The even-dimensional spheres do not admit non-singular vector fields, and a higher-dimensional version of the Seifert conjecture for the odd-dimensional spheres has been established in 1966 by F.W. Wilson [[#References|[a9]]] as follows: Any non-singular vector field on a smooth <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020016.png" />-dimensional manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020017.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020018.png" />, can be modified to a vector field with a set of isolated invariant <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020019.png" />-tori, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020020.png" />, so that for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020021.png" />: | ||
| Line 61: | Line 61: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> G. Kuperberg, "A volume-preserving counterexample to the Seifert conjecture" ''Comment. Math. Helv.'' , '''71''' (1996) pp. 70–97 {{MR|1371679}} {{ZBL|0859.57017}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> V.L. Ginzburg, "A smooth counterexample to the Hamiltonian Seifert conjecture in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020053.png" />" ''Internat. Math. Res. Notices'' , '''13''' (1997) pp. 641–650 {{MR|1459629}} {{ZBL|0913.58022}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> J. Harrison, "<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020054.png" /> counterexamples to the Seifert conjecture" ''Topology'' , '''27''' (1988) pp. 249–278 {{MR|0963630}} {{ZBL|0669.57011}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> H. Hofer, "Pseudoholomorphic curves in symplectizations with applications to the Weinstein conjecture in dimension three" ''Invent. Math.'' , '''114''' (1993) pp. 515–563 {{MR|1244912}} {{ZBL|0797.58023}} </TD></TR><TR><TD valign="top">[a5]</TD> <TD valign="top"> K. Kuperberg, "A smooth counterexample to the Seifert conjecture" ''Ann. of Math.'' , '''140''' (1994) pp. 723–732 {{MR|1307902}} {{ZBL|0856.57024}} </TD></TR><TR><TD valign="top">[a6]</TD> <TD valign="top"> G. Kuperberg, K. Kuperberg, "Generalized counterexamples to the Seifert conjecture" ''Ann. of Math.'' , '''144''' (1996) pp. 239–268 {{MR|1418899}} {{MR|1394969}} {{ZBL|0856.57026}} </TD></TR><TR><TD valign="top">[a7]</TD> <TD valign="top"> P.A. Schweitzer, "Counterexamples to the Seifert conjecture and opening closed leaves of foliations" ''Ann. of Math.'' , '''100''' (1974) pp. 386–400 {{MR|0356086}} {{ZBL|0295.57010}} </TD></TR><TR><TD valign="top">[a8]</TD> <TD valign="top"> H. Seifert, "Closed integral curves in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s130/s130200/s13020055.png" />-space and isotopic two-dimensional deformations" ''Proc. Amer. Math. Soc.'' , '''1''' (1950) pp. 287–302 {{MR|37508}} {{ZBL|}} </TD></TR><TR><TD valign="top">[a9]</TD> <TD valign="top"> F.W. Wilson, "On the minimal sets of non-singular vector fields" ''Ann. of Math.'' , '''84''' (1966) pp. 529–536 {{MR|0202155}} {{ZBL|0156.43803}} </TD></TR></table> |
Revision as of 17:01, 15 April 2012
The assertion that every non-singular (i.e. everywhere non-zero)  vector field on the three-dimensional sphere
vector field on the three-dimensional sphere 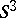 possesses a circular orbit.
possesses a circular orbit.
The conjecture is a three-dimensional analogue of the well-known hairy ball theorem, stating that there is no continuous non-singular vector field on the two-dimensional sphere 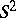 .
.
Integrating a  vector field results in a flow, which on a closed manifold
vector field results in a flow, which on a closed manifold  is a dynamical system, i.e. a mapping
is a dynamical system, i.e. a mapping  with the properties:
with the properties:
1) 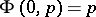 ; and
; and
2) 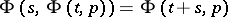 (the parameter
(the parameter 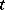 is usually interpreted as time; cf. also Dynamical system; Flow (continuous-time dynamical system)).
is usually interpreted as time; cf. also Dynamical system; Flow (continuous-time dynamical system)).
An orbit, or a trajectory, of a point  is the set
is the set 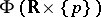 . If an orbit is simple closed curve, then it is called circular, closed or periodic.
. If an orbit is simple closed curve, then it is called circular, closed or periodic.
The Hopf fibration is an essential mapping from 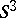 onto
onto 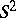 whose fibres, the inverse images of single points, are simple closed curves. The Seifert conjecture has its roots in a 1950 paper of H. Seifert [a8], who proved that a
whose fibres, the inverse images of single points, are simple closed curves. The Seifert conjecture has its roots in a 1950 paper of H. Seifert [a8], who proved that a 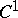 non-singular vector field on
non-singular vector field on  possesses a periodic orbit if it is "almost parallel" to the fibres of the Hopf fibration.
possesses a periodic orbit if it is "almost parallel" to the fibres of the Hopf fibration.
The even-dimensional spheres do not admit non-singular vector fields, and a higher-dimensional version of the Seifert conjecture for the odd-dimensional spheres has been established in 1966 by F.W. Wilson [a9] as follows: Any non-singular vector field on a smooth  -dimensional manifold
-dimensional manifold 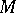 ,
, 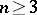 , can be modified to a vector field with a set of isolated invariant
, can be modified to a vector field with a set of isolated invariant 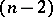 -tori,
-tori, 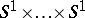 , so that for
, so that for 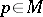 :
:
a) in both cases as 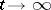 and as
and as 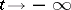 , the orbit
, the orbit 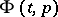 limits on one of the tori; and
limits on one of the tori; and
b) every orbit contained in one of the tori is dense in that torus. Thus, each of the spheres 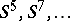 admits a non-singular vector field with no circular orbits.
admits a non-singular vector field with no circular orbits.
For his construction, Wilson introduced a plug, a special non-singular vector field on the  -dimensional disc
-dimensional disc 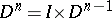 , where
, where  is the unit interval. The plug is constant and parallel to
is the unit interval. The plug is constant and parallel to  on the boundary of
on the boundary of 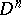 , and satisfies the trapped-orbit condition and the matched-ends condition (see below). The plug can be inserted in a non-singular vector field on an
, and satisfies the trapped-orbit condition and the matched-ends condition (see below). The plug can be inserted in a non-singular vector field on an  -dimensional manifold (the mechanism of insertion is illustrated in Fig.a1).
-dimensional manifold (the mechanism of insertion is illustrated in Fig.a1).
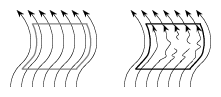
Figure: s130200a
Inserting a plug
The trapped-orbit condition guarantees that at least one orbit enters the disc  at the bottom,
at the bottom, 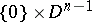 , but never leaves
, but never leaves  . The matched-ends condition means that if an orbit enters the disc
. The matched-ends condition means that if an orbit enters the disc  at the bottom and leaves
at the bottom and leaves  , then the exit point is the point on
, then the exit point is the point on  exactly above the entry point. By appropriately inserting a number of copies of a plug in a vector field on a manifold, Wilson changed the flow so that each orbit starts inside a plug and ends inside one, too.
exactly above the entry point. By appropriately inserting a number of copies of a plug in a vector field on a manifold, Wilson changed the flow so that each orbit starts inside a plug and ends inside one, too.
In dimension three, Wilson's theorem yields isolated circular orbits and does not resolve the Seifert conjecture. The conjecture remained unsolved until a remarkable construction by P.A. Schweitzer in 1972. His 1974 paper [a7] describes a three-dimensional plug without periodic orbits, which Schweitzer used to break the isolated periodic orbits, see Fig.a2 and Fig.a3. Inside the plug, instead of circular orbits, there are invariant Denjoy sets to trap the entering orbits. This, initially 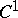 , construction was later improved to
, construction was later improved to 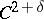 by J.M. Harrison [a3].
by J.M. Harrison [a3].

Figure: s130200b
Schweitzer's plug
Figure: s130200c
Breaking an orbit
Significant changes to the status of the Seifert conjecture came about in 1993 when H. Hofer [a4] proved that the Seifert conjecture holds for the Reeb vector field of a contact form on  (cf. also Contact structure). It was the next, after Seifert, advancement in the spirit of the conjecture.
(cf. also Contact structure). It was the next, after Seifert, advancement in the spirit of the conjecture.
On the other hand, a 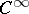 counterexample to the Seifert conjecture (in its original formulation) was found by K. Kuperberg [a5] the same year. This aperiodic vector field on
counterexample to the Seifert conjecture (in its original formulation) was found by K. Kuperberg [a5] the same year. This aperiodic vector field on 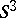 also employs a plug. A partial self-insertion performed on a Wilson-type plug breaks the periodic orbits in the plug itself in a recursive process, see Fig.a4.
also employs a plug. A partial self-insertion performed on a Wilson-type plug breaks the periodic orbits in the plug itself in a recursive process, see Fig.a4.
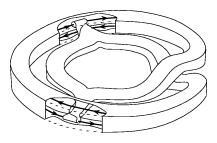
Figure: s130200d
The K-plug — a self-inserted Wilson plug
The following year, G. Kuperberg [a1] modified Schweitzer's vector field to a volume-preserving counterexample to the Seifert conjecture, thereby narrowing the gap between the counterexamples and Hofer's theorem.
The above constructions generalize to higher dimensions, but counterexamples with stronger properties exist in dimensions above three. The Hamiltonian version of the Seifert conjecture is false for 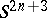 for
for 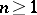 , as V.L. Ginzburg [a2] proved that there is a smooth function
, as V.L. Ginzburg [a2] proved that there is a smooth function  ,
,  , such that the Hamiltonian flow of
, such that the Hamiltonian flow of 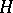 on
on  has no closed orbits (cf. also Hamiltonian system).
has no closed orbits (cf. also Hamiltonian system).
Modified Seifert conjecture.
A minimal set of a dynamical system is an invariant, non-empty, compact set containing no proper invariant, non-empty, compact subsets. The modified Seifert conjecture [a7], [a9] asserts that every non-singular 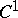 vector field on an odd-dimensional sphere
vector field on an odd-dimensional sphere 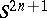 ,
, 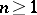 , has a minimal set of codimension at least two, i.e. of dimension at most
, has a minimal set of codimension at least two, i.e. of dimension at most  . The invariant sets in the three-dimensional plugs of Wilson and Schweitzer are one-dimensional. In 1996 it was shown [a6] that the modified Seifert conjecture is false for real-analytic as well as for piecewise-linear flows, for all odd-dimensional spheres: Every non-singular vector field on any manifold can be modified in the given smoothness category so that every minimal set is of codimension one.
. The invariant sets in the three-dimensional plugs of Wilson and Schweitzer are one-dimensional. In 1996 it was shown [a6] that the modified Seifert conjecture is false for real-analytic as well as for piecewise-linear flows, for all odd-dimensional spheres: Every non-singular vector field on any manifold can be modified in the given smoothness category so that every minimal set is of codimension one.
References
| [a1] | G. Kuperberg, "A volume-preserving counterexample to the Seifert conjecture" Comment. Math. Helv. , 71 (1996) pp. 70–97 MR1371679 Zbl 0859.57017 |
| [a2] | V.L. Ginzburg, "A smooth counterexample to the Hamiltonian Seifert conjecture in 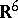 " Internat. Math. Res. Notices , 13 (1997) pp. 641–650 MR1459629 Zbl 0913.58022 " Internat. Math. Res. Notices , 13 (1997) pp. 641–650 MR1459629 Zbl 0913.58022 |
| [a3] | J. Harrison, " counterexamples to the Seifert conjecture" Topology , 27 (1988) pp. 249–278 MR0963630 Zbl 0669.57011 counterexamples to the Seifert conjecture" Topology , 27 (1988) pp. 249–278 MR0963630 Zbl 0669.57011 |
| [a4] | H. Hofer, "Pseudoholomorphic curves in symplectizations with applications to the Weinstein conjecture in dimension three" Invent. Math. , 114 (1993) pp. 515–563 MR1244912 Zbl 0797.58023 |
| [a5] | K. Kuperberg, "A smooth counterexample to the Seifert conjecture" Ann. of Math. , 140 (1994) pp. 723–732 MR1307902 Zbl 0856.57024 |
| [a6] | G. Kuperberg, K. Kuperberg, "Generalized counterexamples to the Seifert conjecture" Ann. of Math. , 144 (1996) pp. 239–268 MR1418899 MR1394969 Zbl 0856.57026 |
| [a7] | P.A. Schweitzer, "Counterexamples to the Seifert conjecture and opening closed leaves of foliations" Ann. of Math. , 100 (1974) pp. 386–400 MR0356086 Zbl 0295.57010 |
| [a8] | H. Seifert, "Closed integral curves in  -space and isotopic two-dimensional deformations" Proc. Amer. Math. Soc. , 1 (1950) pp. 287–302 MR37508 -space and isotopic two-dimensional deformations" Proc. Amer. Math. Soc. , 1 (1950) pp. 287–302 MR37508 |
| [a9] | F.W. Wilson, "On the minimal sets of non-singular vector fields" Ann. of Math. , 84 (1966) pp. 529–536 MR0202155 Zbl 0156.43803 |
Seifert conjecture. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Seifert_conjecture&oldid=12487