Difference between revisions of "Singularities of differentiable mappings"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 15: | Line 15: | ||
The questions a) and b) and many others in the theory of singularities are studied along the following lines: | The questions a) and b) and many others in the theory of singularities are studied along the following lines: | ||
| − | 1) a set of | + | 1) a set of "untypical" and "pathological" mappings is excluded from consideration; |
| − | 2) a criterion of | + | 2) a criterion of "typicality" of a mapping is determined; |
| − | 3) it is ascertained that every mapping can be approximated by | + | 3) it is ascertained that every mapping can be approximated by "typical" mappings; |
| − | 4) the | + | 4) the "typical" mappings are studied. |
The choice of the set of typical mappings depends on the problem to be solved and is not unique: the fewer the mappings that are typical, the easier they are to study, although 2) and 3) require that the set of typical mappings is sufficiently broad and sufficiently constructively defined. | The choice of the set of typical mappings depends on the problem to be solved and is not unique: the fewer the mappings that are typical, the easier they are to study, although 2) and 3) require that the set of typical mappings is sufficiently broad and sufficiently constructively defined. | ||
| Line 35: | Line 35: | ||
===Germs of differentiable mappings.=== | ===Germs of differentiable mappings.=== | ||
| − | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s0856209.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562010.png" /> be smooth manifolds, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562011.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562012.png" />. (Hereafter, the term | + | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s0856209.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562010.png" /> be smooth manifolds, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562011.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562012.png" />. (Hereafter, the term "smooth" will be used as a synonym for infinitely differentiable.) An equivalence class of mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562013.png" /> which coincide in a certain neighbourhood of the point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562014.png" /> is called a germ at the point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562015.png" />; the set of germs of mappings which transfer <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562016.png" /> to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562017.png" /> is denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562018.png" />. The group of germs of smooth changes of variables in <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562019.png" /> which preserve the point <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562020.png" /> is denoted by <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s08562021.png" />. |
An important local problem in the theory of singularities of differentiable mappings is the study of the natural action of the group | An important local problem in the theory of singularities of differentiable mappings is the study of the natural action of the group | ||
| Line 90: | Line 90: | ||
A smooth mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620143.png" /> of smooth manifolds is said to be stable if for any mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620144.png" /> that is sufficiently close to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620145.png" /> there exists diffeomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620146.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620147.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620148.png" />. | A smooth mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620143.png" /> of smooth manifolds is said to be stable if for any mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620144.png" /> that is sufficiently close to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620145.png" /> there exists diffeomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620146.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620147.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620148.png" />. | ||
| − | For small <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620149.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620150.png" />), as well as for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620151.png" /> and any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620152.png" />, stable differentiable mappings are dense in the space of all proper differentiable mappings [[#References|[3]]]. In the space of mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620153.png" />, stable mappings do not form an everywhere-dense set (see [[#References|[1]]]). For certain pairs of manifolds (for example, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620154.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620155.png" />) there is no stable mapping from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620156.png" /> into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620157.png" />. All | + | For small <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620149.png" /> (<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620150.png" />), as well as for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620151.png" /> and any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620152.png" />, stable differentiable mappings are dense in the space of all proper differentiable mappings [[#References|[3]]]. In the space of mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620153.png" />, stable mappings do not form an everywhere-dense set (see [[#References|[1]]]). For certain pairs of manifolds (for example, for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620154.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620155.png" />) there is no stable mapping from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620156.png" /> into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620157.png" />. All "stable dimensions" <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620158.png" /> have been found in [[#References|[14]]], : For any smooth manifolds <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620159.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620160.png" />, the stable mappings from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620161.png" /> into <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620162.png" /> are dense in the space of proper differentiable mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620163.png" />, equipped with the Whitney <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620164.png" />-topology, if and only if the pair <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620165.png" /> satisfies at least one of the following conditions <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620166.png" />: a) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620167.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620168.png" />; b) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620169.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620170.png" />; c) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620171.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620172.png" />; d) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620173.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620174.png" />; e) <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620175.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620176.png" />. |
| − | In proving this theorem, as well as in many other questions, the following two concepts prove to be useful: A mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620177.png" /> is called homotopy stable if for any smooth homotopy <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620178.png" /> of the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620179.png" /> there are smooth homotopies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620180.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620181.png" /> of the identity diffeomorphisms of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620182.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620183.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620184.png" /> for sufficiently small <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620185.png" />. A mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620186.png" /> is called infinitesimal stable if every mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620187.png" /> that is infinitely close to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620188.png" /> can be obtained from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620189.png" /> by diffeomorphisms of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620190.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620191.png" /> which are | + | In proving this theorem, as well as in many other questions, the following two concepts prove to be useful: A mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620177.png" /> is called homotopy stable if for any smooth homotopy <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620178.png" /> of the mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620179.png" /> there are smooth homotopies <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620180.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620181.png" /> of the identity diffeomorphisms of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620182.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620183.png" /> such that <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620184.png" /> for sufficiently small <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620185.png" />. A mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620186.png" /> is called infinitesimal stable if every mapping <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620187.png" /> that is infinitely close to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620188.png" /> can be obtained from <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620189.png" /> by diffeomorphisms of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620190.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620191.png" /> which are "infinitely close to the identity" . For a proper mapping, the concepts of stability, homotopy stability and infinitesimal stability coincide [[#References|[3]]]. The problem of finding local normal forms of stable mappings reduces to the problem of classifying certain finite-dimensional local algebras [[#References|[14]]], . For fixed <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620192.png" />, the number of such normal forms is finite. |
If in the definition of a stable mapping, homeomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620193.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620194.png" /> are taken instead of diffeomorphisms, then the definition of a topologically stable mapping is obtained. The theorem on the density of the set of topological stable mappings in the set of all mappings of any compact manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620195.png" /> into any manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620196.png" /> (for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620197.png" />) has been proved (see [[#References|[8]]]). | If in the definition of a stable mapping, homeomorphisms <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620193.png" /> and <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620194.png" /> are taken instead of diffeomorphisms, then the definition of a topologically stable mapping is obtained. The theorem on the density of the set of topological stable mappings in the set of all mappings of any compact manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620195.png" /> into any manifold <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620196.png" /> (for any <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620197.png" />) has been proved (see [[#References|[8]]]). | ||
| Line 99: | Line 99: | ||
Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620198.png" /> be some equivalence relation on the set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620199.png" /> of germs of mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620200.png" /> which take <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620201.png" /> to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620202.png" />. The <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620203.png" />-jet of any such germ is the segment of order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620204.png" /> of its Taylor series. A germ <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620205.png" /> is said to be <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620207.png" />-defined if any other germ <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620208.png" /> with the same <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620209.png" />-jet satisfies the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620210.png" />. A germ is finitely defined if it is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620211.png" />-defined for a certain <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620212.png" />. A <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620213.png" />-jet <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620214.png" /> such that any two germs <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620215.png" /> which have <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620216.png" /> as <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620217.png" />-jet satisfy the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620218.png" />, is said to be sufficient. The most commonly encountered equivalences have special names: | Let <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620198.png" /> be some equivalence relation on the set <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620199.png" /> of germs of mappings <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620200.png" /> which take <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620201.png" /> to <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620202.png" />. The <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620203.png" />-jet of any such germ is the segment of order <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620204.png" /> of its Taylor series. A germ <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620205.png" /> is said to be <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620207.png" />-defined if any other germ <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620208.png" /> with the same <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620209.png" />-jet satisfies the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620210.png" />. A germ is finitely defined if it is <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620211.png" />-defined for a certain <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620212.png" />. A <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620213.png" />-jet <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620214.png" /> such that any two germs <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620215.png" /> which have <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620216.png" /> as <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620217.png" />-jet satisfy the relation <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620218.png" />, is said to be sufficient. The most commonly encountered equivalences have special names: | ||
| − | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620221.png" />-equivalence — belonging to one orbit of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620222.png" /> of | + | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620221.png" />-equivalence — belonging to one orbit of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620222.png" /> of "correct" changes of coordinates. |
<img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620224.png" />-equivalence — belonging to one orbit of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620225.png" />. | <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620224.png" />-equivalence — belonging to one orbit of the group <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620225.png" />. | ||
| Line 153: | Line 153: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> V.I. Arnol'd, S.M. [S.M. Khusein-Zade] Gusein-Zade, A.N. Varchenko, "Singularities of differentiable maps" , '''1–2''' , Birkhäuser (1985–1988) (Translated from Russian) {{MR|}} {{ZBL|0659.58002}} {{ZBL|0554.58001}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) {{MR|}} {{ZBL|0692.70003}} {{ZBL|0572.70001}} {{ZBL|0647.70001}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> M. Golubitskii, V. Guillemin, "Stable mappings and their singularities" , Springer (1973) {{MR|0467801}} {{ZBL|}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> P. Bröcker, L. Lander, "Differentiable germs and catastrophes" , Cambridge Univ. Press (1975) {{MR|0494220}} {{ZBL|0302.58006}} </TD></TR><TR><TD valign="top">[5]</TD> <TD valign="top"> T. Poston, I. Stewart, "Catastrophe theory and its applications" , Pitman (1978) {{MR|0501079}} {{ZBL|0382.58006}} </TD></TR><TR><TD valign="top">[6]</TD> <TD valign="top"> J. Milnor, "Singular points of complex hypersurfaces" , Princeton Univ. Press (1968) {{MR|0239612}} {{ZBL|0184.48405}} </TD></TR><TR><TD valign="top">[7]</TD> <TD valign="top"> , ''Singularities of differentiable mappings'' , Moscow (1968) (In Russian; translated from English and French) {{MR|}} {{ZBL|0933.57028}} {{ZBL|0921.00024}} {{ZBL|0589.58004}} {{ZBL|0545.58001}} {{ZBL|0513.58001}} {{ZBL|0462.00012}} {{ZBL|0299.35043}} {{ZBL|0288.35020}} {{ZBL|0216.45803}} {{ZBL|0216.20105}} {{ZBL|0178.26903}} {{ZBL|0178.26902}} {{ZBL|0061.37005}} </TD></TR><TR><TD valign="top">[8]</TD> <TD valign="top"> C.G. Gibson, K. Wirthmüller, A.A. du Plessis, E.J.N. Looijenga, "Topological stability of smooth mappings" , ''Lect. notes in math.'' , '''552''' , Springer (1976) {{MR|0436203}} {{ZBL|0377.58006}} </TD></TR><TR><TD valign="top">[9]</TD> <TD valign="top"> R. Thom, "Structural stability and morphogenesis" , Benjamin (1966) (Translated from French) {{MR|1042988}} {{MR|0488156}} {{ZBL|0698.92001}} {{ZBL|0392.92001}} {{ZBL|0303.92002}} </TD></TR><TR><TD valign="top">[10]</TD> <TD valign="top"> V.I. Arnol'd, "Normal forms for functions near degenerate critical points, the Weyl groups <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620329.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620330.png" />, <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620331.png" /> and Lagrangian singularities" ''Funct. Anal. Appl.'' , '''6''' : 4 (1972) pp. 254–272 ''Funkts. Anal. i Prilozhen.'' , '''6''' : 4 (1972) pp. 3–25</TD></TR><TR><TD valign="top">[11a]</TD> <TD valign="top"> V.I. Arnol'd, "Lectures on bifurcation in versal families" ''Russian Math. Surveys'' , '''27''' : 5 (1972) pp. 54–123 ''Uspekhi Mat. Nauk'' , '''27''' : 5 (1972) pp. 119–184</TD></TR><TR><TD valign="top">[11b]</TD> <TD valign="top"> V.I. Arnol'd, "Remarks on the stationary phase method and Coxeter numbers" ''Russian Math. Surveys'' , '''28''' : 5 (1973) pp. 19–48 ''Uspekhi Mat. Nauk'' , '''28''' : 5 (1973) pp. 17–44 {{MR|}} {{ZBL|0291.40005}} </TD></TR><TR><TD valign="top">[11c]</TD> <TD valign="top"> V.I. Arnol'd, "Normal forms of functions in a neighbourhood of a degenerate critical point" ''Russian Math. Surveys'' , '''29''' : 2 (1974) pp. 1–5 ''Uspekhi Mat. Nauk'' , '''29''' : 2 (1974) pp. 11–49</TD></TR><TR><TD valign="top">[11d]</TD> <TD valign="top"> V.I. Arnol'd, "Critical points of smooth functions and their normal forms" ''Russian Math. Surveys'' , '''30''' : 5 (1975) pp. 1–75 ''Uspekhi Mat. Nauk'' , '''30''' : 5 (1975) pp. 3–65 {{MR|}} {{ZBL|0343.58001}} </TD></TR><TR><TD valign="top">[12]</TD> <TD valign="top"> R. Thom, "The bifurcation subspace of a set of maps" N.H. Kuiper (ed.) , ''Manifolds (Amsterdam, 1970)'' , ''Lect. notes in math.'' , '''197''' , Springer (1971) pp. 202–208</TD></TR><TR><TD valign="top">[13a]</TD> <TD valign="top"> A.N. Varchenko, "Local topological properties of differentiable mappings" ''Math. USSR Izv.'' , '''8''' : 5 (1974) pp. 1033–1082 ''Izv. Akad. Nauk SSSR'' , '''38''' : 5 (1974) pp. 1037–1090 {{MR|}} {{ZBL|0313.58009}} </TD></TR><TR><TD valign="top">[13b]</TD> <TD valign="top"> A.N. Varchenko, "A theorem on topological versal deformations" ''Math. USSR Izv.'' , '''9''' : 2 (1975) pp. 277–296 ''Izv. Akad. Nauk SSSR'' , '''39''' : 2 (1975) pp. 294–314 {{MR|}} {{ZBL|0333.32005}} </TD></TR><TR><TD valign="top">[14]</TD> <TD valign="top"> J. Mather, "Stability of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620332.png" />-mappings III" ''Publ. Math. IHES'' , '''35''' (1969) pp. 127–156; 279–308</TD></TR><TR><TD valign="top">[15a]</TD> <TD valign="top"> J. Mather, "Stability of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620333.png" />-mappings IV" ''Publ. Math. IHES'' , '''37''' (1970) pp. 223–248</TD></TR><TR><TD valign="top">[15b]</TD> <TD valign="top"> J. Mather, "Stability of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620334.png" />-mappings V" ''Adv. Math.'' , '''4''' (1970) pp. 301–335 {{MR|275461}} {{ZBL|}} </TD></TR><TR><TD valign="top">[15c]</TD> <TD valign="top"> J. Mather, "Stability of <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/s/s085/s085620/s085620335.png" />-mappings VI" C.T.C. Wall (ed.) , ''Proc. Liverpool Singularities Symposium I'' , ''Lect. notes in math.'' , '''192''' , Springer (1971) pp. 207–253 {{MR|293670}} {{ZBL|}} </TD></TR></table> |
| Line 161: | Line 161: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> V.I. Arnol'd, "Singularities of caustics and wave fronts" , Kluwer (1990) {{MR|}} {{ZBL|0734.53001}} </TD></TR></table> |
Revision as of 17:34, 31 March 2012
A branch of mathematical analysis and differential geometry, in which those properties of mappings are studied which are preserved when the coordinates in the image and pre-image of the mapping are changed (or when changes are made which preserve certain supplementary structures); a general approach is proposed to the solution of various problems on degeneration of mappings, functions, vector fields, etc.; a classification is given of the most commonly encountered degenerations, and their normal forms, as well as algorithms which reduce to the normal forms, are determined.
A point of the domain of definition of a differentiable mapping (i.e. a mapping of class 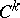 , see Differentiable manifold) is said to be regular if the Jacobi matrix has maximum rank at this point, and critical in the opposite case.
, see Differentiable manifold) is said to be regular if the Jacobi matrix has maximum rank at this point, and critical in the opposite case.
The classical implicit function theorem describes the structure of a mapping in a neighbourhood of a regular point; in a neighbourhood of this point and in a neighbourhood of its image, there exist coordinates in which the mapping is linear.
In many cases it is not sufficient to confine the area of study simply to regular points; it is therefore natural to consider the following questions:
a) the description of a mapping in a neighbourhood of a critical point;
b) the description of the structure of the set of critical points.
For an arbitrary mapping there are no answers to a) and b), for two reasons: In attempting to deal with all mappings, there is no chance of obtaining explicit results (for example, the set of critical points can locally be an arbitrary closed set), and for practical applications it is sufficient to know the answers for only a large set of mappings.
The questions a) and b) and many others in the theory of singularities are studied along the following lines:
1) a set of "untypical" and "pathological" mappings is excluded from consideration;
2) a criterion of "typicality" of a mapping is determined;
3) it is ascertained that every mapping can be approximated by "typical" mappings;
4) the "typical" mappings are studied.
The choice of the set of typical mappings depends on the problem to be solved and is not unique: the fewer the mappings that are typical, the easier they are to study, although 2) and 3) require that the set of typical mappings is sufficiently broad and sufficiently constructively defined.
This scheme is illustrated by the Whitney theorem: Every differentiable mapping 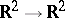 can be approximated by a mapping
can be approximated by a mapping 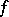 such that for any point
such that for any point 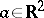 in neighbourhoods of
in neighbourhoods of  and
and 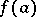 coordinates can be chosen at which the mapping
coordinates can be chosen at which the mapping  has one of the three normal forms:
has one of the three normal forms:
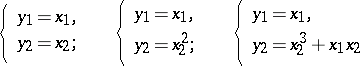 |
(for the typicality criterion, see [3], [4]). The work of H. Whitney (1955), in which this theorem was proved, is considered to be the beginning of the theory of singularities of differentiable mappings, although a number of individual results appeared far earlier (Morse theory of critical points of functions, Whitney's theorem on singularities of imbeddings, the work of L.S. Pontryagin on the connection between singularities and characteristic classes).
Basic concepts in the theory of singularities of differentiable mappings.
Germs of differentiable mappings.
Let 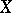 and
and 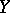 be smooth manifolds,
be smooth manifolds, 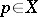 ,
, 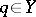 . (Hereafter, the term "smooth" will be used as a synonym for infinitely differentiable.) An equivalence class of mappings
. (Hereafter, the term "smooth" will be used as a synonym for infinitely differentiable.) An equivalence class of mappings  which coincide in a certain neighbourhood of the point
which coincide in a certain neighbourhood of the point 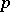 is called a germ at the point
is called a germ at the point  ; the set of germs of mappings which transfer
; the set of germs of mappings which transfer 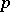 to
to 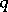 is denoted by
is denoted by 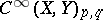 . The group of germs of smooth changes of variables in
. The group of germs of smooth changes of variables in 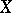 which preserve the point
which preserve the point 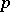 is denoted by
is denoted by 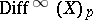 .
.
An important local problem in the theory of singularities of differentiable mappings is the study of the natural action of the group
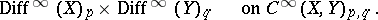 |
The solution of this and many similar problems is usually begun by approximating the function spaces and the infinite-dimensional groups acting on them by finite-dimensional manifolds and the actions on them by Lie groups. The results obtained are then transferred to the initial infinite-dimensional situation.
Jet bundles.
Let 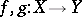 be smooth mappings, and let
be smooth mappings, and let 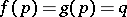 ; the mappings
; the mappings 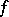 and
and 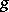 have, by definition, contact of order
have, by definition, contact of order 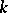 at the point
at the point 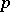 if their Taylor series at this point coincide up to the order
if their Taylor series at this point coincide up to the order 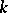 . An equivalence class of mappings which have contact of order
. An equivalence class of mappings which have contact of order 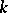 at the point
at the point 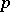 is called a
is called a 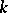 -jet. The set of all
-jet. The set of all 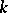 -jets of mappings which transfer
-jets of mappings which transfer  to
to  has the natural structure of a smooth manifold and is denoted by
has the natural structure of a smooth manifold and is denoted by 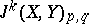 . There is a well-defined natural projection
. There is a well-defined natural projection
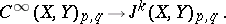 |
The equivalence class of smooth changes of variables in  which preserve the point
which preserve the point 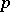 and have contact of order
and have contact of order 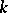 at this point is called an invertible
at this point is called an invertible 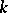 -jet at
-jet at 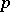 . The invertible
. The invertible 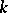 -jets form a Lie group
-jets form a Lie group 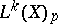 . The Lie group
. The Lie group  acts on
acts on 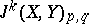 and approximates the action of
and approximates the action of
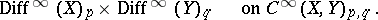 |
Let  . The set
. The set 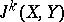 has the natural structure of a smooth bundle over
has the natural structure of a smooth bundle over 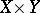 with fibre
with fibre
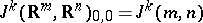 |
and structure group
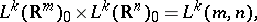 |
where  ,
, 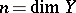 .
.
Singularities and classes of singularities.
An orbit of the action of 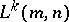 on
on  is called a
is called a 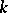 -singularity; any subset in
-singularity; any subset in  that is invariant under
that is invariant under 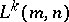 is called a class of
is called a class of 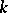 -singularities. Let
-singularities. Let 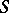 be such a class. Because
be such a class. Because 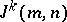 can be identified with
can be identified with 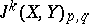 , the subset
, the subset 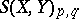 is defined in
is defined in 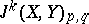 , irrespective of the method of identification. The set
, irrespective of the method of identification. The set 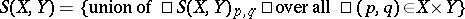 is called a universal class of singularities (or a universal singularity if
is called a universal class of singularities (or a universal singularity if 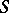 is a singularity). A universal singularity
is a singularity). A universal singularity 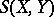 is a submanifold in
is a submanifold in 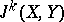 of codimension equal to the codimension of
of codimension equal to the codimension of 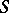 in
in 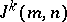 .
.
Let 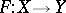 be a smooth mapping. The smooth mapping
be a smooth mapping. The smooth mapping 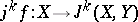 , called the
, called the 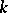 -jet extension of
-jet extension of 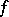 , is obtained by assigning to each point
, is obtained by assigning to each point  the
the 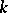 -jet of
-jet of 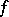 at
at  . The mapping
. The mapping 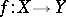 has, by definition, a singularity of type
has, by definition, a singularity of type  at the point
at the point 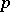 if
if 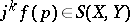 . The set
. The set 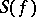 of all points at which
of all points at which 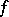 has a singularity of type
has a singularity of type 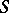 is none other than
is none other than 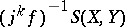 . The study of the set
. The study of the set 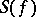 thus divides into two stages: the study of the universal set
thus divides into two stages: the study of the universal set 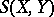 in
in 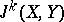 , which reduces to the study of
, which reduces to the study of  in
in  , and the study of the mutual positions of
, and the study of the mutual positions of 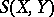 and
and  . In the second stage one usually employs Thom's transversality theorem.
. In the second stage one usually employs Thom's transversality theorem.
Transversality.
A smooth mapping 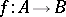 of smooth manifolds is transversal to a submanifold
of smooth manifolds is transversal to a submanifold 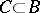 (denoted
(denoted 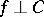 ) if for any point
) if for any point 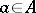 either
either 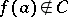 or
or 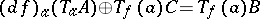 . If
. If 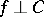 , then the set
, then the set  is either empty or is a submanifold in
is either empty or is a submanifold in  of codimension equal to the codimension of
of codimension equal to the codimension of 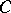 in
in 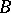 .
.
Thom's transversality theorem: Let 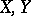 be smooth manifolds and let
be smooth manifolds and let 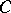 be a submanifold in
be a submanifold in 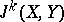 ; then the set of those
; then the set of those 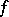 for which
for which 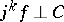 is a massive subset in
is a massive subset in 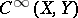 in the Whitney
in the Whitney 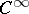 -topology. (A set is said to be massive (generic) if it is the intersection of a countable number of open dense subsets. A property is called generic if it holds for all
-topology. (A set is said to be massive (generic) if it is the intersection of a countable number of open dense subsets. A property is called generic if it holds for all 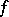 in a generic subset.)
in a generic subset.)
The Whitney topology. Let 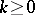 and let
and let 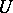 be an open set in
be an open set in 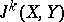 . Let
. Let
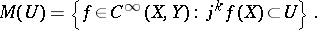 |
The sets 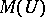 form the basis of some topology, called the Whitney
form the basis of some topology, called the Whitney  -topology, on
-topology, on  . In this topology,
. In this topology,  is a Baire space, i.e. every massive subset is dense.
is a Baire space, i.e. every massive subset is dense.
Multi-jets.
In the study of the self-intersection of the image of a smooth mapping, the concept of a multi-jet is used. Let 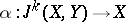 be the natural projection. Let
be the natural projection. Let
 |
and let 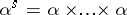 (
( times). The set
times). The set 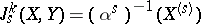 may be given the natural structure of a smooth manifold and is called an
may be given the natural structure of a smooth manifold and is called an  -fold bundle of
-fold bundle of 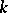 -jets. For
-jets. For  -fold jets, the
-fold jets, the 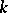 -jet extension of a mapping
-jet extension of a mapping 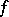 ,
, 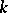 -singularities, universal singularities, etc., are defined, and the analogue of Thom's transversality theorem has been proved.
-singularities, universal singularities, etc., are defined, and the analogue of Thom's transversality theorem has been proved.
Stable differentiable mappings.
A central problem in the early days of the theory of singularities of differentiable mappings was the study of stable differentiable mappings.
A smooth mapping 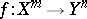 of smooth manifolds is said to be stable if for any mapping
of smooth manifolds is said to be stable if for any mapping 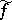 that is sufficiently close to
that is sufficiently close to 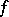 there exists diffeomorphisms
there exists diffeomorphisms 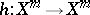 and
and  such that
such that 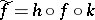 .
.
For small 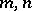 (
( ), as well as for
), as well as for  and any
and any 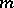 , stable differentiable mappings are dense in the space of all proper differentiable mappings [3]. In the space of mappings
, stable differentiable mappings are dense in the space of all proper differentiable mappings [3]. In the space of mappings 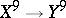 , stable mappings do not form an everywhere-dense set (see [1]). For certain pairs of manifolds (for example, for
, stable mappings do not form an everywhere-dense set (see [1]). For certain pairs of manifolds (for example, for  ,
, 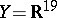 ) there is no stable mapping from
) there is no stable mapping from  into
into 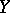 . All "stable dimensions"
. All "stable dimensions" 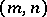 have been found in [14], : For any smooth manifolds
have been found in [14], : For any smooth manifolds  and
and 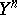 , the stable mappings from
, the stable mappings from 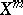 into
into 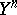 are dense in the space of proper differentiable mappings
are dense in the space of proper differentiable mappings 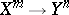 , equipped with the Whitney
, equipped with the Whitney 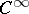 -topology, if and only if the pair
-topology, if and only if the pair 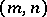 satisfies at least one of the following conditions
satisfies at least one of the following conditions 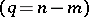 : a)
: a) 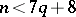 and
and  ; b)
; b) 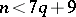 and
and 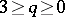 ; c)
; c) 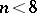 and
and 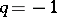 ; d)
; d) 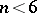 and
and 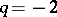 ; e)
; e) 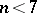 and
and  .
.
In proving this theorem, as well as in many other questions, the following two concepts prove to be useful: A mapping 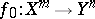 is called homotopy stable if for any smooth homotopy
is called homotopy stable if for any smooth homotopy 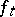 of the mapping
of the mapping 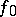 there are smooth homotopies
there are smooth homotopies 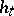 and
and 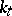 of the identity diffeomorphisms of
of the identity diffeomorphisms of 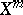 and
and 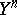 such that
such that  for sufficiently small
for sufficiently small 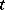 . A mapping
. A mapping 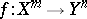 is called infinitesimal stable if every mapping
is called infinitesimal stable if every mapping 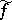 that is infinitely close to
that is infinitely close to 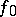 can be obtained from
can be obtained from  by diffeomorphisms of
by diffeomorphisms of 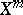 and
and 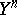 which are "infinitely close to the identity" . For a proper mapping, the concepts of stability, homotopy stability and infinitesimal stability coincide [3]. The problem of finding local normal forms of stable mappings reduces to the problem of classifying certain finite-dimensional local algebras [14], . For fixed
which are "infinitely close to the identity" . For a proper mapping, the concepts of stability, homotopy stability and infinitesimal stability coincide [3]. The problem of finding local normal forms of stable mappings reduces to the problem of classifying certain finite-dimensional local algebras [14], . For fixed 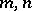 , the number of such normal forms is finite.
, the number of such normal forms is finite.
If in the definition of a stable mapping, homeomorphisms 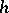 and
and 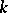 are taken instead of diffeomorphisms, then the definition of a topologically stable mapping is obtained. The theorem on the density of the set of topological stable mappings in the set of all mappings of any compact manifold
are taken instead of diffeomorphisms, then the definition of a topologically stable mapping is obtained. The theorem on the density of the set of topological stable mappings in the set of all mappings of any compact manifold 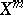 into any manifold
into any manifold 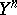 (for any
(for any 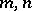 ) has been proved (see [8]).
) has been proved (see [8]).
Finitely defined germs.
Let 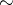 be some equivalence relation on the set
be some equivalence relation on the set 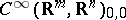 of germs of mappings
of germs of mappings  which take
which take  to
to  . The
. The 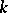 -jet of any such germ is the segment of order
-jet of any such germ is the segment of order  of its Taylor series. A germ
of its Taylor series. A germ  is said to be
is said to be 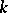 -defined if any other germ
-defined if any other germ 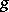 with the same
with the same 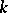 -jet satisfies the relation
-jet satisfies the relation 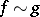 . A germ is finitely defined if it is
. A germ is finitely defined if it is 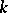 -defined for a certain
-defined for a certain 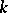 . A
. A 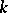 -jet
-jet 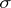 such that any two germs
such that any two germs 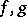 which have
which have 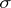 as
as 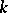 -jet satisfy the relation
-jet satisfy the relation 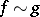 , is said to be sufficient. The most commonly encountered equivalences have special names:
, is said to be sufficient. The most commonly encountered equivalences have special names:
 -equivalence — belonging to one orbit of the group
-equivalence — belonging to one orbit of the group 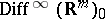 of "correct" changes of coordinates.
of "correct" changes of coordinates.
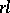 -equivalence — belonging to one orbit of the group
-equivalence — belonging to one orbit of the group 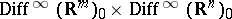 .
.
Topological equivalence — belonging to one orbit of the group 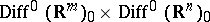 .
.
The study of 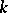 -defined germs reduces to the study of mappings defined by polynomials of degree
-defined germs reduces to the study of mappings defined by polynomials of degree 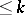 .
.
The question of whether a germ is 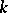 -defined with respect to
-defined with respect to 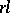 -equivalence reduces to the problem of the solvability of an explicit system of finitely many linear equations.
-equivalence reduces to the problem of the solvability of an explicit system of finitely many linear equations.
The set of finitely defined germs with respect to  -equivalence is open in
-equivalence is open in 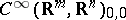 , but is not dense for any
, but is not dense for any 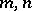 . It is natural to consider the coarser relation of topological equivalence. After rejection of a subset of finite codimension from
. It is natural to consider the coarser relation of topological equivalence. After rejection of a subset of finite codimension from 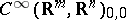 , a countable number of topological equivalence classes remains, each of which is a semi-algebraic set. It follows that the mappings with germs that are topologically equivalent to polynomial ones form an open dense set in
, a countable number of topological equivalence classes remains, each of which is a semi-algebraic set. It follows that the mappings with germs that are topologically equivalent to polynomial ones form an open dense set in 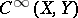 (
(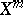 is compact) .
is compact) .
Deformation.
If a mapping depends on parameters, then a family of mappings is said to be defined. If the family of mappings is studied locally, then when the parameters are changed slightly in a neighbourhood of fixed values, one speaks of a deformation of the mapping corresponding to these fixed values of the parameters. In many cases, the study of all possible deformations reduces to the study of a single natural deformation, from which all others are obtained. This deformation, in a sense the largest, contains all essentially different deformations of the given mapping. It is called the versal deformation (see , [12], ).
Critical points of functions.
A critical point of a function is called non-degenerate if the second differential is a non-degenerate quadratic form. A function in general position has only non-degenerate critical points, while in a neighbourhood of each of them it can be reduced to a standard form. Degenerate critical points are studied when these functions depend on parameters, and the greater the number of these parameters, the more complex critical points will be encountered that are non-removable (by a small shift) for certain values of the parameters.
A family of functions depending on any number of parameters can be transformed by a small shift into a family in which, for every value of the parameter in a neighbourhood of any point of the domain of definition, the function is represented by a polynomial in a local system of coordinates. This means that in the local study of functions it is possible to examine only polynomials and to use complex analysis.
Classification.
It is natural to begin by classifying the germs at 0 of holomorphic functions in  , taking two germs to be equivalent if one can be transferred into the other by the germ of a holomorphic change of coordinates in
, taking two germs to be equivalent if one can be transferred into the other by the germ of a holomorphic change of coordinates in  which preserves 0. A jet (Taylor polynomial) of a holomorphic function at 0 is sufficient if it defines the function up to equivalence. A germ whose critical point 0 is isolated always has a sufficient jet and, consequently, is equivalent to a polynomial. The number of non-degenerate critical points into which the critical point 0 splits when the function is shifted slightly is called the multiplicity (or Milnor number)
which preserves 0. A jet (Taylor polynomial) of a holomorphic function at 0 is sufficient if it defines the function up to equivalence. A germ whose critical point 0 is isolated always has a sufficient jet and, consequently, is equivalent to a polynomial. The number of non-degenerate critical points into which the critical point 0 splits when the function is shifted slightly is called the multiplicity (or Milnor number) 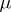 of the critical point 0. If the multiplicity of a critical point of a function
of the critical point 0. If the multiplicity of a critical point of a function 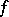 is equal to
is equal to 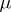 , then the
, then the 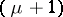 -jet is sufficient. Since the multiplicity
-jet is sufficient. Since the multiplicity 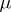 cannot increase under a small change of
cannot increase under a small change of 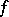 , the classification of functions close to a function with an isolated critical point reduces to the study of the action of the Lie group of
, the classification of functions close to a function with an isolated critical point reduces to the study of the action of the Lie group of 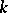 -jets of changes of variables on the space of
-jets of changes of variables on the space of 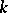 -jets for a sufficiently large
-jets for a sufficiently large 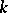 . In a space of
. In a space of  -jets of functions
-jets of functions 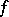 for which
for which  ,
,  , the codimension of the orbit of
, the codimension of the orbit of 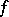 is equal to
is equal to  ; thus, the critical points of multiplicity
; thus, the critical points of multiplicity 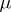 are non-removable in families of functions which depend on
are non-removable in families of functions which depend on 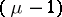 parameters. A classification (see [10]) of all critical points of multiplicity
parameters. A classification (see [10]) of all critical points of multiplicity 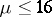 and an algorithm for reducing any such function to a normal form have been obtained. The complexity of a critical point is defined not only by its multiplicity
and an algorithm for reducing any such function to a normal form have been obtained. The complexity of a critical point is defined not only by its multiplicity 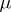 , but also by its modality
, but also by its modality 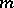 (the number of moduli). A critical point is simple (or
(the number of moduli). A critical point is simple (or  -modal) if among the critical points near to it there are at most finitely many pairwise non-equivalent points. Two germs of functions are called stably equivalent if they become equivalent after being added directly to non-degenerate quadratic forms in the appropriate number of variables (for germs of functions in the same number of variables, stable equivalence is the same ordinary equivalence).
-modal) if among the critical points near to it there are at most finitely many pairwise non-equivalent points. Two germs of functions are called stably equivalent if they become equivalent after being added directly to non-degenerate quadratic forms in the appropriate number of variables (for germs of functions in the same number of variables, stable equivalence is the same ordinary equivalence).
Simple germs are exhausted up to stable equivalence by the following list:
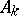 :
: 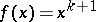 ,
,  ;
;
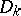 :
: 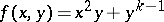 ,
, 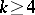 ;
;
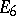 :
: 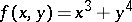 ;
;
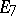 :
: 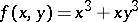 ;
;
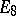 :
: 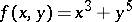 .
.
The modality of a point 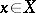 under the action of a Lie group
under the action of a Lie group 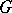 on a manifold
on a manifold 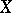 is the smallest number
is the smallest number 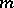 such that a sufficiently small neighbourhood of
such that a sufficiently small neighbourhood of  is covered by a finite number of
is covered by a finite number of 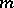 -parametric families of orbits.
-parametric families of orbits.
A classification of germs of functions of modality 1 and 2 has also been obtained (see [10]). The classification of simple singularities and singularities of small modality is related to Lie groups, the Coxeter and Weyl series 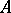 ,
,  ,
,  , the theory of Artin braids, the classification of regular polyhedra in
, the theory of Artin braids, the classification of regular polyhedra in  -dimensional space, the Kodaira classification of degenerate elliptic curves, and the classification of triangles in the Lobachevskii plane (see [10], ).
-dimensional space, the Kodaira classification of degenerate elliptic curves, and the classification of triangles in the Lobachevskii plane (see [10], ).
Boundary singularities.
A series of geometric problems requires the study of critical points of functions on a manifold with boundary.
In the complex case this situation corresponds to the study of the germ of a function defined in the space 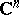 with a distinguished subspace
with a distinguished subspace 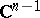 . These germs are studied up to changes of variables in
. These germs are studied up to changes of variables in 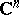 which transfer
which transfer  onto itself. In this situation, a classification has also been obtained of all simple germs, and of germs of modalities 1 and 2. The classification of simple boundary singularities proves to be related to the simple Lie algebras
onto itself. In this situation, a classification has also been obtained of all simple germs, and of germs of modalities 1 and 2. The classification of simple boundary singularities proves to be related to the simple Lie algebras  ,
, 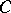 and
and 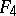 .
.
Topological characteristics of the germ of a holomorphic function.
Let 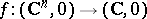 be a holomorphic function in a neighbourhood of zero, with a critical point of multiplicity
be a holomorphic function in a neighbourhood of zero, with a critical point of multiplicity 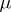 at zero. Let
at zero. Let 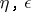 be positive numbers, let
be positive numbers, let 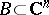 be the ball
be the ball  , let
, let 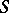 be its boundary, let
be its boundary, let  be the disc
be the disc 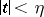 , and let
, and let 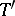 be the punctured disc
be the punctured disc 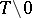 . Let
. Let 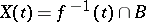 and
and 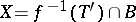 . For appropriate
. For appropriate  and
and 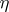 (
( is sufficiently small and
is sufficiently small and 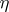 is sufficiently small in relation to
is sufficiently small in relation to  ), the mapping
), the mapping 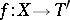 is a smooth locally trivial fibration. The fibre
is a smooth locally trivial fibration. The fibre 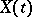 of this fibration is a
of this fibration is a  -dimensional manifold with a boundary that is homotopically equivalent to a bouquet of
-dimensional manifold with a boundary that is homotopically equivalent to a bouquet of 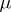
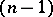 -dimensional spheres. The boundary of
-dimensional spheres. The boundary of 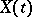 is a
is a 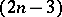 -dimensional manifold which is diffeomorphic to
-dimensional manifold which is diffeomorphic to 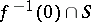 . Even for relatively simple
. Even for relatively simple 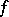 , this manifold can be non-trivial. For example, the 28 manifolds
, this manifold can be non-trivial. For example, the 28 manifolds
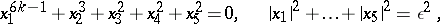 |
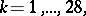 |
are 28 Milnor spheres (which are all homeomorphic to an ordinary  -dimensional sphere, but are not pairwise diffeomorphic). The reduced homology group
-dimensional sphere, but are not pairwise diffeomorphic). The reduced homology group 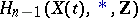 is isomorphic to
is isomorphic to 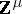 . The intersection index defines an integer-valued bilinear form on
. The intersection index defines an integer-valued bilinear form on 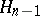 . The transfer of fibres of the fibration
. The transfer of fibres of the fibration 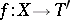 along curves in
along curves in  defines the action of the fundamental group
defines the action of the fundamental group 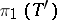 in the
in the 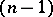 -dimensional homology space of the fibre. The automorphism of the homology group corresponding to the generator of
-dimensional homology space of the fibre. The automorphism of the homology group corresponding to the generator of 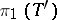 is called the monodromy operator. The monodromy operator retains the intersection form. The eigenvalues of the monodromy operator contain information on the asymptotics of various integrals related to the function
is called the monodromy operator. The monodromy operator retains the intersection form. The eigenvalues of the monodromy operator contain information on the asymptotics of various integrals related to the function  .
.
References
| [1] | V.I. Arnol'd, S.M. [S.M. Khusein-Zade] Gusein-Zade, A.N. Varchenko, "Singularities of differentiable maps" , 1–2 , Birkhäuser (1985–1988) (Translated from Russian) Zbl 0659.58002 Zbl 0554.58001 |
| [2] | V.I. Arnol'd, "Mathematical methods of classical mechanics" , Springer (1978) (Translated from Russian) Zbl 0692.70003 Zbl 0572.70001 Zbl 0647.70001 |
| [3] | M. Golubitskii, V. Guillemin, "Stable mappings and their singularities" , Springer (1973) MR0467801 |
| [4] | P. Bröcker, L. Lander, "Differentiable germs and catastrophes" , Cambridge Univ. Press (1975) MR0494220 Zbl 0302.58006 |
| [5] | T. Poston, I. Stewart, "Catastrophe theory and its applications" , Pitman (1978) MR0501079 Zbl 0382.58006 |
| [6] | J. Milnor, "Singular points of complex hypersurfaces" , Princeton Univ. Press (1968) MR0239612 Zbl 0184.48405 |
| [7] | , Singularities of differentiable mappings , Moscow (1968) (In Russian; translated from English and French) Zbl 0933.57028 Zbl 0921.00024 Zbl 0589.58004 Zbl 0545.58001 Zbl 0513.58001 Zbl 0462.00012 Zbl 0299.35043 Zbl 0288.35020 Zbl 0216.45803 Zbl 0216.20105 Zbl 0178.26903 Zbl 0178.26902 Zbl 0061.37005 |
| [8] | C.G. Gibson, K. Wirthmüller, A.A. du Plessis, E.J.N. Looijenga, "Topological stability of smooth mappings" , Lect. notes in math. , 552 , Springer (1976) MR0436203 Zbl 0377.58006 |
| [9] | R. Thom, "Structural stability and morphogenesis" , Benjamin (1966) (Translated from French) MR1042988 MR0488156 Zbl 0698.92001 Zbl 0392.92001 Zbl 0303.92002 |
| [10] | V.I. Arnol'd, "Normal forms for functions near degenerate critical points, the Weyl groups 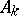 , , 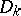 , , 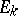 and Lagrangian singularities" Funct. Anal. Appl. , 6 : 4 (1972) pp. 254–272 Funkts. Anal. i Prilozhen. , 6 : 4 (1972) pp. 3–25 and Lagrangian singularities" Funct. Anal. Appl. , 6 : 4 (1972) pp. 254–272 Funkts. Anal. i Prilozhen. , 6 : 4 (1972) pp. 3–25 |
| [11a] | V.I. Arnol'd, "Lectures on bifurcation in versal families" Russian Math. Surveys , 27 : 5 (1972) pp. 54–123 Uspekhi Mat. Nauk , 27 : 5 (1972) pp. 119–184 |
| [11b] | V.I. Arnol'd, "Remarks on the stationary phase method and Coxeter numbers" Russian Math. Surveys , 28 : 5 (1973) pp. 19–48 Uspekhi Mat. Nauk , 28 : 5 (1973) pp. 17–44 Zbl 0291.40005 |
| [11c] | V.I. Arnol'd, "Normal forms of functions in a neighbourhood of a degenerate critical point" Russian Math. Surveys , 29 : 2 (1974) pp. 1–5 Uspekhi Mat. Nauk , 29 : 2 (1974) pp. 11–49 |
| [11d] | V.I. Arnol'd, "Critical points of smooth functions and their normal forms" Russian Math. Surveys , 30 : 5 (1975) pp. 1–75 Uspekhi Mat. Nauk , 30 : 5 (1975) pp. 3–65 Zbl 0343.58001 |
| [12] | R. Thom, "The bifurcation subspace of a set of maps" N.H. Kuiper (ed.) , Manifolds (Amsterdam, 1970) , Lect. notes in math. , 197 , Springer (1971) pp. 202–208 |
| [13a] | A.N. Varchenko, "Local topological properties of differentiable mappings" Math. USSR Izv. , 8 : 5 (1974) pp. 1033–1082 Izv. Akad. Nauk SSSR , 38 : 5 (1974) pp. 1037–1090 Zbl 0313.58009 |
| [13b] | A.N. Varchenko, "A theorem on topological versal deformations" Math. USSR Izv. , 9 : 2 (1975) pp. 277–296 Izv. Akad. Nauk SSSR , 39 : 2 (1975) pp. 294–314 Zbl 0333.32005 |
| [14] | J. Mather, "Stability of 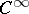 -mappings III" Publ. Math. IHES , 35 (1969) pp. 127–156; 279–308 -mappings III" Publ. Math. IHES , 35 (1969) pp. 127–156; 279–308 |
| [15a] | J. Mather, "Stability of 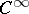 -mappings IV" Publ. Math. IHES , 37 (1970) pp. 223–248 -mappings IV" Publ. Math. IHES , 37 (1970) pp. 223–248 |
| [15b] | J. Mather, "Stability of 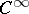 -mappings V" Adv. Math. , 4 (1970) pp. 301–335 MR275461 -mappings V" Adv. Math. , 4 (1970) pp. 301–335 MR275461 |
| [15c] | J. Mather, "Stability of 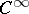 -mappings VI" C.T.C. Wall (ed.) , Proc. Liverpool Singularities Symposium I , Lect. notes in math. , 192 , Springer (1971) pp. 207–253 MR293670 -mappings VI" C.T.C. Wall (ed.) , Proc. Liverpool Singularities Symposium I , Lect. notes in math. , 192 , Springer (1971) pp. 207–253 MR293670 |
Comments
References
| [a1] | V.I. Arnol'd, "Singularities of caustics and wave fronts" , Kluwer (1990) Zbl 0734.53001 |
Singularities of differentiable mappings. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Singularities_of_differentiable_mappings&oldid=13079