Difference between revisions of "Tacnode"
From Encyclopedia of Mathematics
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 7: | Line 7: | ||
They are exemplified by the curves <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t130/t130010/t1300107.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t130/t130010/t1300108.png" />. | They are exemplified by the curves <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t130/t130010/t1300107.png" /> for <img align="absmiddle" border="0" src="https://www.encyclopediaofmath.org/legacyimages/t/t130/t130010/t1300108.png" />. | ||
| − | The terms | + | The terms "crunode" and "spinode" are seldom used nowadays (2000). |
See also [[Node|Node]]; [[Cusp(2)|Cusp]]. | See also [[Node|Node]]; [[Cusp(2)|Cusp]]. | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> A. Dimca, "Topics on real and complex singularities" , Vieweg (1987) pp. 175 {{MR|1013785}} {{ZBL|0628.14001}} </TD></TR><TR><TD valign="top">[a2]</TD> <TD valign="top"> R.J. Walker, "Algebraic curves" , Princeton Univ. Press (1950) (Reprint: Dover 1962) {{MR|0033083}} {{ZBL|0039.37701}} </TD></TR><TR><TD valign="top">[a3]</TD> <TD valign="top"> Ph. Griffiths, J. Harris, "Principles of algebraic geometry" , Wiley (1978) pp. 293; 507 {{MR|0507725}} {{ZBL|0408.14001}} </TD></TR><TR><TD valign="top">[a4]</TD> <TD valign="top"> S.S. Abhyankar, "Algebraic geometry for scientists and engineers" , Amer. Math. Soc. (1990) pp. 3; 60 {{MR|1075991}} {{ZBL|0709.14001}} {{ZBL|0721.14001}} </TD></TR></table> |
Revision as of 21:56, 30 March 2012
point of osculation, osculation point, double cusp
The third in the series of 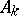 -curve singularities. The point
-curve singularities. The point 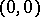 is a tacnode of the curve
is a tacnode of the curve 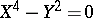 in
in 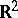 .
.
The first of the  -curve singularities are: an ordinary double point, also called a node or crunode; the cusp, or spinode; the tacnode; and the ramphoid cusp.
-curve singularities are: an ordinary double point, also called a node or crunode; the cusp, or spinode; the tacnode; and the ramphoid cusp.
They are exemplified by the curves 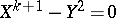 for
for  .
.
The terms "crunode" and "spinode" are seldom used nowadays (2000).
References
| [a1] | A. Dimca, "Topics on real and complex singularities" , Vieweg (1987) pp. 175 MR1013785 Zbl 0628.14001 |
| [a2] | R.J. Walker, "Algebraic curves" , Princeton Univ. Press (1950) (Reprint: Dover 1962) MR0033083 Zbl 0039.37701 |
| [a3] | Ph. Griffiths, J. Harris, "Principles of algebraic geometry" , Wiley (1978) pp. 293; 507 MR0507725 Zbl 0408.14001 |
| [a4] | S.S. Abhyankar, "Algebraic geometry for scientists and engineers" , Amer. Math. Soc. (1990) pp. 3; 60 MR1075991 Zbl 0709.14001 Zbl 0721.14001 |
How to Cite This Entry:
Tacnode. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tacnode&oldid=14597
Tacnode. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Tacnode&oldid=14597
This article was adapted from an original article by M. Hazewinkel (originator), which appeared in Encyclopedia of Mathematics - ISBN 1402006098. See original article