Multiplicity of a module

with respect to an ideal

Let 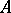be a commutative ring with unit. A module 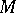over 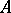is said to be of finite length if there is a sequence of submodules (a Jordan–Hölder sequence) 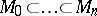such that each of the quotients 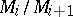, , 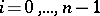is a simple 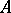-module. (The number does not depend on the sequence chosen, by the Jordan–Hölder theorem.) Now let be an -module of finite type and an ideal contained in the radical of 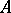and such that 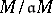is of finite length, and let be of Krull dimension . (The Krull dimension of a module is equal to the dimension of the ring 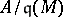where 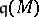is the annihilator of 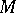, i.e. .) Then there exists a unique integer 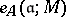such that

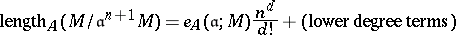for large enough. The number is called the multiplicity of 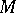with respect to . The multiplicity of an ideal is 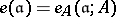. Thus, the multiplicity of the maximal ideal of a local ring of dimension 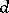is equal to 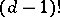times the leading coefficient of the Hilbert–Samuel polynomial of 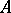, cf. Local ring.

There are some mild terminological discrepancies in the literature with respect to the Hilbert–Samuel polynomial. Let 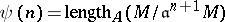and 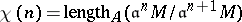. Then both 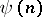and 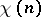are sometimes called Hilbert–Samuel functions. For both 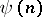and there are polynomials in (of degree and 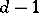, respectively) such that 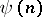and 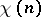coincide with these polynomials for large . Both these polynomials occur in the literature under the name Hilbert–Samuel polynomial.

For a more general set-up cf. [a1].

The multiplicity of a local ring 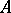is the multiplicity of its maximal ideal , .

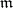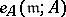References

[a1]	N. Bourbaki, "Algèbre commutative" , Masson (1983) pp. Chapt. 8, §4: Dimension MR2333539 MR2284892 MR0260715 MR0194450 MR0217051 MR0171800 Zbl 0579.13001
[a2]	M. Nagata, "Local rings" , Interscience (1962) pp. Chapt. III, §23 MR0155856 Zbl 0123.03402
[a3]	D. Mumford, "Algebraic geometry" , 1. Complex projective varieties , Springer (1976) pp. Appendix to Chapt. 6 MR0453732 Zbl 0356.14002
[a4]	O. Zariski, P. Samuel, "Commutative algebra" , 2 , v. Nostrand (1960) pp. Chapt. VIII, §10 MR0120249 Zbl 0121.27801