Difference between revisions of "Derivations, module of"
(Importing text file) |
Ulf Rehmann (talk | contribs) m (MR/ZBL numbers added) |
||
| Line 50: | Line 50: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[1]</TD> <TD valign="top"> I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) {{MR|0447223}} {{ZBL|0362.14001}} </TD></TR><TR><TD valign="top">[2]</TD> <TD valign="top"> A. Grothendieck (ed.) et al. (ed.) , ''Revêtements étales et groupe fondamental. SGA 1'' , ''Lect. notes in math.'' , '''224''' , Springer (1971) {{MR|0354651}} {{ZBL|1039.14001}} </TD></TR><TR><TD valign="top">[3]</TD> <TD valign="top"> A. Grothendieck, "Eléments de géométrie algébrique IV. Etude locale des schémes et des morphismes de schémes" ''Publ. Math. IHES'' , '''20''' (1964) {{MR|0173675}} {{ZBL|}} </TD></TR><TR><TD valign="top">[4]</TD> <TD valign="top"> E. Kähler, "Algebra und Differentialrechnung" , Deutsch. Verlag Wissenschaft. (1958) {{MR|0094593}} {{ZBL|0079.05701}} </TD></TR></table> |
| Line 58: | Line 58: | ||
====References==== | ====References==== | ||
| − | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> | + | <table><TR><TD valign="top">[a1]</TD> <TD valign="top"> R. Hartshorne, "Algebraic geometry" , Springer (1977) {{MR|0463157}} {{ZBL|0367.14001}} </TD></TR></table> |
Revision as of 21:51, 30 March 2012
module of Kähler derivations
An algebraic analogue of the concept of the differential of a function. Let 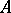 be a commutative ring regarded as an algebra over a subring
be a commutative ring regarded as an algebra over a subring 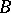 of it. The module of derivations of the
of it. The module of derivations of the 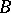 -algebra
-algebra  is defined as the quotient module
is defined as the quotient module 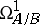 of the free
of the free  -module with basis
-module with basis 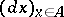 by the submodule generated by the elements of the type
by the submodule generated by the elements of the type
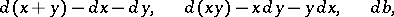 |
where 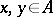 ,
, 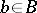 . The canonical homomorphism of
. The canonical homomorphism of 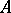 -modules
-modules 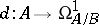 is a
is a 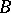 -derivation in the ring
-derivation in the ring 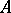 (cf. Derivation in a ring) with values in the
(cf. Derivation in a ring) with values in the  -module
-module  having the following universality property: For any
having the following universality property: For any 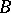 -derivation
-derivation 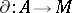 with values in an
with values in an 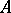 -module
-module  there exists a uniquely defined homomorphism of
there exists a uniquely defined homomorphism of 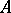 -modules
-modules 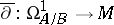 such that
such that 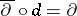 . The correspondence
. The correspondence  defines an isomorphism of
defines an isomorphism of 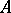 -modules:
-modules:
 |
In particular, the module of derivations of a ring  into itself is isomorphic to the dual
into itself is isomorphic to the dual 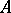 -module to the module
-module to the module 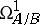 .
.
If  is regarded as an
is regarded as an 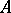 -algebra with respect to the homomorphism
-algebra with respect to the homomorphism
 |
and 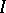 is the ideal generated by the elements of the type
is the ideal generated by the elements of the type
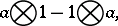 |
then the 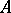 -module
-module 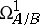 is isomorphic to the
is isomorphic to the 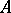 -module
-module 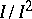 .
.
The module 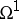 of derivations has the following properties:
of derivations has the following properties:
1) If 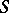 is a multiplicatively closed set in
is a multiplicatively closed set in 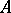 and
and 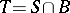 , then there is a canonical localization isomorphism:
, then there is a canonical localization isomorphism:
 |
2) If 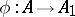 is a homomorphism of
is a homomorphism of 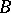 -algebras, then there is a canonical exact sequence of
-algebras, then there is a canonical exact sequence of 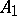 -modules:
-modules:
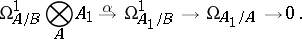 |
3) If 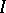 is an ideal of the ring
is an ideal of the ring 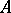 and
and  , then there is an exact canonical sequence of
, then there is an exact canonical sequence of 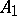 -modules:
-modules:
 |
where the homomorphism 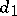 is induced by the derivation
is induced by the derivation 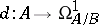 .
.
4) A field 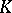 is a separable extension of a field
is a separable extension of a field 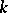 of finite transcendence degree
of finite transcendence degree  if and only if there is a
if and only if there is a 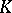 -space isomorphism
-space isomorphism  .
.
5) If 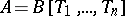 is an algebra of polynomials, then
is an algebra of polynomials, then 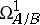 is a free
is a free  -module with as basis
-module with as basis 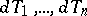 .
.
6) An algebra 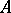 of finite type over a perfect field
of finite type over a perfect field 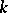 is a regular ring if and only if the
is a regular ring if and only if the 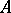 -module
-module 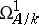 is projective.
is projective.
7) Concerning 2) above, the  -algebra
-algebra 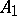 of finite type is smooth over
of finite type is smooth over 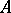 if and only if the homomorphism
if and only if the homomorphism 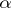 is injective while the module
is injective while the module 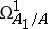 of derivations is projective and its rank is equal to the relative dimension of
of derivations is projective and its rank is equal to the relative dimension of 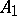 over
over 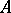 .
.
The 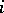 -th exterior power
-th exterior power  of the module
of the module 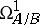 of derivations is said to be the module of (differential)
of derivations is said to be the module of (differential) 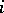 -forms of the
-forms of the 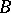 -algebra
-algebra 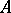 and is denoted by
and is denoted by 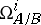 .
.
By virtue of 1) it is possible to define, for any morphism of schemes 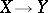 , the sheaf of relative (or Kähler) derivations
, the sheaf of relative (or Kähler) derivations 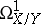 and its exterior powers
and its exterior powers 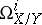 .
.
References
| [1] | I.R. Shafarevich, "Basic algebraic geometry" , Springer (1977) (Translated from Russian) MR0447223 Zbl 0362.14001 |
| [2] | A. Grothendieck (ed.) et al. (ed.) , Revêtements étales et groupe fondamental. SGA 1 , Lect. notes in math. , 224 , Springer (1971) MR0354651 Zbl 1039.14001 |
| [3] | A. Grothendieck, "Eléments de géométrie algébrique IV. Etude locale des schémes et des morphismes de schémes" Publ. Math. IHES , 20 (1964) MR0173675 |
| [4] | E. Kähler, "Algebra und Differentialrechnung" , Deutsch. Verlag Wissenschaft. (1958) MR0094593 Zbl 0079.05701 |
Comments
References
| [a1] | R. Hartshorne, "Algebraic geometry" , Springer (1977) MR0463157 Zbl 0367.14001 |
Derivations, module of. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Derivations,_module_of&oldid=18054