Difference between revisions of "Grötzsch principle"
Ulf Rehmann (talk | contribs) m (moved Grötzsch principle to Grotzsch principle: ascii title) |
Ulf Rehmann (talk | contribs) m (moved Grotzsch principle to Grötzsch principle over redirect: accented title) |
(No difference)
| |
Revision as of 07:54, 26 March 2012
A principle in the theory of conformal mapping, proposed in 1928 by H. Grötzsch
and used in proving inequalities for the lengths of curves of certain families and the area of the surface they bound. Grötzsch subsequently developed numerous applications of the strip method (cf. Strip method (analytic functions)) in the theory of univalent functions defined in finitely-connected or infinitely-connected domains.
Grötzsch' principle can be explained as follows. Let an annulus 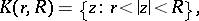
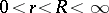 , contain a finite number of pairwise-disjoint simply-connected domains
, contain a finite number of pairwise-disjoint simply-connected domains 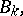
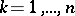 , with Jordan boundaries containing arcs
, with Jordan boundaries containing arcs 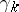 and
and  of the respective circles
of the respective circles  ,
, 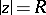 which do not degenerate into points (the
which do not degenerate into points (the 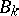 form strips which connect the boundary components of
form strips which connect the boundary components of 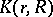 ). If
). If 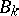 is mapped into some rectangle
is mapped into some rectangle  so that
so that 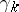 and
and 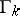 pass to sides of length
pass to sides of length  , respectively
, respectively  , then
, then
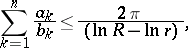 |
and equality is attained only if 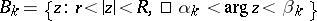 ,
, 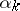 ,
,  are constants,
are constants, 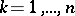 , and the union
, and the union 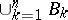 covers
covers  except for the intervals of the rays
except for the intervals of the rays 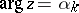 ,
, 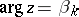 which belong to it.
which belong to it.
Grötzsch' principle and the strip method are constituent parts of the method of the extremal metric (cf. Extremal metric, method of the) and are used not only in conformal mapping, but also in mapping of a more general nature such as quasi-conformal mapping.
References
| [1a] | H. Grötzsch, "Ueber einige Extremalprobleme der konformen Abbildung I" Ber. Verh. Sächsisch. Akad. Wiss. Leipzig, Math.-Naturwiss. Kl. , 80 (1928) pp. 367–376 |
| [1b] | H. Grötzsch, "Ueber einige Extremalprobleme der konformen Abbildung II" Ber. Verh. Sächsisch. Akad. Wiss. Leipzig, Math.-Naturwiss. Kl. , 80 (1928) pp. 497–502 |
| [1c] | H. Grötzsch, "Ueber die Verzerrung bei schlichter konformer Abbildung mehrfach zusammenhängender Bereiche II" Ber. Verh. Sächsisch. Akad. Wiss. Leipzig, Math.-Naturwiss. Kl. , 81 (1929) pp. 217–221 |
| [1d] | H. Grötzsch, Ber. Verh. Sächsisch. Akad. Wiss. Leipzig, Math.-Naturwiss. Kl. , 82 (1930) pp. 69–80 |
| [1e] | H. Grötzsch, "Zum Parallelschlitztheorem der konformen Abbildung schlichter unendlich-vielfach zusammenhängender Bereiche" Ber. Verh. Sächsisch. Akad. Wiss. Leipzig, Math.-Naturwiss. Kl. , 83 (1931) pp. 185–200 |
| [1f] | H. Grötzsch, "Ueber möglichts konformen Abbildungen von schlichter Bereiche" Ber. Verh. Sächsisch. Akad. Wiss. Leipzig, Math.-Naturwiss. Kl. , 84 (1932) pp. 114–120 |
| [2] | G.M. Goluzin, "Geometric theory of functions of a complex variable" , Transl. Math. Monogr. , 26 , Amer. Math. Soc. (1969) (Translated from Russian) |
| [3] | J.A. Jenkins, "Univalent functions and conformal mappings" , Springer (1958) |
Comments
Cf. also Grötzsch theorems; Distortion theorems.
Grötzsch principle. Encyclopedia of Mathematics. URL: http://encyclopediaofmath.org/index.php?title=Gr%C3%B6tzsch_principle&oldid=22533